(Solved): Problem 3. Parametric Curve Design (4 points). For the data points \( \mathrm{P}_{0}, \mathrm{P}_ ...

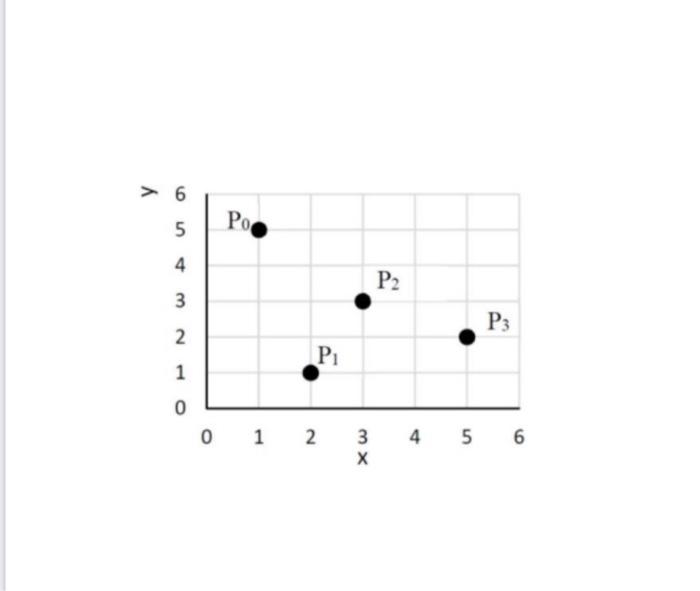
Problem 3. Parametric Curve Design (4 points). For the data points \( \mathrm{P}_{0}, \mathrm{P}_{1} \), and \( \mathrm{P}_{2} \), determine the equation for the parametric cubic spline with a tangent vector at point \( \mathrm{P}_{0} \) in \( (\mathrm{x}, \mathrm{y}) \) coordinates as \( \left(\cos \left(-30^{\circ}\right), \sin \left(-30^{\circ}\right)\right) \) that passes through these three points. Problem 4. Bezier Curve (4 points). For the given data points in the above plot, determine the equation for the Bezier curve if \( \mathrm{P}_{2} \) is moved to \( (4,2) \) and \( \mathrm{P}_{3} \) is moved to \( (7,4.5) \). Assume \( \mathrm{u}=0 \) at \( \mathrm{P}_{0} \) and \( \mathrm{u}=1 \) at \( \mathrm{P}_{3} \). Make a sketch of the Bezier curve that uses enough points on the curve to show the behavior near each data point. Clearly indicate how the curve behaves near each data point (above, below, on the point). You can use MATALB, Excel, or make a table and plot using a graph paper.