(Solved): PROBLEM 3 For the single-degree-of-freedom system shown below, \( m_{l}=1 \mathrm{~kg}, I_{O}=1 \m ...

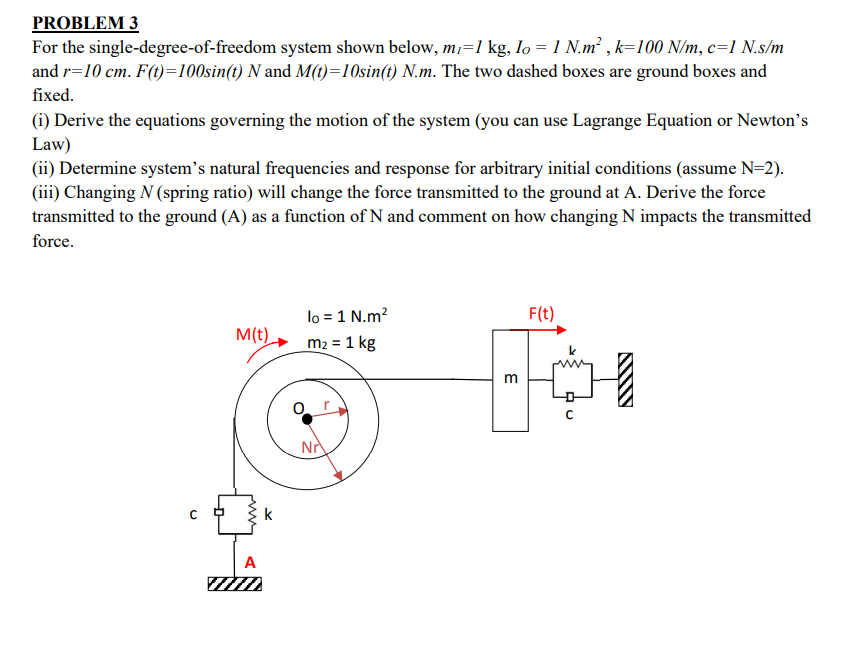
PROBLEM 3 For the single-degree-of-freedom system shown below, \( m_{l}=1 \mathrm{~kg}, I_{O}=1 \mathrm{N.m^{2 }}, k=100 \mathrm{~N} / \mathrm{m}, c=1 \mathrm{~N} . \mathrm{s} / \mathrm{m} \) and \( r=10 \mathrm{~cm} . F(t)=100 \sin (t) N \) and \( M(t)=10 \sin (t) N . \mathrm{m} \). The two dashed boxes are ground boxes and fixed. (i) Derive the equations governing the motion of the system (you can use Lagrange Equation or Newton's Law) (ii) Determine system's natural frequencies and response for arbitrary initial conditions (assume \( \mathrm{N}=2 \) ). (iii) Changing \( N \) (spring ratio) will change the force transmitted to the ground at \( \mathrm{A} \). Derive the force transmitted to the ground (A) as a function of \( \mathrm{N} \) and comment on how changing \( \mathrm{N} \) impacts the transmitted force.