(Solved): Problem 2: The cross-section of the beam is double-symmetric about its center and has the dimensio ...

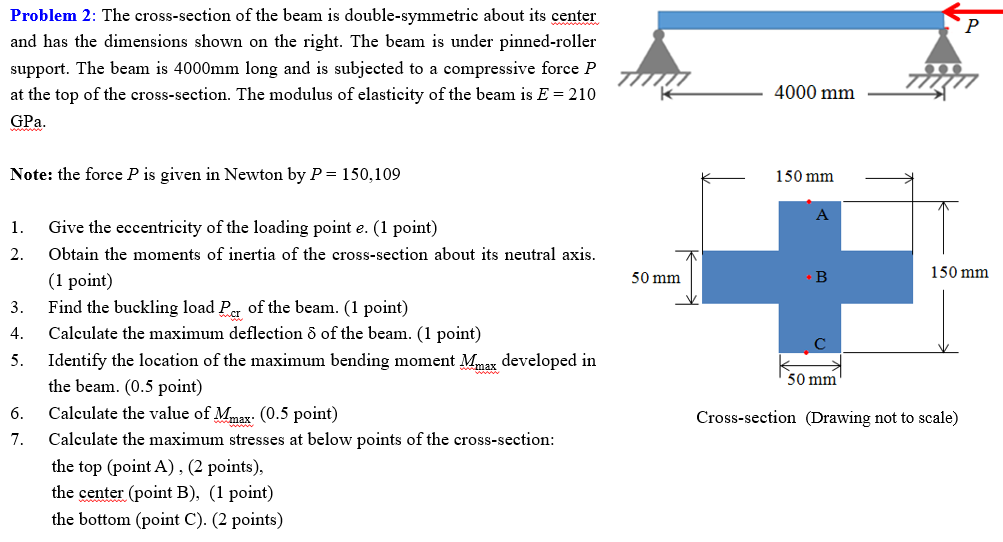
Problem 2: The cross-section of the beam is double-symmetric about its center and has the dimensions shown on the right. The beam is under pinned-roller support. The beam is \( 4000 \mathrm{~mm} \) long and is subjected to a compressive force \( P \) at the top of the cross-section. The modulus of elasticity of the beam is \( E=210 \) GPa. Note: the force \( P \) is given in Newton by \( P=150,109 \) 1. Give the eccentricity of the loading point \( e \). (1 point) 2. Obtain the moments of inertia of the cross-section about its neutral axis. (1 point) 3. Find the buckling load \( P_{c r} \) of the beam. (1 point) 4. Calculate the maximum deflection \( \delta \) of the beam. (1 point) 5. Identify the location of the maximum bending moment \( M_{\max } \) developed in the beam. ( \( 0.5 \) point) 6. Calculate the value of \( M_{\max } \) ( \( 0.5 \) point) \( \quad \) Cross-section (Drawing not to scale) 7. Calculate the maximum stresses at below points of the cross-section: the top (point A), (2 points), the center (point B), (1 point) the bottom (point \( C) \cdot(2 \) points)