(Solved): Problem 2. PN junction and applications (29 + Extra 5 pts) We have a silicon based PN junction dev ...

Problem 2. PN junction and applications (29 + Extra 5 pts) We have a silicon based PN junction device. The doping levels are: \( p \)-type; \( N_{A}=10^{18} \mathrm{~cm}^{-3} ; n \) type; \( N_{D}=10^{15} \mathrm{~cm}^{-3} \). Please refer to page 1 for relevant constants of silicon when necessary. 2a. (12 pts) Please calculate the \( V_{b i} \), junction width \( \mathrm{x}_{\mathrm{n}}, \mathrm{x}_{\mathrm{p}} \), and maximum electric field \( \varepsilon_{\max } \) (6 pts) Then draw the following at thermal equilibrium (6 pts): - the charge distribution (including \( x_{n}, x_{p} \) ), - electric field distribution (including \( \mathrm{x}_{\mathrm{n}}, \mathrm{x}_{\mathrm{p}} \) ), - energy band diagram \( \left(\mathbf{E}_{c}, \mathbf{E}_{\mathbf{v}}, \mathbf{E}_{\mathbf{i}}, \mathbf{E}_{\mathbf{f}}\right) \). You don't have to draw exactly to the scale, but need to show the difference in \( x_{n} \) and \( x_{p} \) and the \( \mathrm{E}_{\mathrm{f}} \) location relative to \( \mathrm{E}_{\mathrm{c}} \) and \( \mathrm{E}_{\mathrm{v}} \) in the p-type and n-type sides. 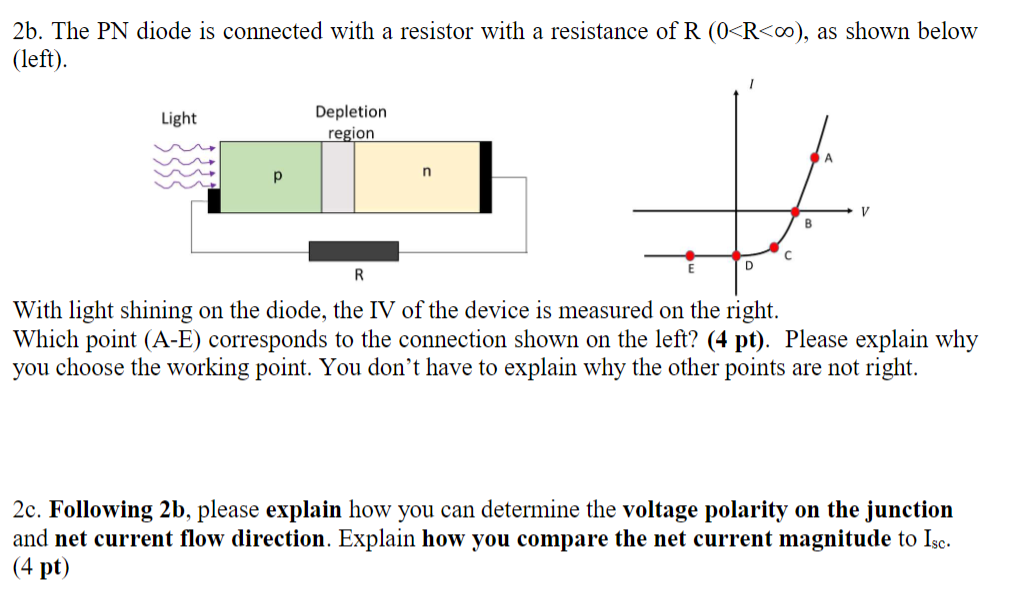2b. The PN diode is connected with a resistor with a resistance of \( \mathrm{R}(0<\mathrm{R}<\infty) \), as shown below (left). With light shining on the diode, the IV of the device is measured on the right. Which point (A-E) corresponds to the connection shown on the left? \( \mathbf{( 4} \mathbf{p t} \mathbf{)} \). Please explain why you choose the working point. You don't have to explain why the other points are not right. 2c. Following 2b, please explain how you can determine the voltage polarity on the junction and net current flow direction. Explain how you compare the net current magnitude to \( I_{s c} \). 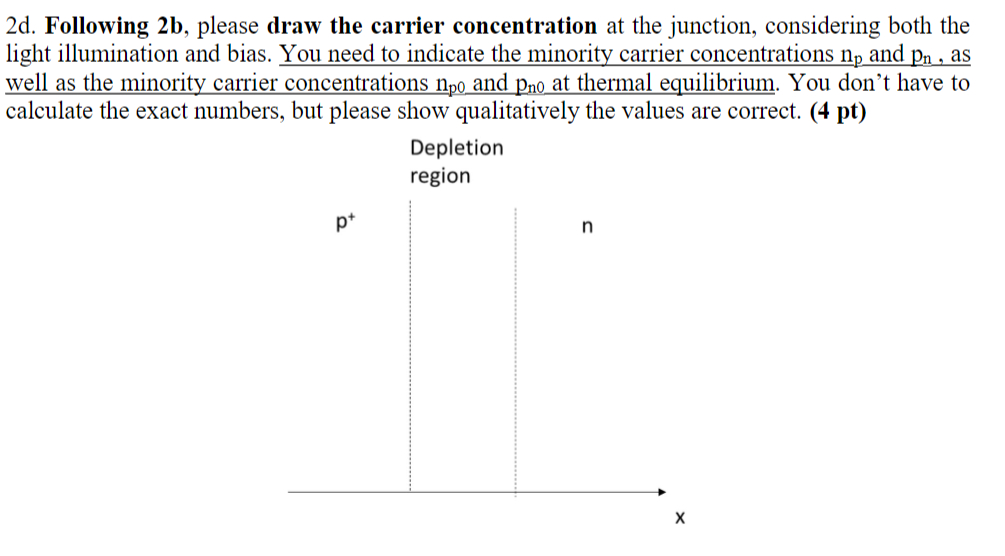(4 pt) \( 2 \mathrm{~d} \). Following \( \mathbf{2 b} \), please draw the carrier concentration at the junction, considering both the light illumination and bias. You need to indicate the minority carrier concentrations \( n_{p} \) and \( p_{n} \), as well as the minority carrier concentrations \( \mathrm{n}_{\mathrm{p} 0} \) and \( \mathrm{p}_{\mathrm{n} 0} \) at thermal equilibrium. You don't have to calculate the exact numbers, but please show qualitatively the values are correct. \( (\mathbf{4} \mathbf{p t}) \) 2e. Following \( \mathbf{2 b} \), please explain how you determine the voltage \( V \) created on the PN junction. \( (\mathbf{5} \) pts) Hint: You just need to show the expressions that can be directly solved, but don't need to calculate the exact numbers. To get the full credit, the only unknown in your expression should be \( V \). For example, you need to calculate the value of diode saturation current \( I_{S} \) in order to determine the total current of the solar cell. Additional parameters you may need: the photocurrent \( \mathrm{I}_{\mathrm{L}}=1 \mathrm{~mA} \), junction cross-sectional area \( \mathrm{A}=100 \mathrm{~cm}^{2} \), and a resistance \( \mathrm{R}=100 \mathrm{ohm} \). Carrier lifetime \( \tau_{p}=\tau_{n}=1 \mu \mathrm{s}, D_{n}=100 \mathrm{~cm}^{2}(\mathrm{~s})^{-1} \) and \( D_{p}=16 \) \( \mathrm{cm}^{2}(\mathrm{~s})^{-1} \). Assume ideal diode, i.e. the ideality factor \( \mathrm{n}=1 \). 2f. (This part is a general question on PN junction) The minority-carrier diffusion equation is a basic tool developed in this course for analyzing semiconductor devices such as PN diodes. Here, carriers are treated as classical particles with effective masses and they move according to classical trajectories. What is the main justification for this? (Extra \( 5 \mathrm{pts} \) ) Hint: Consider the semiconductor band structure and explain how effective mass can be used to represent the charged particle movement in a semiconductor crystal lattice.