(Solved): Problem 2 For the following reactions (a) and (b) i) Set up a stoichiometric table for each of the ...

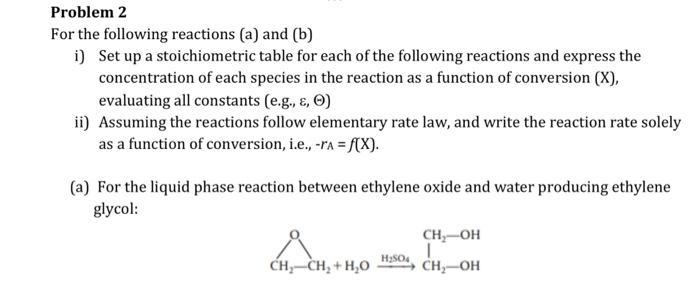
Problem 2 For the following reactions (a) and (b) i) Set up a stoichiometric table for each of the following reactions and express the concentration of each species in the reaction as a function of conversion \( (X) \), evaluating all constants (e.g., \( \varepsilon, \Theta \) ) ii) Assuming the reactions follow elementary rate law, and write the reaction rate solely as a function of conversion, i.e., \( -r_{\mathrm{A}}=f(\mathrm{X}) \). 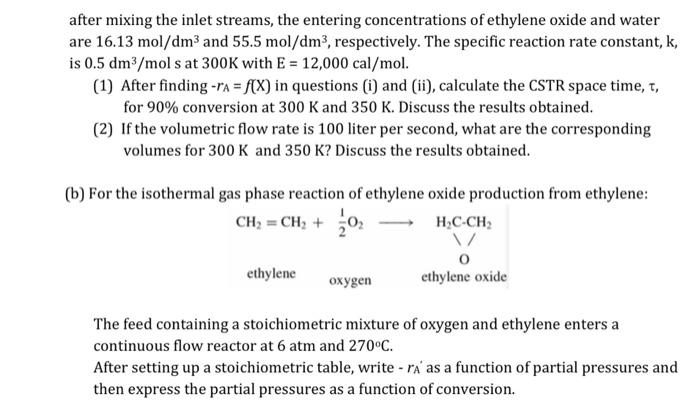(a) For the liquid phase reaction between ethylene oxide and water producing ethylene glycol: after mixing the inlet streams, the entering concentrations of ethylene oxide and water are \( 16.13 \mathrm{~mol} / \mathrm{dm}^{3} \) and \( 55.5 \mathrm{~mol} / \mathrm{dm}^{3} \), respectively. The specific reaction rate constant, \( \mathrm{k} \), is \( 0.5 \mathrm{dm}^{3} / \mathrm{mol} \mathrm{s} \) at \( 300 \mathrm{~K} \) with \( \mathrm{E}=12,000 \mathrm{cal} / \mathrm{mol} \). (1) After finding \( -r_{\mathrm{A}}=f(\mathrm{X}) \) in questions (i) and (ii), calculate the CSTR space time, \( \tau \), for \( 90 \% \) conversion at \( 300 \mathrm{~K} \) and \( 350 \mathrm{~K} \). Discuss the results obtained. (2) If the volumetric flow rate is 100 liter per second, what are the corresponding volumes for \( 300 \mathrm{~K} \) and \( 350 \mathrm{~K} \) ? Discuss the results obtained. (b) For the isothermal gas phase reaction of ethylene oxide production from ethylene: The feed containing a stoichiometric mixture of oxygen and ethylene enters a continuous flow reactor at \( 6 \mathrm{~atm} \) and \( 270^{\circ} \mathrm{C} \). After setting up a stoichiometric table, write - \( r_{A}{ }^{\prime} \) as a function of partial pressures and then express the partial pressures as a function of conversion.