(Solved): Problem 2: 25 points A simply supported beam ABCD is subjected to a linearly distributed load, wit ...
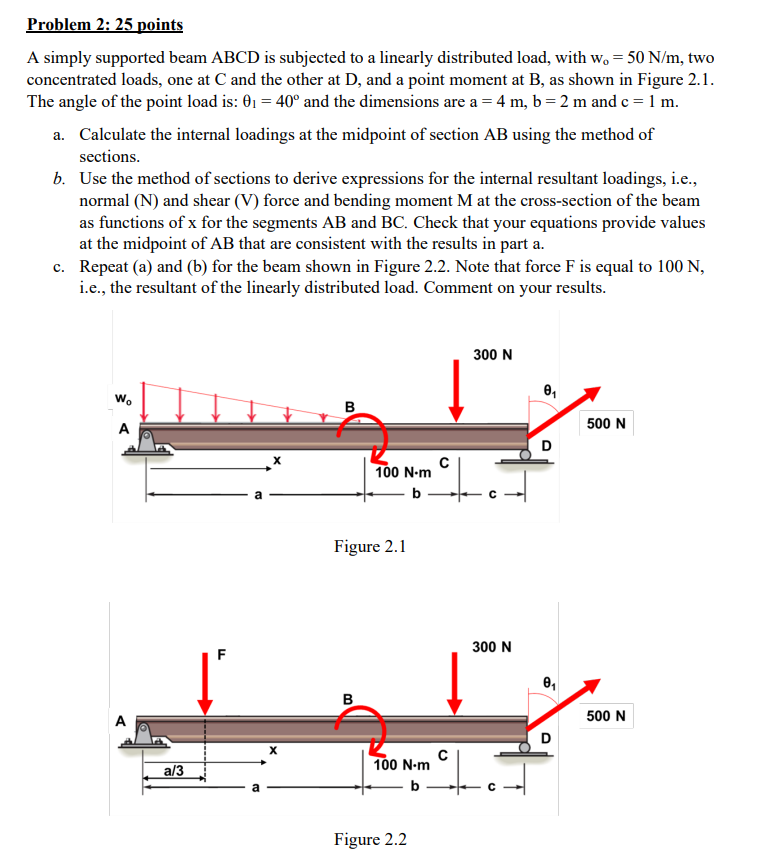
Problem 2: 25 points A simply supported beam ABCD is subjected to a linearly distributed load, with wo = 50 N/m, two concentrated loads, one at C and the other at D, and a point moment at B, as shown in Figure 2.1. The angle of the point load is: ?1 = 40o and the dimensions are a = 4 m, b = 2 m and c = 1 m.
a. Calculate the internal loadings at the midpoint of section AB using the method of sections.
b. Use the method of sections to derive expressions for the internal resultant loadings, i.e., normal (N) and shear (V) force and bending moment M at the cross-section of the beam as functions of x for the segments AB and BC. Check that your equations provide values at the midpoint of AB that are consistent with the results in part a.
c. Repeat (a) and (b) for the beam shown in Figure 2.2. Note that force F is equal to 100 N, i.e., the resultant of the linearly distributed load. Comment on your results.
Expert Answer
Pleaseobserve the solution carefully, comme