(Solved): Problem 1: Use u-substitution to convert each of the integrals in \( x \) to an integral in \( u \) ...

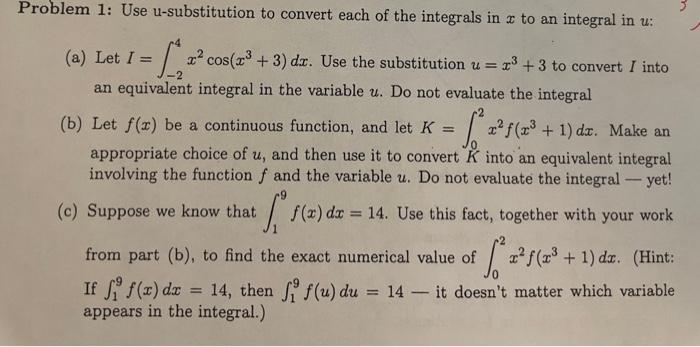
Problem 1: Use u-substitution to convert each of the integrals in \( x \) to an integral in \( u \) : (a) Let \( I=\int_{-2}^{4} x^{2} \cos \left(x^{3}+3\right) d x \). Use the substitution \( u=x^{3}+3 \) to convert \( I \) into an equivalent integral in the variable \( u \). Do not evaluate the integral (b) Let \( f(x) \) be a continuous function, and let \( K=\int_{0}^{2} x^{2} f\left(x^{3}+1\right) d x \). Make an appropriate choice of \( u \), and then use it to convert \( K \) into an equivalent integral involving the function \( f \) and the variable \( u \). Do not evaluate the integral - yet! (c) Suppose we know that \( \int_{1}^{9} f(x) d x=14 \). Use this fact, together with your work from part (b), to find the exact numerical value of \( \int_{0}^{2} x^{2} f\left(x^{3}+1\right) d x \). (Hint: If \( \int_{1}^{9} f(x) d x=14 \), then \( \int_{1}^{9} f(u) d u=14 \) - it doesn't matter which variable appears in the integral.)