(Solved): Problem 1 The figure below shows a field-controlled DC motor. Unlike the armature-controlled DC mot ...

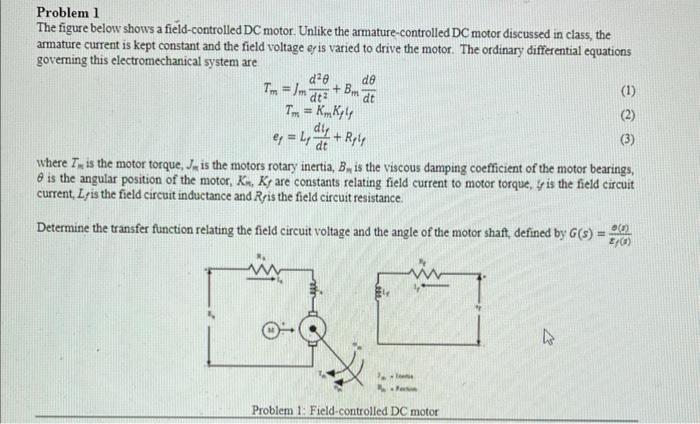
Problem 1 The figure below shows a field-controlled DC motor. Unlike the armature-controlled DC motor discussed in class, the armature current is kept constant and the field voltage ef is varied to drive the motor. The ordinary differential equations governing this electromechanical system are \[ \begin{array}{c} T_{m}=J_{m} \frac{d^{2} \theta}{d t^{2}}+B_{m} \frac{d \theta}{d t} \\ T_{m}=K_{m} K_{f} l_{f} \\ e_{f}=L_{f} \frac{d l_{f}}{d t}+R_{f} i_{f} \end{array} \] where \( T_{m} \) is the motor torque, \( J_{m} \) is the motors rotary inertia, \( B_{m} \) is the viscous damping coefficient of the motor bearings, \( \theta \) is the angular position of the motor, \( K_{n}, K_{f} \) are constants relating field current to motor torque, 4 is the field circuit, current, \( L \), is the field circuit inductance and \( R \), is the field circuit resistance. Determine the transfer function relating the field circuit voltage and the angle of the motor shaft, defined by \( G(s)=\frac{o(d)}{\varepsilon_{f}(d)} \) Problem 1: Field-controlled DC motor