(Solved): postulate from the axioms of neutral geometry. This flawed proof was given by Adrien-Marie Legendr ...

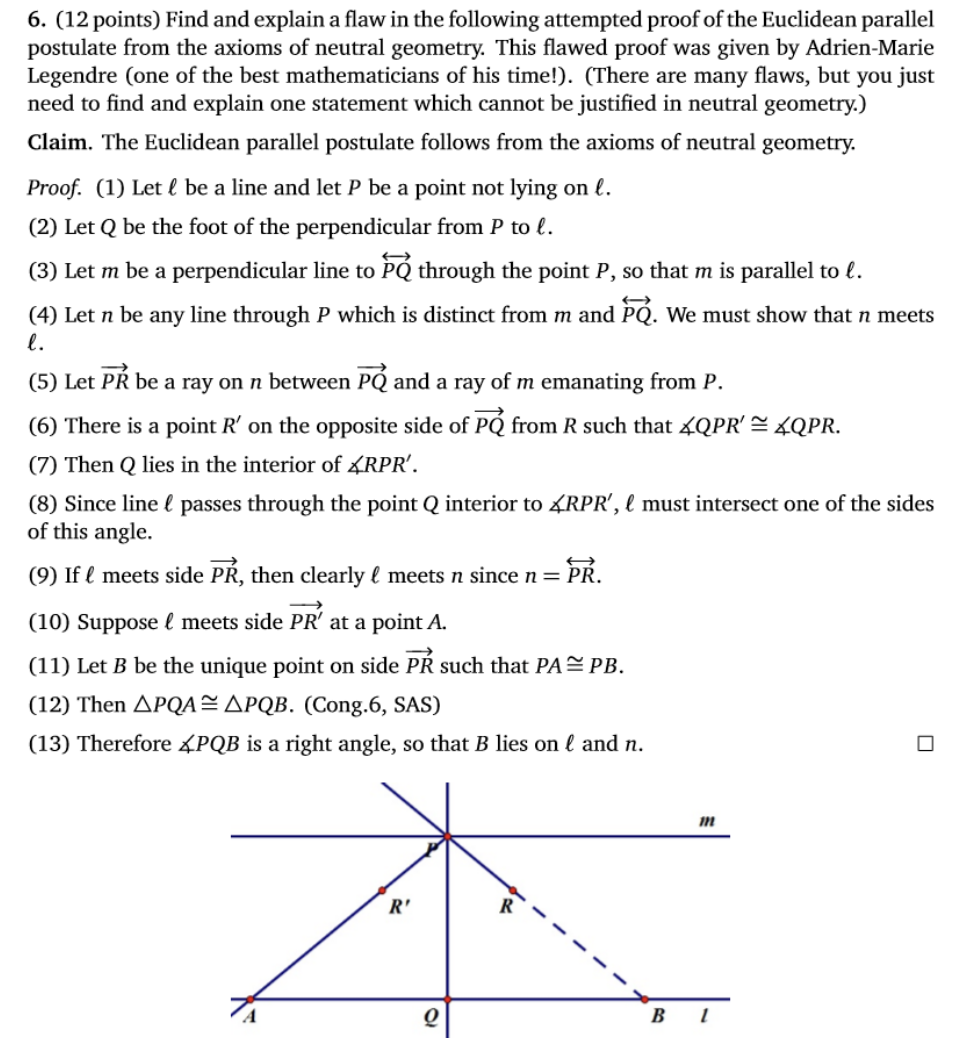
postulate from the axioms of neutral geometry. This flawed proof was given by Adrien-Marie Legendre (one of the best mathematicians of his time!). (There are many flaws, but you just need to find and explain one statement which cannot be justified in neutral geometry.) Claim. The Euclidean parallel postulate follows from the axioms of neutral geometry. Proof. (1) Let \( \ell \) be a line and let \( P \) be a point not lying on \( \ell \). (2) Let \( Q \) be the foot of the perpendicular from \( P \) to \( \ell \). (3) Let \( m \) be a perpendicular line to \( \overleftrightarrow{P Q} \) through the point \( P \), so that \( m \) is parallel to \( \ell \). (4) Let \( n \) be any line through \( P \) which is distinct from \( m \) and \( \overleftrightarrow{P Q} \). We must show that \( n \) meets \( \ell \) (5) Let \( \overrightarrow{P R} \) be a ray on \( n \) between \( \overrightarrow{P Q} \) and a ray of \( m \) emanating from \( P \). (6) There is a point \( R^{\prime} \) on the opposite side of \( \overrightarrow{P Q} \) from \( R \) such that \( \Varangle Q P R^{\prime} \cong \Varangle Q P R \). (7) Then \( Q \) lies in the interior of \( \angle R P R^{\prime} \). (8) Since line \( \ell \) passes through the point \( Q \) interior to \( \angle R P R^{\prime}, \ell \) must intersect one of the sides of this angle. (9) If \( \ell \) meets side \( \overrightarrow{P R} \), then clearly \( \ell \) meets \( n \) since \( n=\overleftrightarrow{P R} \) (10) Suppose \( \ell \) meets side \( \overrightarrow{P R^{\prime}} \) at a point \( A \). (11) Let \( B \) be the unique point on side \( \overrightarrow{P R} \) such that \( P A \cong P B \). (12) Then \( \triangle P Q A \cong \triangle P Q B \). (Cong.6, SAS) (13) Therefore \( \triangle P Q B \) is a right angle, so that \( B \) lies on \( \ell \) and \( n \).