(Solved): please use the following data: please answer the following: Understanding Standard Reduction Po ...

Understanding Standard Reduction Potentials A spontaneous reaction generates energy has a positive potential. Half-reaction potentials for reactions are typically given for reduction reactions only. Standard reduction potentials as measured against the standard hydrogen electrode, which is assigned a value of \( 0 \mathrm{~V} \). Any half-reactions with positive reduction potential is spontaneous as written. Half-reactions with negative reduction potential are non-spontaneous and more likely to occur in the reverse direction, as oxidation reactions. The standard reduction potential for copper and zinc are given below: \[ \begin{array}{l} \mathrm{Cu}^{2+}(\mathrm{aq})+2 \mathrm{e}^{-} \longrightarrow \mathrm{Cu}(\mathrm{s}), E_{\mathrm{red}}^{\circ}=+0.337 \mathrm{~V} \\ \mathrm{Zn}^{2+}(\mathrm{aq})+2 \mathrm{e}^{-} \longrightarrow \mathrm{Zn}(\mathrm{s}), E_{\mathrm{red}}^{\circ}=-0.763 \mathrm{~V} \end{array} \] Based on the standard reduction potentials, which ismore likely to be reduced? copper A voltaic cell creates a potential of usable electrical energy, in other words, the net process has a positive cell potential and is spontaneous. Consider a voltaic cell that consists of a copper electrode in a solution of \( \mathrm{Cu}^{2+} \) and a zinc electrode in a solution of \( \mathrm{Zn}^{2 t} \). connected by a salt bridge. Based on the standard reduction potentials, what is the anode and what is the cathode? Remember Zn(s) is the anode; that oxidation occurs at the anode and reduction occurs at the cathode. Cu(s) is the cathode The standard potential of a cell is calculated as the potential difference between the reduction potentials for the two half: reactions: \[ E_{\mathrm{cul}}^{\mathrm{v}}=E_{\mathrm{rad}}^{\mathrm{u}} \text { (cathode) }-E_{\text {ivi }}^{\mathrm{v}} \text { (anode) } \] What is the standard cell potential for the cell described above? 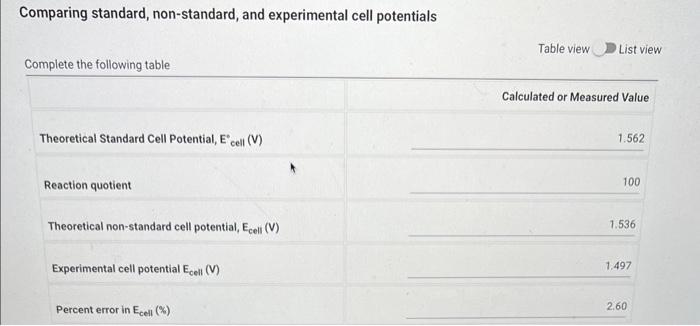\( 1.100 \) A standard potential, denoted by a degree sign, is one measured at a temperature of \( 298 \mathrm{~K} \) with all involved solutes present in one molar \( (1 \mathrm{M}) \) concentrations. Potentials under non-standard conditions of temperature and concentration can be calculated using the Nernst equation \[ E_{c o l l}=E_{\text {cil }}^{\circ}-\frac{R T}{n P} \ln Q \] Where \( \mathrm{R} \) is the ideal gas, \( 8.3145 \mathrm{~J} /\{\mathrm{K} \).mol) and \( \mathrm{F} \) is Faraday's constant, \( 96,485 \mathrm{C} / \mathrm{mol}, \mathrm{T} \) is temperature in Kelvin, \( n \) is the number of moles of electrons transferred in the balanced overall cell reactionm and \( Q \) is the reaction quotient. Consider the following voltaic cell: \[ \mathrm{Zn}(\mathrm{s})\left|\mathrm{Zn}^{2+}(1.0 \mathrm{M}) \| \mathrm{Ag}^{+}(0.1 \mathrm{M})\right| \mathrm{Ag}(\mathrm{s}) \] The standard reduction potential for zinc and silver are as follows: \[ \begin{array}{l} \mathrm{Zn}^{2+}(\mathrm{aq})+2 \mathrm{e}^{-} \longrightarrow \mathrm{Zn}(\mathrm{s}), E_{\text {red }}^{\circ}=-0.763 \mathrm{~V} \\ \mathrm{Ag}^{+}(\mathrm{aq})+\mathrm{e}^{-} \longrightarrow \mathrm{Ag}(\mathrm{s}), E_{\text {red }}^{\circ}=+0.799 \mathrm{~V} \end{array} \] Experimental cell potential: \( 0.5 \mathrm{pts}) \) What is the half-reaction for the anode? A. \( \mathrm{Zn}^{2+}(\mathrm{aq})+2 \mathrm{e}^{-} \longrightarrow \mathrm{Zn}(\mathrm{s}) \) B. \( \mathrm{Ag}^{+}(\mathrm{aq})+\mathrm{e}^{-} \longrightarrow \mathrm{Ag}(\mathrm{s}) \) C. \( \mathrm{Zn}(\mathrm{s}) \longrightarrow \mathrm{Zn}^{2+}(\mathrm{aq})+2 \mathrm{e} \) D. \( \mathrm{Ag}(\mathrm{s}) \longrightarrow \mathrm{Ag}^{+}(\mathrm{aq})+\mathrm{e}^{-} \) \( (0.5 \mathrm{pts}) \) What is the half-reaction for the cathode? A. \( \mathrm{Zn}^{2+}(\mathrm{aq})+2 \mathrm{e}^{-} \rightarrow \mathrm{Zn}(\mathrm{s}) \) B. \( \mathrm{Ag}^{+}(\mathrm{aq})+\mathrm{e}^{-} \rightarrow \mathrm{Ag}(\mathrm{s}) \) C. \( \mathrm{Zn}(\mathrm{s}) \rightarrow \mathrm{Zn}^{2+}(\mathrm{aq})+2 \mathrm{e} \) D. \( \mathrm{Ag}(\mathrm{s}) \rightarrow \mathrm{Ag}^{+}(\mathrm{aq})+\mathrm{e}^{-} \) (1pts) What is the complete redox reaction for the cell? A. \( \mathrm{Zn}(\mathrm{s})+2 \mathrm{Ag}^{+}(\mathrm{aq}) \rightarrow \mathrm{Zn}^{2+}(\mathrm{aq})+2 \mathrm{Ag}(\mathrm{s}) \) B. \( \mathrm{Zn}^{2+}(\mathrm{aq})+\mathrm{Ag}(\mathrm{s}) \longrightarrow \mathrm{Zn}(\mathrm{s})+\mathrm{Ag}^{+}(\mathrm{aq}) \) C. \( \mathrm{Zn}(\mathrm{s})+\mathrm{Ag}(\mathrm{s}) \rightarrow \mathrm{Zn}^{2+}(\mathrm{aq})+\mathrm{Ag}^{+}(\mathrm{aq}) \) D. \( \mathrm{Zn}(\mathrm{s})+\mathrm{Ag}^{+}(\mathrm{aq}) \longrightarrow \mathrm{Zn}^{2+}(\mathrm{aq})+\mathrm{Ag}(\mathrm{s}) \) Comparing standard, non-standard, and experimental cell potentials Table view \( \longrightarrow \) List view