Home /
Expert Answers /
Advanced Math /
please-solve-b-and-e-i-would-like-to-see-the-detailed-steps-to-please-5-104-for-each-case-below-pa229
(Solved): please solve b) and e) I would like to see the detailed steps to please 5.104 For each case below, ...
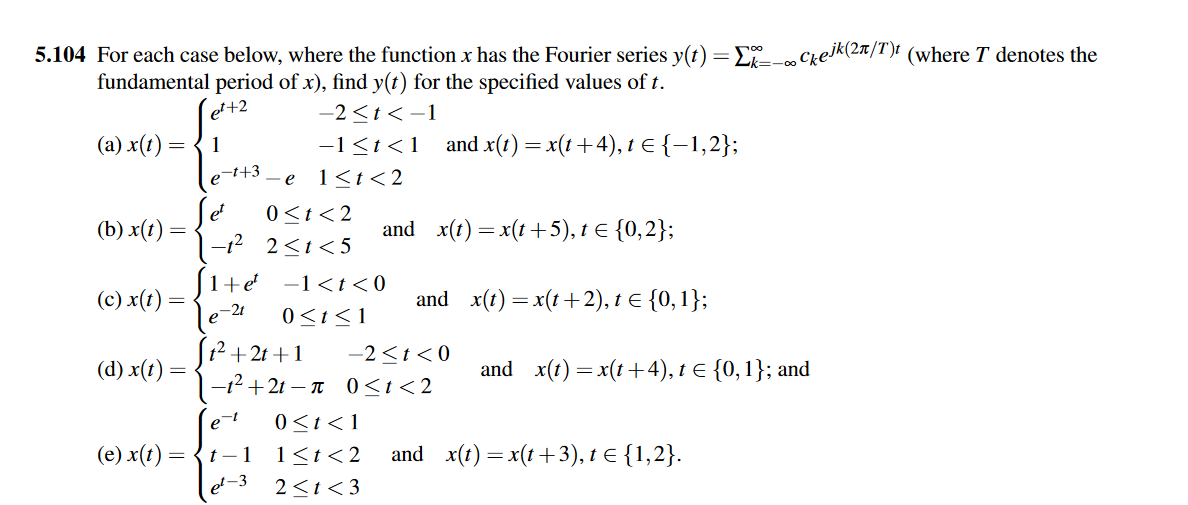
please solve b) and e) I would like to see the detailed steps to please 5.104 For each case below, where the function
xhas the Fourier series
y(t)=\sum_(k=-\infty )^(\infty ) c_(k)e^(jk(2(\pi )/(T))t)(where
Tdenotes the fundamental period of
x), find
y(t)for the specified values of
t. (a)
x(t)={(e^(t+2),-2<=t<-1),(1,-1<=t<1),(e^(-t+3)-e,1<=t<2):}and
x(t)=x(t+4),tin{-1,2}; (b)
x(t)={(e^(t),0<=t<2),(-t^(2),2<=t<5),():}and
,x(t)=x(t+5),tin{0,2}; (c)
,x(t)=x(t+2),tin{0,1}x(t)={(t^(2)+2t+1,-2<=t<0),(-t^(2)+2t-\pi ,0<=t<2),():},x(t)=x(t+4),tin{0,1};x(t)={(e^(-t),0<=t<1),(t-1,1<=t<2),(e^(t-3),2<=t<3),():},x(t)=x(t+3),tin{1,2}