(Solved): Please help. Problem 2. Consider the differential element shown below with normal \\( (X \\) and \\( ...

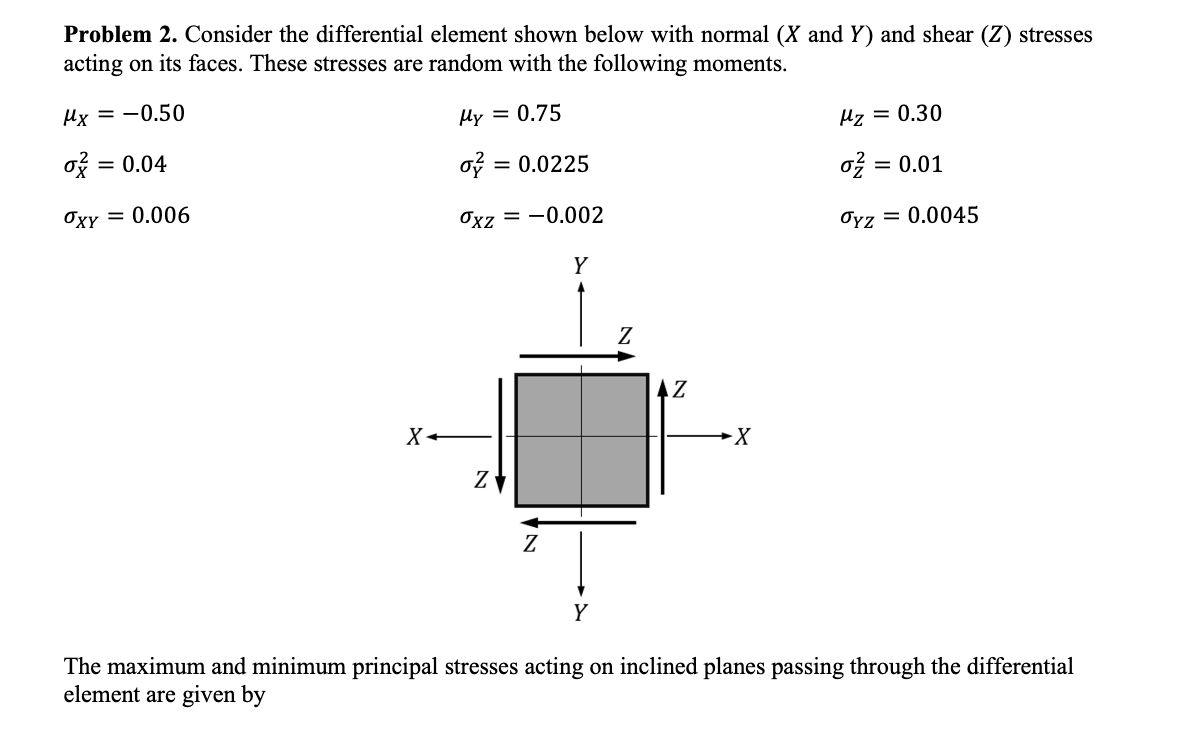
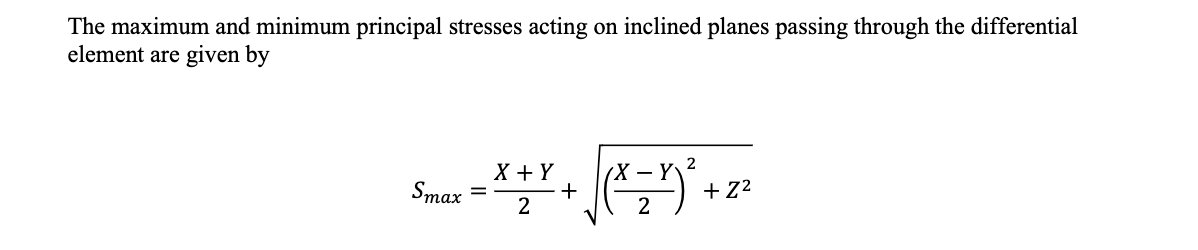
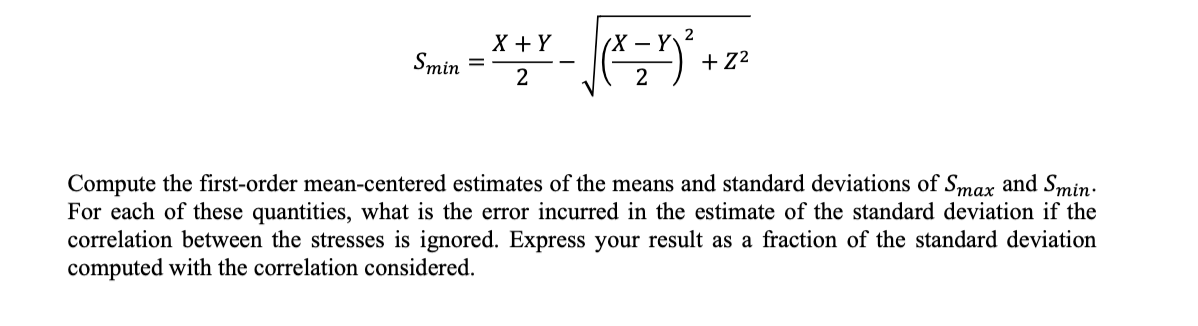
Problem 2. Consider the differential element shown below with normal \\( (X \\) and \\( Y) \\) and shear \\( (Z) \\) stresses acting on its faces. These stresses are random with the following moments. \\[ \\begin{array}{lll} \\mu_{X}=-0.50 & \\mu_{Y}=0.75 & \\mu_{Z}=0.30 \\\\ \\sigma_{X}^{2}=0.04 & \\sigma_{Y}^{2}=0.0225 & \\sigma_{Z}^{2}=0.01 \\\\ \\sigma_{X Y}=0.006 & \\sigma_{X Z}=-0.002 & \\sigma_{Y Z}=0.0045 \\end{array} \\] The maximum and minimum principal stresses acting on inclined planes passing through the differential element are given by\r\nThe maximum and minimum principal stresses acting on inclined planes passing through the differential element are given by \\[ S_{\\max }=\\frac{X+Y}{2}+\\sqrt{\\left(\\frac{X-Y}{2}\\right)^{2}+Z^{2}} \\]\r\n\\[ S_{\\min }=\\frac{X+Y}{2}-\\sqrt{\\left(\\frac{X-Y}{2}\\right)^{2}+Z^{2}} \\] Compute the first-order mean-centered estimates of the means and standard deviations of \\( S_{\\max } \\) and \\( S_{\\min } \\). For each of these quantities, what is the error incurred in the estimate of the standard deviation if the correlation between the stresses is ignored. Express your result as a fraction of the standard deviation computed with the correlation considered.