(Solved): Please Help A. For the three cases shown determine the factored moment, Mu, at the critical section ...

Please Help

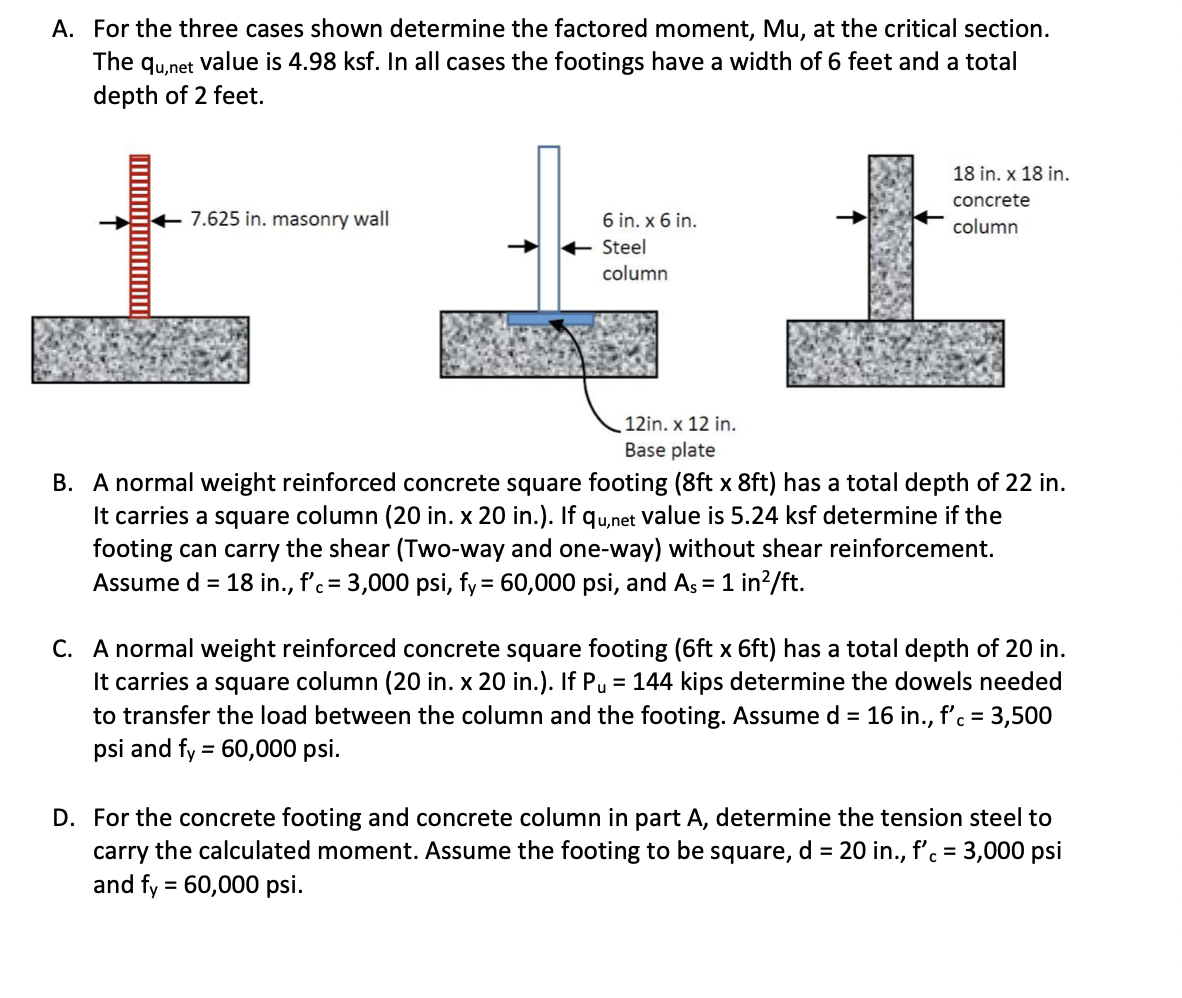
A. For the three cases shown determine the factored moment, Mu, at the critical section. The \( q_{u, \text { net }} \) value is \( 4.98 \mathrm{ksf} \). In all cases the footings have a width of 6 feet and a total depth of 2 feet. B. A normal weight reinforced concrete square footing \( (8 \mathrm{ft} x 8 \mathrm{ft}) \) has a total depth of 22 in. It carries a square column (20 in. \( x 20 \) in.). If \( q_{u, \text { net }} \) value is \( 5.24 \mathrm{ksf} \) determine if the footing can carry the shear (Two-way and one-way) without shear reinforcement. Assume \( d=18 \) in., \( f^{\prime}{ }_{c}=3,000 \mathrm{psi}, f_{y}=60,000 \mathrm{psi} \), and \( A_{s}=1 \mathrm{in}^{2} / \mathrm{ft} \). C. A normal weight reinforced concrete square footing \( (6 \mathrm{ft} x 6 \mathrm{ft}) \) has a total depth of 20 in. It carries a square column (20 in. \( x 20 \) in.). If \( P_{u}=144 \) kips determine the dowels needed to transfer the load between the column and the footing. Assume \( d=16 \) in., \( f^{\prime}{ }_{c}=3,500 \) psi and \( f_{y}=60,000 \) psi. D. For the concrete footing and concrete column in part A, determine the tension steel to carry the calculated moment. Assume the footing to be square, \( d=20 \) in., \( f^{\prime}{ }_{c}=3,000 \mathrm{psi} \) and \( \mathrm{f}_{\mathrm{y}}=60,000 \mathrm{psi} \).