(Solved): Part 1.Re-write the definite integral in terms of the variable u and remember to use the ...

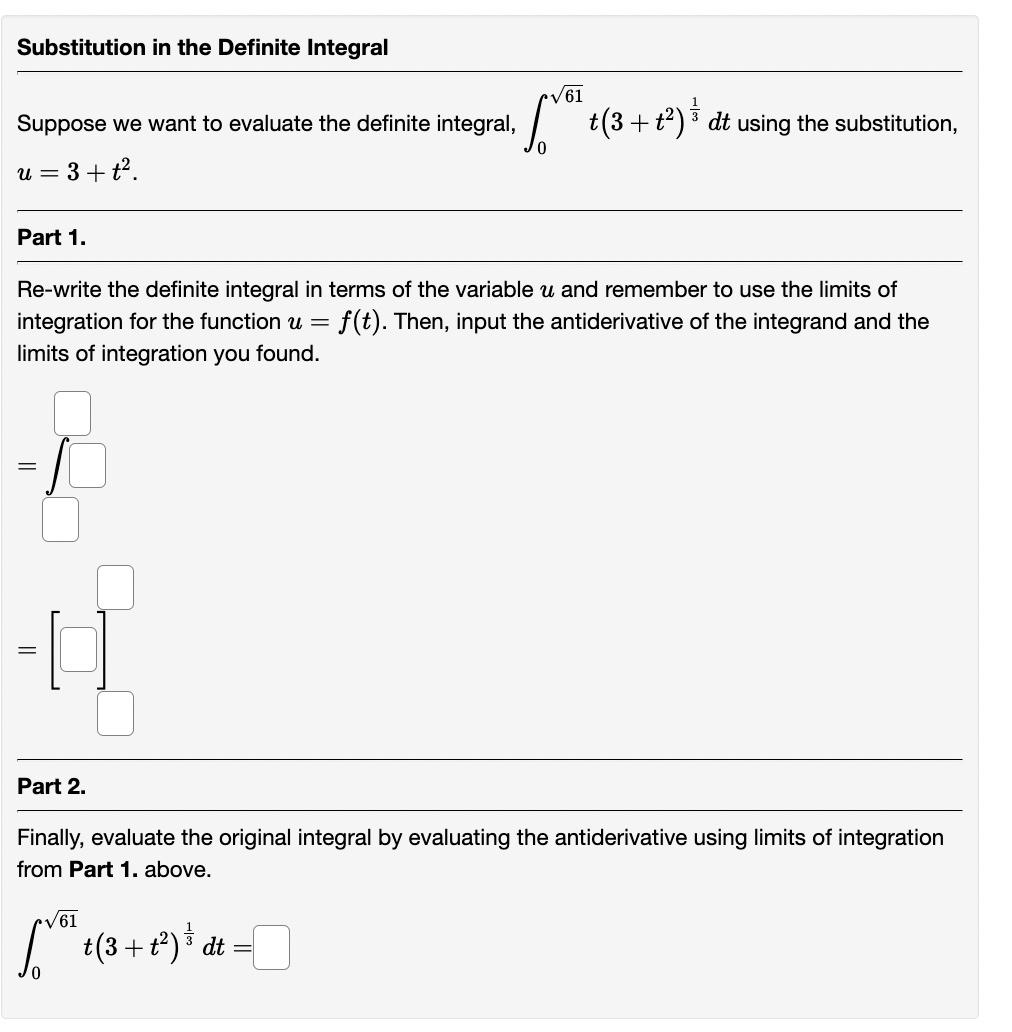
Part 1.Re-write the definite integral in terms of the variable u and remember to use the limits of integration for the function u=f(t). Then, input the antiderivative of the integrand and the limits of integration you found.

Part 2.Finally, evaluate the original integral by evaluating the antiderivative using limits of integration from Part 1. above.

please answer blank spots, thank you 

Suppose we want to evaluate the definite integral, \( \int_{0}^{\sqrt{61}} t\left(3+t^{2}\right)^{\frac{1}{3}} d t \) using the substitution, \( u=3+t^{2} \) Part 1. Re-write the definite integral in terms of the variable \( u \) and remember to use the limits of integration for the function \( u=f(t) \). Then, input the antiderivative of the integrand and the limits of integration you found. \[ =\int \] \[ =[\rceil \] Part 2. Finally, evaluate the original integral by evaluating the antiderivative using limits of integration from Part 1. above. \[ \int_{0}^{\sqrt{61}} t\left(3+t^{2}\right)^{\frac{1}{3}} d t= \]