(Solved): Objective To introduce the principles of indentation hardness testing. Introduction Hardness is def ...

Objective To introduce the principles of indentation hardness testing. Introduction Hardness is defined as the resistance of a material to plastic deformation such as indentation, wear, scratch, and abrasion. Hardness is directly related to the mechanical properties of the material. Factors influencing hardness include microstructure, grain size, strain hardening, etc. In hardness test, a small indenter is forced into the surface of a material to be tested. The depth or size of the resulting indentation is measured and related to a hardness number; the softer the material, the larger and deeper the indentation, and the lower the hardness index number. Hardness tests are performed more frequently than any other mechanical test for several reasons: a) They are simple and inexpective- typically, no special specimen need be prepared, and the testing apparatus is relatively inexpensive. b) The test is non-destructive- the specimen is neither fractured nor excessively deformed; a small indentation is the only deformation. c) Other mechanical properties often may be estimated from hardness data, such as tensile strength. The most popular methods are Rockwel, Brinell, and Vickers hardness tests for metals and alloys. 1. Brinell Hardness Test In Brinell tests, a hard, spherical indenter is forced into the surface of the material to be tested. The diameter of the hardened steel (or tungsten carbide) indenter is \( 10 \mathrm{~mm} \). Standard loads range between 500 and \( 3000 \mathrm{~kg} \) in \( 500 \mathrm{~kg} \) increments are used. The load is applied for 10 to 30 seconds. Harder materials require greater applied loads. The Brinell hardness number is calculated by dividing the load applied by the surface area of the indentation (Figure 1). Figure 1. The sciematic diagram of the Brineli hardness test \[ H B=\frac{\text { Applicd load }(\mathrm{kg})}{\text { Surface area of impression }\left(\mathrm{mm}^{2}\right)}=\frac{P}{\frac{\pi \cdot D \cdot\left(D-\sqrt{D^{2}-d^{2}}\right)}{2}} \] \[ H B=\frac{2 \cdot P}{\pi \cdot D \cdot\left(D-\sqrt{\left.D^{2}-d^{2}\right)}\right.} \] where \( \mathrm{P}= \) lond in kilograms \( \mathrm{D}= \) ball diameter in mm \( \mathrm{d}= \) diameter of impression in \( \mathrm{mm} \). The diameter of the impression is the avernge of two readings at right angles. The Brinell hardness number reveals the test condition, and looks like this, \( { }^{-75} \mathrm{HB} 10 / 50030^{\circ} \) which means that a Brinell Hardness of 75 was obtained using a \( 10 \mathrm{~mm} \) diameter hardened steel ball with a \( 500 \mathrm{~kg} \) load applied for a period of 30 seconds. In order to obtain accurate Brinell values the relationship betwecn d and D must be \( \mathrm{d}=0.25 \mathrm{D} \) to \( 0.50 \mathrm{D} \). Hence, the ball diameter and load applied is specified for the material under test e.g. for steels, \( \frac{P}{D^{2}}-30 \) for coper alloys, \( \frac{P}{D^{2}} 10 \). For example, the load used in a Brinell Test carried out on a stecl component using a \( 10 \mathrm{~mm} \) diameter stecl ball: \[ \frac{P}{D^{2}}-30 \] when \( \mathrm{D}=10, \mathrm{P}=30 \mathrm{x} 10^{2}=3000 \mathrm{~kg} \). Hence a \( 3000 \mathrm{~kg} \) load is required. The Brinell Test has the following limitations: a) The impression is large \( (2-4 \mathrm{~mm} \) in diameter) and this may act as a stress raiser in a component. It may also be unacceptable on grounds of appearance c.g-a car body panel, although acceptable on a car cylinder block. b) The large depth of the impression precludes its use on plated or surfice hardened components. c) Very hard materials will deform the indenter, hence the Brinell test is limited to materials of up to \( 450 \mathrm{HB} \) for a steel ball, and \( 600 \mathrm{HB} \) for tungsten carbide ball. 2. Rockwell Hardness Test The Rockwell test is the most common method used to measure hardness because they are so simple to perform and require no special skills. 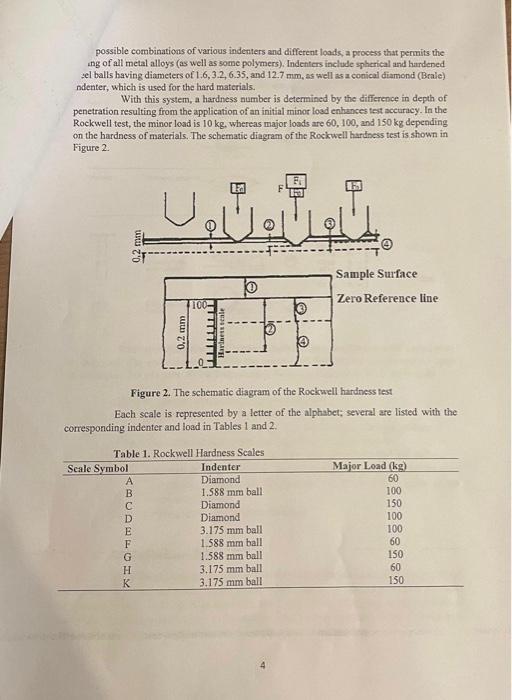Several different scales may be used 3 possible combinations of various indenters and different loads, a process that permits the ing of all metal alloys (as well as some polymers). Indenters include spherical and handened sel balls having diameters of 1.6,3.2,6.35, and \( 12.7 \mathrm{~mm} \), as well as a conical diamond (Brale) ndenter, which is used for the hard materials. With this system, a hardness number is determined by the difference in depth of penetration resulting from the application of an initial minor load enhances test accuracy. In the Rockwell test, the minor load is \( 10 \mathrm{~kg} \), whereas major loads are 60,100 , and \( 150 \mathrm{~kg} \) depending on the hardness of materials. The schematie diagram of the Rockwell hardness test is shown in Figure 2. Figure 2. The schematic diagram of the Rockwell hardness test Each scale is represented by a letter of the alphabet; several are listed with the corresponding indenter and toad in Tables 1 and 2 . 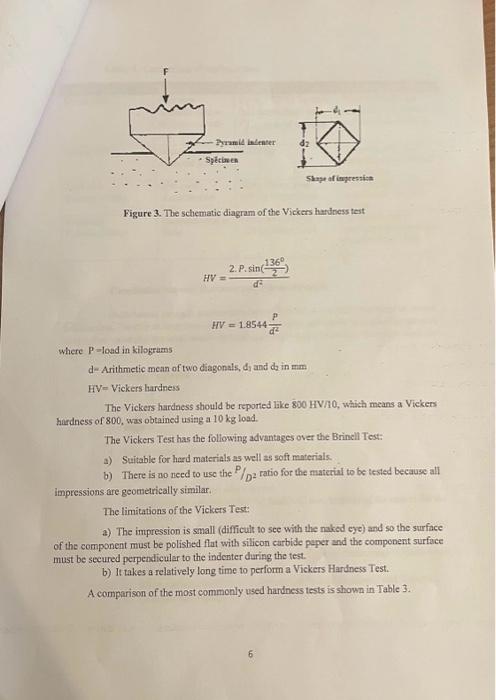Table 2. Hardness Tests When specifying Rockwell hardness, both hardness number and scale symbol must be indicated. The scale is designated by the symbol He followed by the appropriate scale identification. For example, 80 HRB represents a Rockwell hardness of 80 on the B scale. There are several considerations for Rociswell test: - Require clean and well positioned indenter and anvil - The test sample should be clean, dry, smocth and oxide-free surface - The surface should be flat and perpendicular to the indenter - Low reading of hardness value might be expected in cylindrical surfaces - Specimen thickness should be 10 times higher than the depth of the indenter - The spacing berween the indentations should be 3 to 5 times of the indentation diameter - Losding specd should be standardized 3. Vickers Hardness Test The Vickers hardness test is based on the same principle as the Brinell test, except the indenter is a diamond pyramid with square based (Figure 3). The angle between the faces of pyrumid is \( 136^{\circ} \). Test load is selected between 1 and \( 120 \mathrm{~kg} \) depending on the hardness of materials. The load applied for 10 to 15 seconds. The Vickers Hardness (HV) of materials is obtained by dividing the applied losd by the surfice area of iodentation. Figure 3. The schematic diagram of the Vickers harlocss test. \[ \begin{array}{l} H V=\frac{2 . P \cdot \sin \left(\frac{136^{\circ}}{2}\right)}{d^{2}} \\ H V=1.8544 \frac{P}{d^{2}} \end{array} \] where \( \mathrm{P}= \) load in kilograms \( \mathrm{d} \) - Arithmetic mean of two diagonals, \( \mathrm{d}_{1} \) and \( \mathrm{d}_{2} \) in \( m \) \( \mathrm{HV}= \) Vickers hardness The Vickers hardness should be reported like 800 HV/10, which means a Vickers hardness of 800 , was obtained using a \( 10 \mathrm{~kg} \) load. The Vickers Test has the following advantages over the Brinell Test: a) Suitable for hard materials as well as soft materials. b) There is no need to use the \( P / D^{2} \) ratio for the material to be tested because all impressions are geometrically similar. The limitations of the Vickers Test: a) The impression is small (difficult to see with the naked cye) and so the surface of the component must be polished flat with silicon curbide puper and the component surface must be secured perpendicular to the indenter during the test. b) It takes a relatively long time to perform a Vickers Hardness Test. A comparison of the most commonly used hardness tests is shown in Table 3 . Table 3. Comparison of hardness iests. Correlation between Hardeess and Tensile Strength Both tensile strength and haddeess are indicators of a metal's resistance to plastic deformation. There is an enpirical relation between hardness and tensile strength of metals and alloys. For example, for steel tersile strength can be rooghly estimated from the handess measarement as follows: TS (MPa) \( 3.45 \times 14 B \) To Report: 1. List hardness values of Rockwell test regults. 2. Discussion a) Define the hardness of a material. b) Describe the procedure for the Rockwell test, explaining the resson for the pre-load. c) What is the limitation on the thickness of specimens for a hardness tex?? Explain. d) What are the limitations for the distance from specimen edge to indentation and distance between indentations? e) What surface condition is necessary for Brincll, Rockwell and Vickers? f) Compute the average and standard deviation values.