(Solved): Now, in order to compute the semi-major axis (a) of Europa's orbit, we need the distance of Jupiter ...

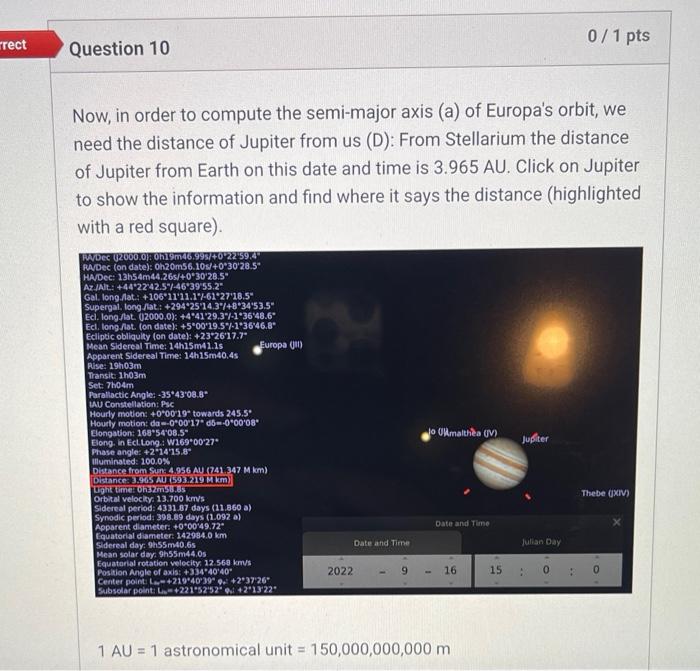
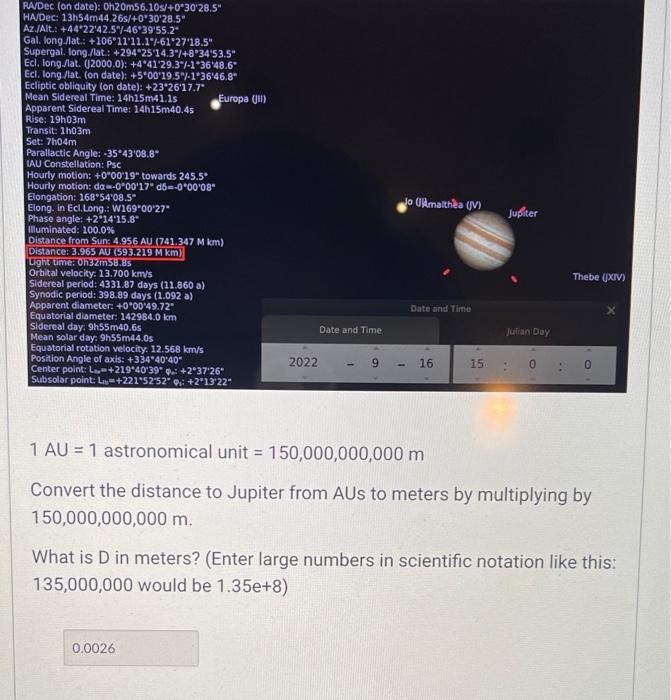
Now, in order to compute the semi-major axis (a) of Europa's orbit, we need the distance of Jupiter from us (D): From Stellarium the distance of Jupiter from Earth on this date and time is \( 3.965 \mathrm{AU} \). Click on Jupiter to show the information and find where it says the distance (highlighted with a red square). \( 1 \mathrm{AU}=1 \) astronomical unit \( =150,000,000,000 \mathrm{~m} \) \( 1 \mathrm{AU}=1 \) astronomical unit \( =150,000,000,000 \mathrm{~m} \) Convert the distance to Jupiter from AUs to meters by multiplying by \( 150,000,000,000 \mathrm{~m} \). What is D in meters? (Enter large numbers in scientific notation like this: \( 135,000,000 \) would be \( 1.35 \mathrm{e}+8) \) Next use the small angle equation to compute the semi-major axis (a) of Europa's orbit. Use the distance of Jupiter from Earth (D) from the previous question in meters and the angular distance between Jupiter and Europa in radians \( (\alpha) \) from Question 9. Remember to use your original answer with all the significant figures. \[ a=D \times \alpha \] (Enter large numbers in scientific notation like this: \( 135,000,000 \) would be \( 1.35 \mathrm{e}+8) \) This is the semi-major axis of Europa in meters: Unlike Callisto, Europe's orbit passes directly in line with Jupiter. Therefore to make things easier, to measure the revolution period of Europa, we suggest writing down the time in which Europa touches the limb of Jupiter (it is tangent to Jupiter - fig. 6), then we accelerate the time, follow the orbit of Europa around Jupiter and write down the time in which we see a second time the same configuration (Europa tangent to the limb of Jupiter after a revolution). Make sure it does a complete orbit. In this configuration, Europa has just finished moving in front of Jupiter. The second time recorded is when it is done moving in front of Jupiter again. If you stop Europa when it is headed behind Jupiter you are only seeing half way through its orbit. To get the placement and time accurate, you can pause the time and then use the Date/time window to increase the time by hour and minutes until Europa is close to the edge of Jupiter. You can also center on Europa to keep your screen centered on Europa has time advances. Use the search button to find Europa and then make sure the "center the selected object" (\$I) button is on. The difference between the two times corresponds to the revolution period of Europa. Fig. 6: Example of start point for the computation of the revolution period of Europa around Jupiter. For the start point, the time is \( 13: 39: 00 \) on \( 9 / 17 / 2022 \). For the end point, we will tell you the date 9/21/2022, but not the time. You must find the time when Europa is at the same position as it was on \( 9 / 17 / 2022 \) at 13:39:00. Use this website to calculate the difference in time: https://www.timeanddate.com/date/timeduration.html? \( \mathrm{d} 1=\& \mathrm{~m} 1=07 \& \mathrm{y} 1=\& \mathrm{~d} 2=\& \mathrm{~m} 2=\& \mathrm{y} 2=\& \mathrm{~h} 1=\& \mathrm{1} 1=\& \mathrm{~s} 1=\& \mathrm{~h} 2=\& \mathrm{i} 2=\& \mathrm{~s} 2=\& \mathrm{c} \) Start date and time in this example: \( 13: 39: 00 \mathrm{pm} \) on \( 9 / 17 / 2022 \). End date and time in this example: \( x x: x x: x x \) xm on 9/21/2022. (BE CAREFUL: Stellarium displays time in \( 24 \mathrm{hr} \) clock format. 10:30:52 means 10:30:52am and 22:49:00 means 10:49:00pm) Enter the time difference in seconds. 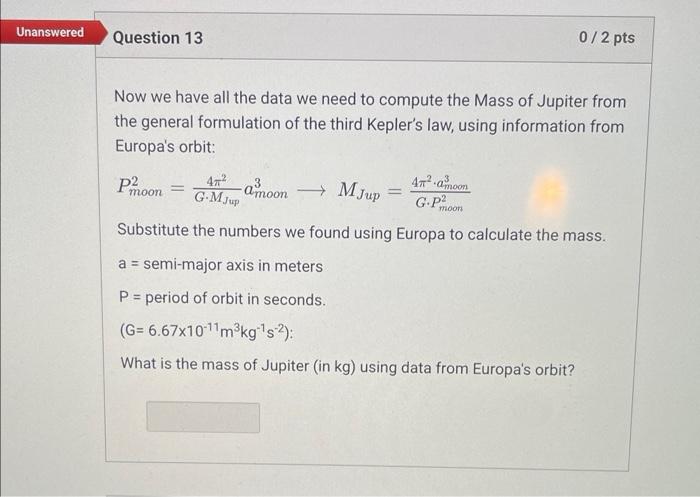This is the period (P) of Europa: Now we have all the data we need to compute the Mass of Jupiter from the general formulation of the third Kepler's law, using information from Europa's orbit: \[ P_{\text {moon }}^{2}=\frac{4 \pi^{2}}{G \cdot M_{\text {Jup }}} a_{\text {moon }}^{3} \longrightarrow M_{J u p}=\frac{4 \pi^{2} \cdot a_{\text {moon }}^{3}}{G \cdot P_{\text {moon }}^{2}} \] Substitute the numbers we found using Europa to calculate the mass. \( a= \) semi-major axis in meters \( P= \) period of orbit in seconds. \[ \left(G=6.67 \times 10^{-11} \mathrm{~m}^{3} \mathrm{~kg}^{-1} \mathrm{~s}^{-2}\right) \text { : } \] What is the mass of Jupiter (in \( \mathrm{kg} \) ) using data from Europa's orbit? 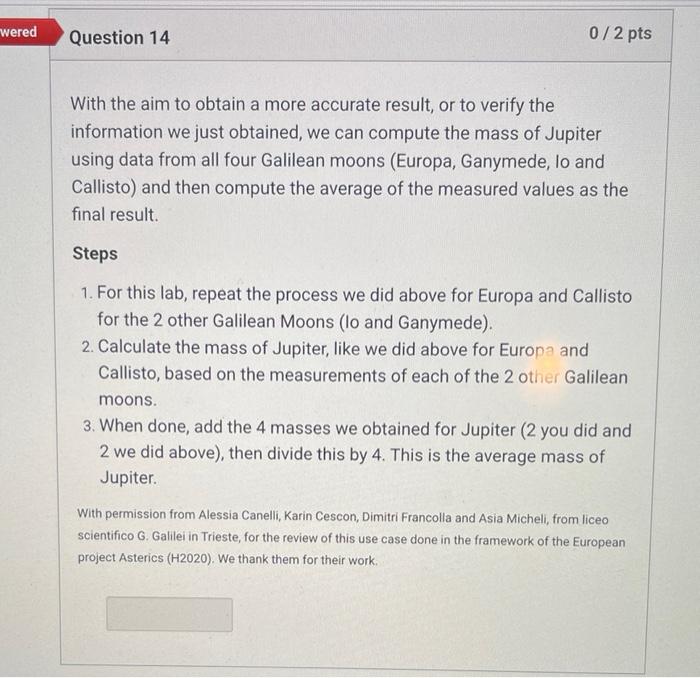With the aim to obtain a more accurate result, or to verify the information we just obtained, we can compute the mass of Jupiter using data from all four Galilean moons (Europa, Ganymede, Io and Callisto) and then compute the average of the measured values as the final result. Steps 1. For this lab, repeat the process we did above for Europa and Callisto for the 2 other Galilean Moons (Io and Ganymede). 2. Calculate the mass of Jupiter, like we did above for Europa and Callisto, based on the measurements of each of the 2 other Galilean moons. 3. When done, add the 4 masses we obtained for Jupiter ( 2 you did and 2 we did above), then divide this by 4 . This is the average mass of Jupiter. With permission from Alessia Canelli, Karin Cescon, Dimitri Francolla and Asia Micheli, from liceo scientifico G. Galilei in Trieste, for the review of this use case done in the framework of the European project Asterics (H2020). We thank them for their work.