(Solved):     this question are already solved but please explain me step by step the last 4 val ...

 

 

this question are already solved but please explain me step by step the last 4 values(V,f,v,Re) how they find from above equation

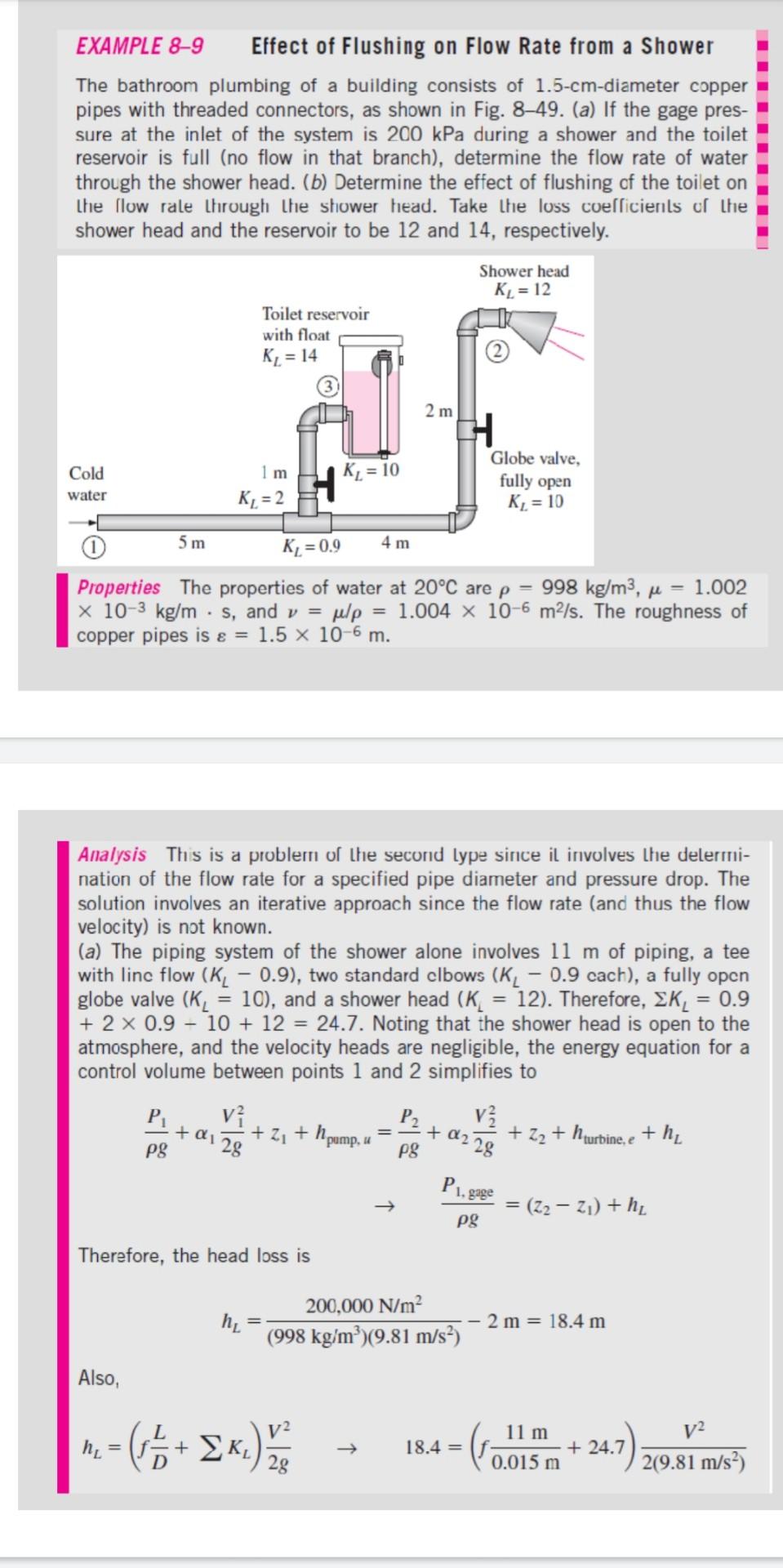
EXAMPLE 8-9 Effect of Flushing on Flow Rate from a Shower The bathroom plumbing of a building consists of \( 1.5-\mathrm{cm} \)-diameter copper pipes with threaded connectors, as shown in Fig. 8-49. (a) If the gage pressure at the inlet of the system is \( 200 \mathrm{kPa} \) during a shower and the toilet reservoir is full (no flow in that branch), determine the flow rate of water through the shower head. (b) Determine the effect of flushing of the toilet on the flow rale through the shower head. Take the loss coefficients of the shower head and the reservoir to be 12 and 14 , respectively. Properties The properties of water at \( 20^{\circ} \mathrm{C} \) are \( \rho=998 \mathrm{~kg} / \mathrm{m}^{3}, \mu=1.002 \) \( \times 10^{-3} \mathrm{~kg} / \mathrm{m} \cdot \mathrm{s} \), and \( \nu=\mu / \rho=1.004 \times 10^{-6} \mathrm{~m}^{2} / \mathrm{s} \). The roughness of copper pipes is \( \varepsilon=1.5 \times 10^{-6} \mathrm{~m} \). Analysis This is a problern of the second lype since it involves the delermination of the flow rate for a specified pipe diameter and pressure drop. The solution involves an iterative approach since the flow rate (and thus the flow velocity) is not known. (a) The piping system of the shower alone involves \( 11 \mathrm{~m} \) of piping, a tee with line flow \( \left(K_{L}-0.9\right) \), two standard clbows \( \left(K_{L}-0.9\right. \) cach), a fully open globe valve \( \left(K_{L}=10\right) \), and a shower head \( \left(K_{L}=12\right) \). Therefore, \( \Sigma K_{L}=0.9 \) \( +2 \times 0.9-10+12=24.7 \). Noting that the shower head is open to the atmosphere, and the velocity heads are negligible, the energy equation for a control volume between points 1 and 2 simplifies to \[ \begin{aligned} \frac{P_{1}}{\rho g}+\alpha_{1} \frac{V_{1}^{2}}{2 g}+z_{1}+h_{\text {pump, }, u} & =\frac{P_{2}}{\rho g}+\alpha_{2} \frac{V_{2}^{2}}{2 g}+z_{2}+h_{\text {turboine }, e}+h_{L} \\ & \rightarrow \quad \frac{P_{1, g g e}}{\rho g}=\left(z_{2}-z_{1}\right)+h_{L} \end{aligned} \] Therefore, the head loss is \[ h_{L}=\frac{200,000 \mathrm{~N} / \mathrm{m}^{2}}{\left(998 \mathrm{~kg} / \mathrm{m}^{3}\right)\left(9.81 \mathrm{~m} / \mathrm{s}^{2}\right)}-2 \mathrm{~m}=18.4 \mathrm{~m} \] Also, \[ h_{L}=\left(f \frac{L}{D}+\sum K_{L}\right) \frac{V^{2}}{2 g} \quad \rightarrow \quad 18.4=\left(f \frac{11 \mathrm{~m}}{0.015 \mathrm{~m}}+24.7\right) \frac{V^{2}}{2\left(9.81 \mathrm{~m} / \mathrm{s}^{2}\right)} \] 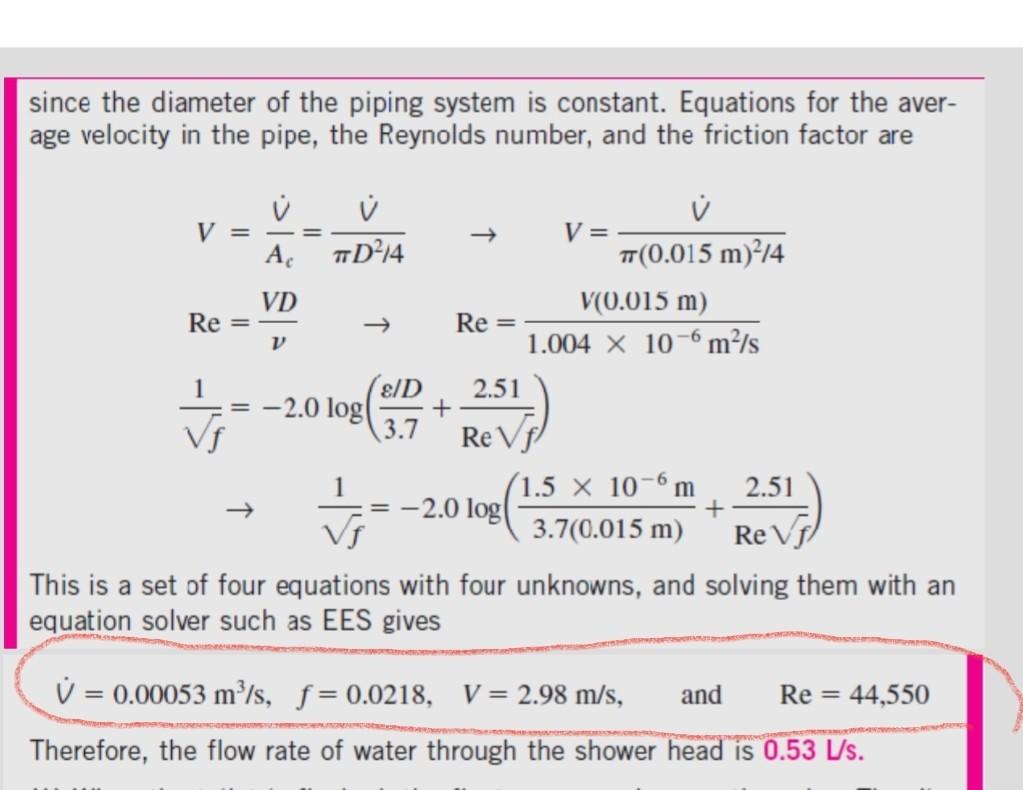since the diameter of the piping system is constant. Equations for the average velocity in the pipe, the Reynolds number, and the friction factor are \[ \begin{aligned} V & =\frac{\dot{V}}{A_{c}}=\frac{\dot{V}}{\pi D^{2} / 4} \quad \rightarrow \quad V=\frac{\dot{V}}{\pi(0.015 \mathrm{~m})^{2} / 4} \\ \operatorname{Re} & =\frac{V D}{\nu} \quad \rightarrow \quad \operatorname{Re}=\frac{V(0.015 \mathrm{~m})}{1.004 \times 10^{-6} \mathrm{~m}^{2} / \mathrm{s}} \\ \frac{1}{\sqrt{f}} & =-2.0 \log \left(\frac{\varepsilon / D}{3.7}+\frac{2.51}{\operatorname{Re} \sqrt{f}}\right) \\ & \rightarrow \quad \frac{1}{\sqrt{f}}=-2.0 \log \left(\frac{1.5 \times 10^{-6} \mathrm{~m}}{3.7(0.015 \mathrm{~m})}+\frac{2.51}{\operatorname{Re} \sqrt{f}}\right) \end{aligned} \] This is a set of four equations with four unknowns, and solving them with an equation solver such as EES gives \( \dot{V}=0.00053 \mathrm{~m}^{3} / \mathrm{s}, \quad f=0.0218, \quad V=2.98 \mathrm{~m} / \mathrm{s}, \quad \) and \( \quad \operatorname{Re}=44,550 \) Therefore, the flow rate of water through the shower head is \( 0.53 \mathrm{~L} / \mathrm{s} \).