(Solved):    1. Write an equation for the solar constant, S, i.e., the flux striking the surface ...

 

 

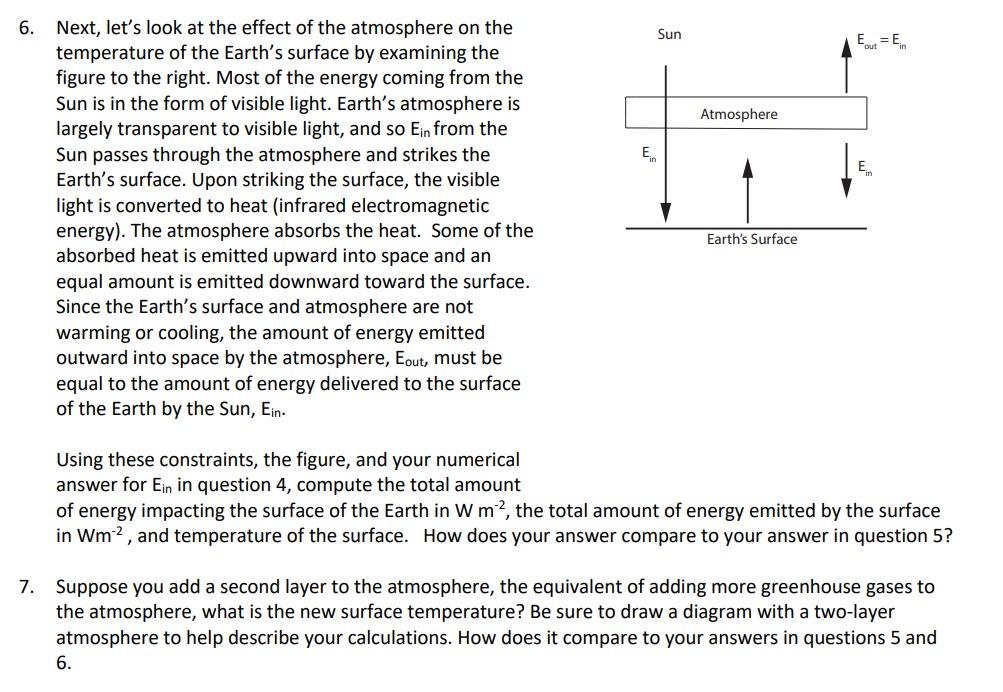
1. Write an equation for the solar constant, S, i.e., the flux striking the surface of the Earth in terms of the luminosity of the Sun, \( L_{\odot} \), and the distance between the Earth and the Sun, r. Use your equation to compute a numerical value for the solar constant in units of \( \mathrm{W} \mathrm{m}^{-2} \). 2. Write an equation for the solar power, \( \mathrm{P} \), falling on the Earth in terms of \( \mathrm{S} \) and the radius of the Earth, \( R_{\oplus} \). Hint: Use the cross-sectional area of the Earth facing the Sun. Compute a numerical value for P. Give your answer in terra watts (TW). 1 TW \( =1.0 \times 10^{12} \mathrm{~W} \). Humanity consumes about \( 16 \mathrm{TW} \). From your calculations, Is solar energy the "holy grail" of renewable energy? 3. Not all solar energy is absorbed by the Earth. Some of it is reflected into space by clouds, ice, etc. Albedo is the faction of incident solar photons reflected into space. It is represented by \( \alpha \). Modify your equation for solar power, \( \mathrm{P} \), in question 2 above to include albedo. 4. Modify your equation in question 3 above to give the solar energy going into each square meter over the entire Earth's surface, \( \mathrm{E}_{\mathrm{in}} \). Hints: Use the surface area of the entire Earth. \( \mathrm{E}_{\text {in }} \) has units of \( \mathrm{Wm}^{-2} \). Compute \( \mathrm{E}_{\text {in }} \) for \( \alpha=0.3 \). 5. Write an equation for the temperature of the Earth by balancing the solar energy into a square meter by the energy emitted by a square meter on the surface of the Earth. Compute a numerical value for the temperature of the Earth in K. Is it below the freezing temperature of ice? Hint: Remember the StefanBoltzman Law. Next, let's look at the effect of the atmosphere on the temperature of the Earth's surface by examining the figure to the right. Most of the energy coming from the Sun is in the form of visible light. Earth's atmosphere is largely transparent to visible light, and so \( \mathrm{E}_{\text {in }} \) from the Sun passes through the atmosphere and strikes the Earth's surface. Upon striking the surface, the visible light is converted to heat (infrared electromagnetic energy). The atmosphere absorbs the heat. Some of the absorbed heat is emitted upward into space and an equal amount is emitted downward toward the surface. Since the Earth's surface and atmosphere are not warming or cooling, the amount of energy emitted outward into space by the atmosphere, Eout, must be equal to the amount of energy delivered to the surface of the Earth by the Sun, Ein. Using these constraints, the figure, and your numerical answer for \( E_{\text {in }} \) in question 4, compute the total amount of energy impacting the surface of the Earth in \( \mathrm{W} \mathrm{m}^{-2} \), the total amount of energy emitted by the surface in \( \mathrm{Wm}^{-2} \), and temperature of the surface. How does your answer compare to your answer in question 5 ? Suppose you add a second layer to the atmosphere, the equivalent of adding more greenhouse gases to the atmosphere, what is the new surface temperature? Be sure to draw a diagram with a two-layer atmosphere to help describe your calculations. How does it compare to your answers in questions 5 and 6.