(Solved):   (1 point) Transform the differential equation \[ \begin{array}{l} 3 y^{\prime \prime \prim ...

 

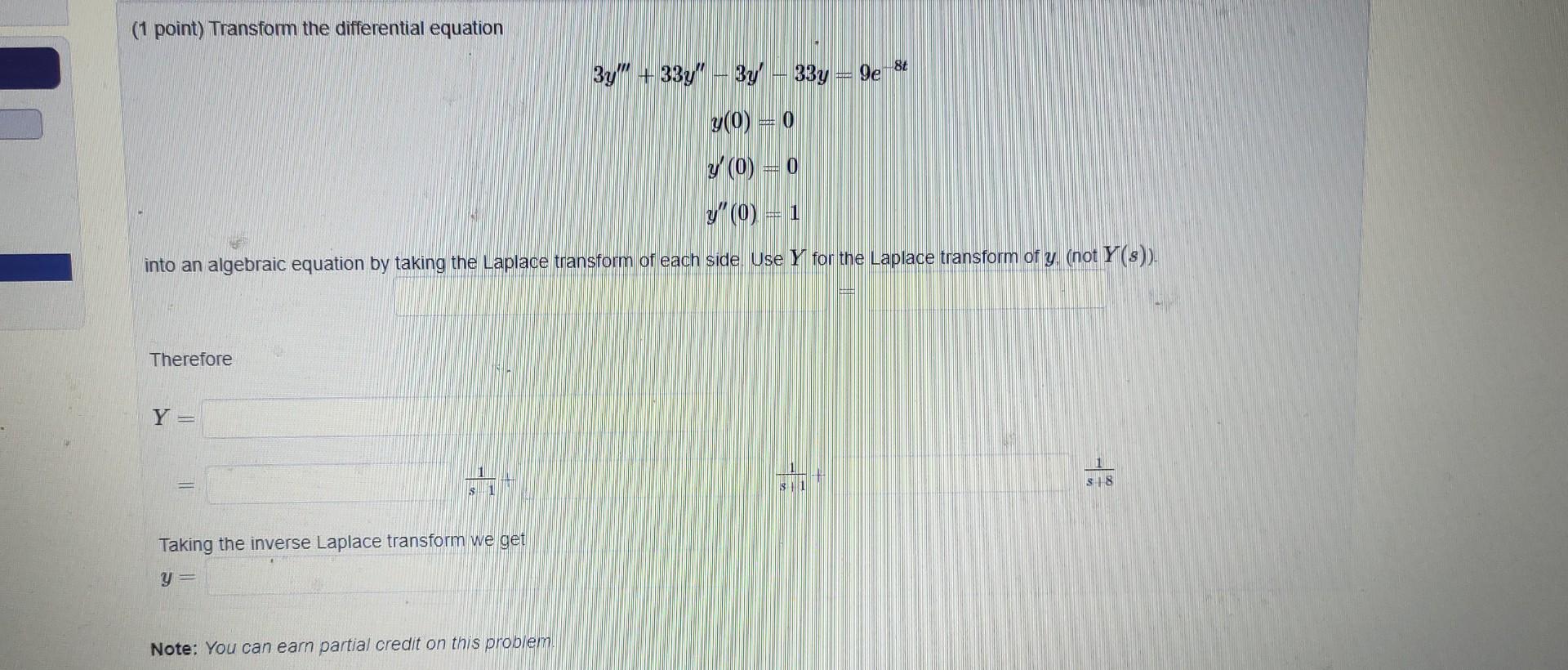
(1 point) Transform the differential equation \[ \begin{array}{l} 3 y^{\prime \prime \prime}+33 y^{\prime \prime}-3 y^{\prime}-33 y=9 e^{-8 t} \\ y(0)=0 \\ y^{\prime}(0)= 0 \\ y^{\prime \prime}(0)=1 \end{array} \] into an algebraic equation by taking the Laplace transform of each side. Use \( Y \) for the Laplace transform of \( y \). (not \( Y(s)) \). Therefore \[ Y= \] \[ \frac{1}{s+1}+\quad \frac{1}{s 18} \] Taking the inverse Laplace transform we get \[ y= \] Note: You can earn partial credit on this problem.