(Solved):   1. In a fully degenerate gas, all the particles have energies lower than the Fermi energy. ...

 

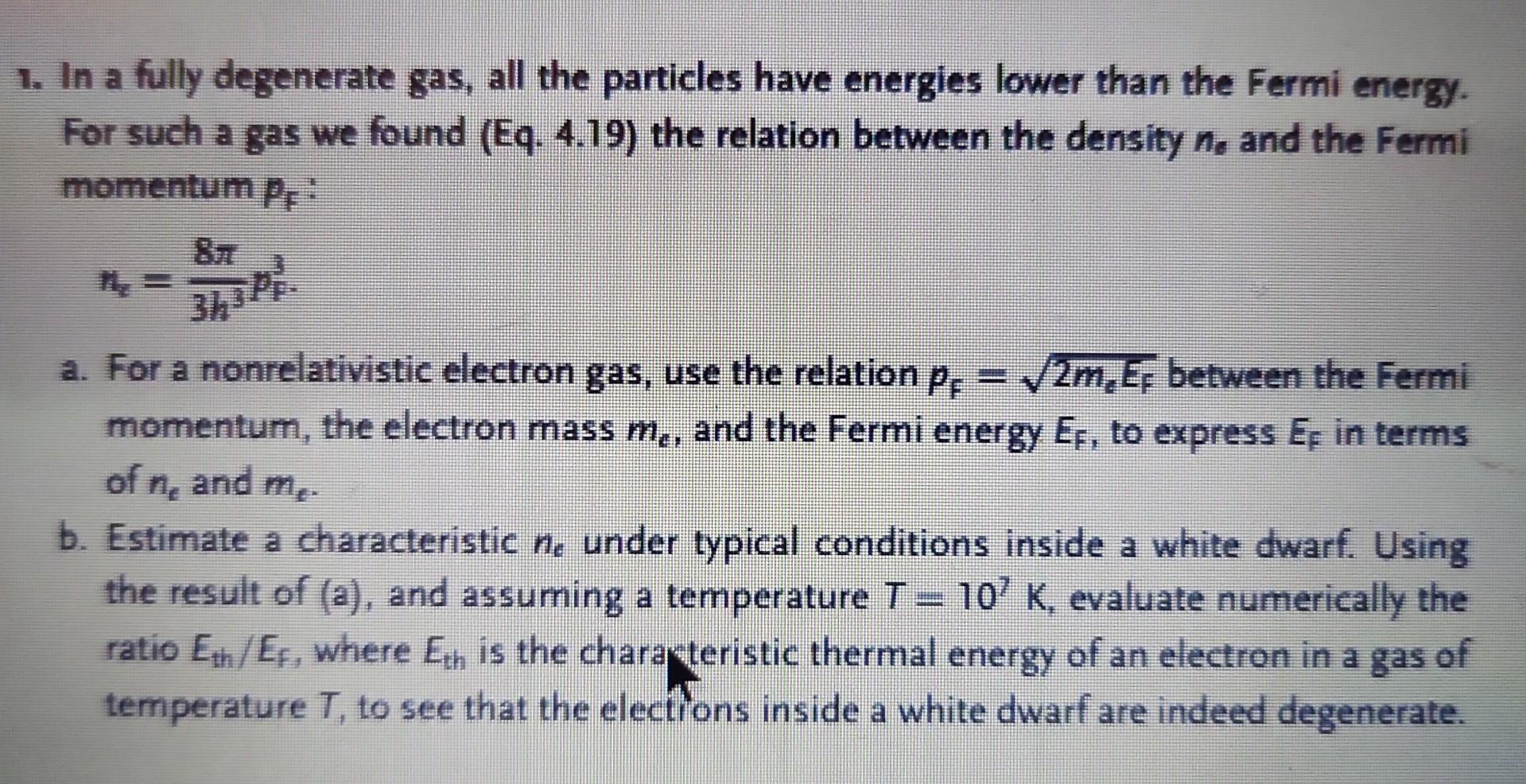
1. In a fully degenerate gas, all the particles have energies lower than the Fermi energy. For such a gas we found \( (\mathrm{Eq} .4 .19) \) the relation between the density \( n_{s} \) and the Fermi momentum \( p_{F}: \) \[ n_{z}=\frac{8 \pi}{3 h^{3}} p^{3} \] a. For a nonrelativistic electron gas, use the relation \( p_{\mathrm{F}}=\sqrt{2 m_{c} E_{\mathrm{F}}} \) between the Fermi momentum, the electron mass \( m_{c} \), and the Fermi energy \( E_{F} \), to express \( E_{F} \) in terms of \( n_{e} \) and \( m_{\epsilon} \). b. Estimate a characteristic \( n_{c} \) under typical conditions inside a white dwarf. Using the result of (a), and assuming a temperature \( T=10^{7} \mathrm{~K} \), evaluate numerically the ratio \( E_{t h} / E_{F} \), where \( E_{\text {th }} \) is the chararteristic thermal energy of an electron in a gas of temperature \( T \), to see that the elections inside a white dwarf are indeed degenerate.