(Solved): Mass transfer from a sphere: catalyst sphere of radius R is suspended in a large, motionless bod ...

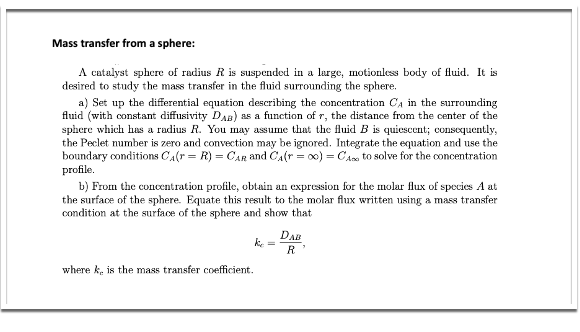
Mass transfer from a sphere: $?$ catalyst sphere of radius $R$ is suspended in a large, motionless body of fluid. It is desired to study the mass transfer in the fluid surrounding the sphere. a) Set up the differential equation describing the concentration $C_A$ in the surrounding fluid (with constant diffusivity $D_{AB}$ ) as a function of $r$, the distance from the center of the sphere which has a radius $R$. You may assume that the fluid $B$ is quiescent; consexuently, the Peclet number is zero and convection may be ignored. Integrate the equation and use the boundary conditions $C_A(r=R)=C_{AR}$ and $C_A(r=?)=C_{A?}$ to solve for the concentration profile. b) From the concentration profile, obtain an expression for the molar flux of species $A$ at the surface of the sphere. Equate this result to the molar flux written using a mass transfer condition at the surface of the sphere and show that $k_c=\frac{D_{AB}}{R},$ where $k_{\mathrm{r}}$ is the mass transfer coefficient.