(Solved): Many dairy cows now receive injections of a hormone intended to spur greater milk production. After ...

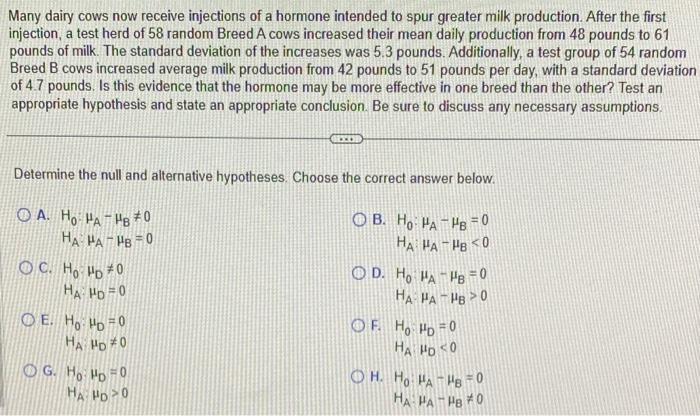
Many dairy cows now receive injections of a hormone intended to spur greater milk production. After the first injection, a test herd of 58 random Breed A cows increased their mean daily production from 48 pounds to 61 pounds of milk. The standard deviation of the increases was \( 5.3 \) pounds. Additionally, a test group of 54 random Breed \( \mathrm{B} \) cows increased average milk production from 42 pounds to 51 pounds per day, with a standard deviation of \( 4.7 \) pounds. Is this evidence that the hormone may be more effective in one breed than the other? Test an appropriate hypothesis and state an appropriate conclusion. Be sure to discuss any necessary assumptions. Determine the null and alternative hypotheses. Choose the correct answer below. A. \( H_{0} \cdot \mu_{A}-\mu_{B} \neq 0 \) B. \( H_{0}: \mu_{A}-\mu_{B}=0 \) \( \mathrm{H}_{\mathrm{A}} \cdot \mu_{\mathrm{A}}-\mu_{\mathrm{B}}=0 \) \( H_{A}: \mu_{A}-\mu_{B}<0 \) c. \( H_{0} \cdot \mu_{D} \neq 0 \) D. \( H_{0}: \mu_{A}-\mu_{B}=0 \) \( H_{A} \cdot \mu_{D}=0 \) \( H_{A} \cdot \mu_{A}-\mu_{B}>0 \) E. \( H_{0}: \mu_{D}=0 \) F. \( H_{0}: \mu_{D}=0 \) \( H_{A} \cdot \mu_{D} \neq 0 \) \( H_{A}: \mu_{\mathrm{D}}<0 \) G. \( H_{0} \cdot \mu_{D}=0 \) H. 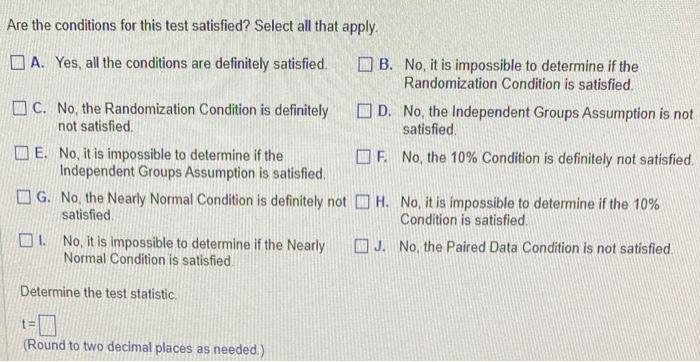\( H_{0}: \mu_{A}-\mu_{B}=0 \) \( H_{A} \cdot \mu_{D}>0 \) \( H_{A}: \mu_{A}-\mu_{B} \neq 0 \) Are the conditions for this test satisfied? Select all that apply. A. Yes, all the conditions are definitely satisfied. B. No, it is impossible to determine if the Randomization Condition is satisfied. C. No, the Randomization Condition is definitely D. No, the Independent Groups Assumption is not not satisfied. satisfied. E. No, it is impossible to determine if the F. No, the \( 10 \% \) Condition is definitely not satisfied. Independent Groups Assumption is satisfied. H. No, it is impossible to determine if the \( 10 \% \) G. No, the Nearly Normal Condition is definitely not satisfied. Condition is satisfied. 1. No, it is impossible to determine if the Nearly J. No, the Paired Data Condition is not satisfied. Normal Condition is satisfied Determine the test statistic. \( t= \) (Round to two decimal places as needed.) Round to three decimal places as needed.) Determine the proper conclusion. Choose the correct answer below. A. Fail to reject \( \mathrm{H}_{0} \) at \( \alpha=0.05 \). There is sufficient evidence that the hormone may be more effective in one breed than the other. B. Reject \( \mathrm{H}_{0} \) at \( \alpha=0.05 \). There is insufficient evidence that the hormone may be more effective in one breed than the other. C. Reject \( H_{0} \) at \( \alpha=0.05 \). There is sufficient evidence that the hormone may be more effective in one breed than the other. D. Fail to reject \( \mathrm{H}_{0} \) at \( \alpha=0.05 \). There is insufficient evidence that the hormone may be more effective in one breed than the other. Identify any necessary assumptions for this test. Select all that apply. A. It must be assumed that the cows were selected from the same farm. B. It must be assumed that the two sample sizes are more than \( 10 \% \) of the entire population of their respective breeds. C. It must be assumed that the two groups of cows are representative of their breeds. D. It must be assumed that the groups represent all breeds of cows. E. It must be assumed that the population distributions for the two breeds are Normal.