Let Tn(x) be the nth degree Taylor polynomial for sinz centered at 0. Let ( T_{n}(x) ) be the ( n ) th-degree Taylor polynomial for ( sin x ) centered at 0 . Use Taylor's Inequality to find an ( n ) that makes ( left| sin x-T_{n}(x) right| leq 0.001 ) for ( |x| leq 1 / 2 ). Recall Taylor's inequality: ( left|f solutionspile.com

Question

Let Tn(x) be the nth degree Taylor polynomial for sinz centered at 0.

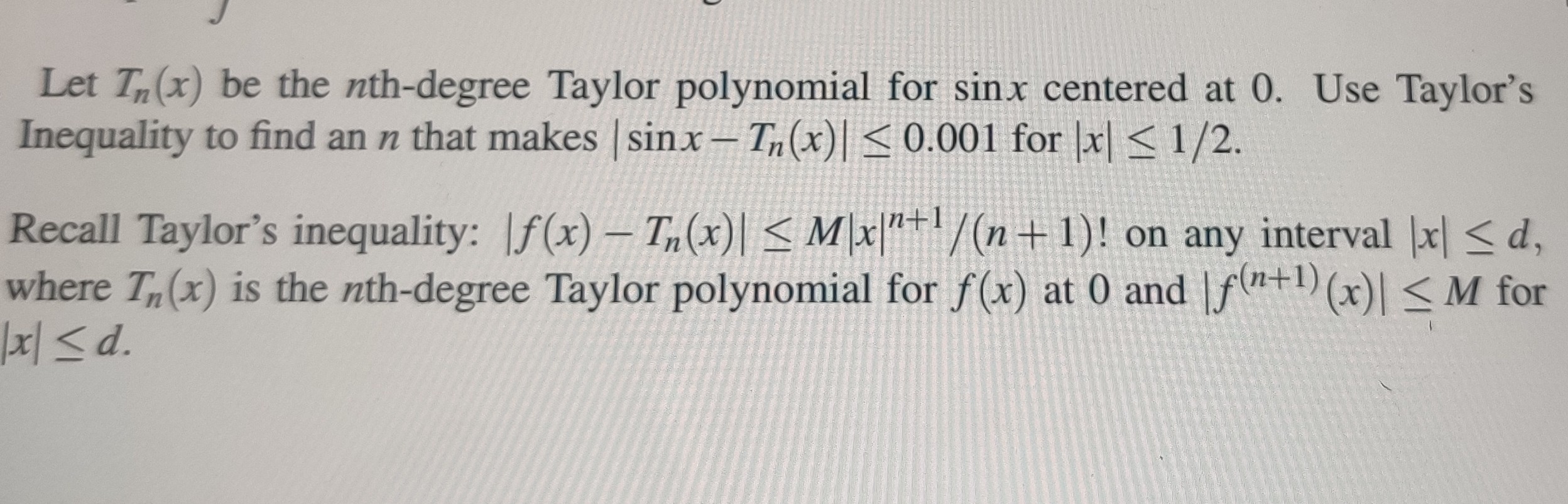
student submitted image, transcription available below

Let \\( T_{n}(x) \\) be the \\( n \\) th-degree Taylor polynomial for \\( \\sin x \\) centered at 0 . Use Taylor's Inequality to find an \\( n \\) that makes \\( \\left|\\sin x-T_{n}(x)\\right| \\leq 0.001 \\) for \\( |x| \\leq 1 / 2 \\). Recall Taylor's inequality: \\( \\left|f(x)-T_{n}(x)\\right| \\leq M|x|^{n+1} /(n+1) \\) ! on any interval \\( |x| \\leq d \\), where \\( T_{n}(x) \\) is the \\( n \\) th-degree Taylor polynomial for \\( f(x) \\) at 0 and \\( \\left|f^{(n+1)}(x)\\right| \\leq M \\) for \\( |x| \\leq d \\)

Accepted Answer

Expert Answer to - Let Tn(x) be the nth degree Taylor polynomial for sinz centered at 0.  Let   ( T_{n}(x)   ) be the

Answer

Solution for - Let Tn(x) be the nth degree Taylor polynomial for sinz centered at 0.  Let   ( T_{n}(x)   ) be the

Answer

This an additional answer to - Let Tn(x) be the nth degree Taylor polynomial for sinz centered at 0.  Let   ( T_{n}(x)   ) be the

(Solved): Let Tn(x) be the nth degree Taylor polynomial for sinz centered at 0. Let \\( T_{n}(x) \\) be the \ ...

View Expert Answer

Expert Answer

Buy This Answer $5

Place Order

We Provide Services Across The Globe