(Solved): Let \( Q \) be the region bounded by the parallelepiped whose faces are the planes of equations \( ...

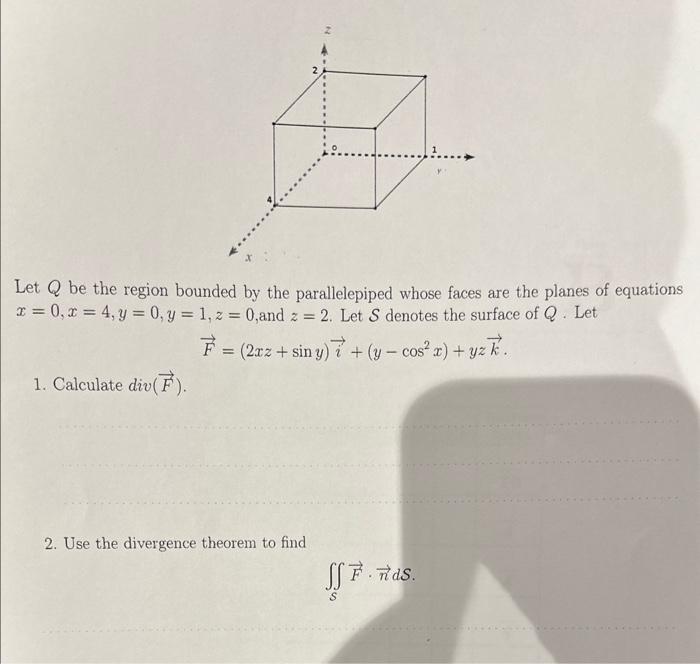
Let \( Q \) be the region bounded by the parallelepiped whose faces are the planes of equations \( x=0, x=4, y=0, y=1, z=0 \), and \( z=2 \). Let \( \mathcal{S} \) denotes the surface of \( Q \). Let \[ \vec{F}=(2 x z+\sin y) \vec{i}+\left(y-\cos ^{2} x\right)+y z \vec{k} \text {. } \] 1. Calculate \( \operatorname{div}(\vec{F}) \). 2. Use the divergence theorem to find \[ \iint_{\mathcal{S}} \vec{F} \cdot \vec{n} d S . \]