(Solved): Let \( D \subseteq \mathbb{R}^{2} \) be an open set with regular, \( C^{1} \) boundary, \( \partia ...

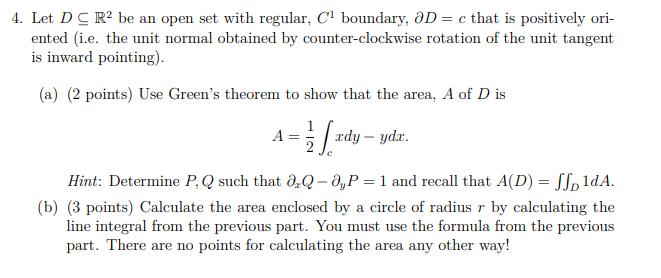
Let \( D \subseteq \mathbb{R}^{2} \) be an open set with regular, \( C^{1} \) boundary, \( \partial D=c \) that is positively oriented (i.e. the unit normal obtained by counter-clockwise rotation of the unit tangent is inward pointing). (a) (2 points) Use Green's theorem to show that the area, \( A \) of \( D \) is \[ A=\frac{1}{2} \int_{c} x d y-y d x \] Hint: Determine \( P, Q \) such that \( \partial_{x} Q-\partial_{y} P=1 \) and recall that \( A(D)=\iint_{D} 1 d A \). (b) (3 points) Calculate the area enclosed by a circle of radius \( r \) by calculating the line integral from the previous part. You must use the formula from the previous part. There are no points for calculating the area any other way!