(Solved): Let \( C \) be the following closed curve in the \( x y \) plane: \( C \) con ...

???????

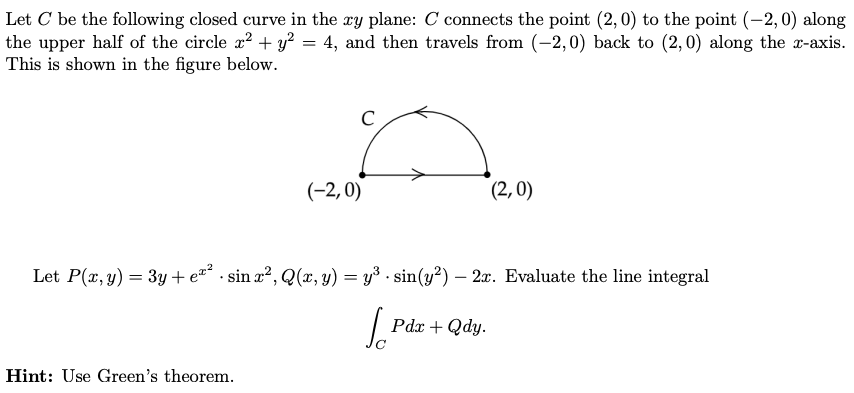
Let \( C \) be the following closed curve in the \( x y \) plane: \( C \) connects the point \( (2,0) \) to the point \( (-2,0) \) along the upper half of the circle \( x^{2}+y^{2}=4 \), and then travels from \( (-2,0) \) back to \( (2,0) \) along the \( x \)-axis. This is shown in the figure below. Let \( P(x, y)=3 y+e^{x^{2}} \cdot \sin x^{2}, Q(x, y)=y^{3} \cdot \sin \left(y^{2}\right)-2 x \). Evaluate the line integral \[ \int_{C} P d x+Q d y . \] Hint: Use Green's theorem.