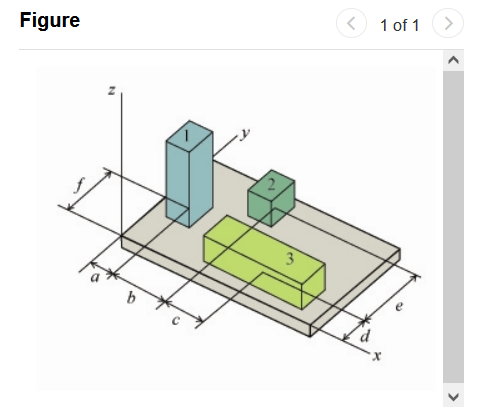
(Solved): Learning Goal: To determine the center of gravity of a composite body using the principle of superpo ...

Learning Goal: To determine the center of gravity of a composite body using the principle of superposition. The layout shown is a representation of a machine shop. Components 1 and 2 have masses of m1=260kg and m2=225kg , respectively. Component 3 must be treated as a distributed load, which is w=175 kg/m2, determined by the area of contact between the component and the shop floor. The dimensions shown have been measured to be a=0.550 m, b=3.00 m, c=1.90 m, d=0.750 m, e=3.70 m, and f=1.10 m. These dimensions represent the x and y components of the locations of the centers of gravity for the respective components. Component 3 has x,y cross-sectional dimensions of x3=1.00 m and y3=0.150 m. Assume the components experience uniform weight distribution in all principle directions. The dimensions a through f locate the centroid of the respective component from the y?z plane and x?z plane.(Figure 1)

Figure 1 of 1 Part A - The \( x \) Component of the Center of Gravity of All the Components Determine the \( x \) component of the center of gravity of all the components. Express your answer to three significant figures and include the appropriate units. Part B - The \( y \) Component of the Center of Gravity of All the Components Determine the \( y \) component of the center of gravity of all the components. Express your answer to three significant figures and include the appropriate units. Part C - The \( z \) Component of the Center of Gravity of All the Components If the heights of the components are given as \( z_{1}=2.00 \mathrm{~m}, z_{2}=0.600 \mathrm{~m} \), and \( z_{3}=0.100 \mathrm{~m} \), determine the \( z \) component of the center of gravity of all the components. Express your answer to three significant figures and include the appropriate units.