(Solved): (Lagrange interpolation formula, 5 points) We write Lagrange's interpolation formula as \[ p_{n}(x ...

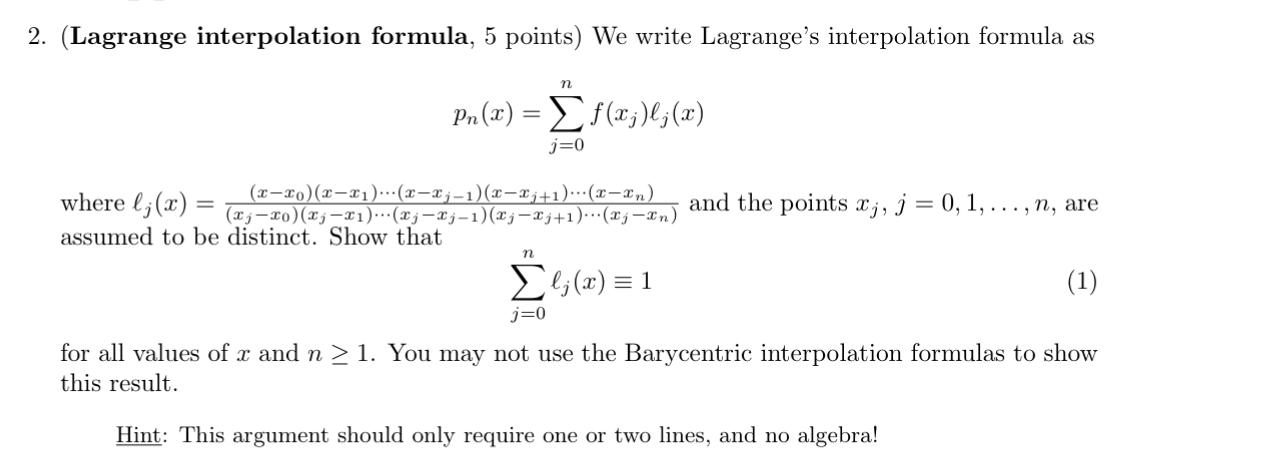
(Lagrange interpolation formula, 5 points) We write Lagrange's interpolation formula as \[ p_{n}(x)=\sum_{j=0}^{n} f\left(x_{j}\right) \ell_{j}(x) \] where \( \ell_{j}(x)=\frac{\left(x-x_{0}\right)\left(x-x_{1}\right) \cdots\left(x-x_{j-1}\right)\left(x-x_{j+1}\right) \cdots\left(x-x_{n}\right)}{\left(x_{j}-x_{0}\right)\left(x_{j}-x_{1}\right) \cdots\left(x_{j}-x_{j-1}\right)\left(x_{j}-x_{j+1}\right) \cdots\left(x_{j}-x_{n}\right)} \) and the points \( x_{j}, j=0,1, \ldots, n \), are assumed to be distinct. Show that \[ \sum_{j=0}^{n} \ell_{j}(x) \equiv 1 \] for all values of \( x \) and \( n \geq 1 \). You may not use the Barycentric interpolation formulas to show this result. Hint: This argument should only require one or two lines, and no algebra!