(Solved): Just need the last part answered (2 points) torsion lever 02 Dimensions: Problem Statement: Lever \ ...

Just need the last part answered

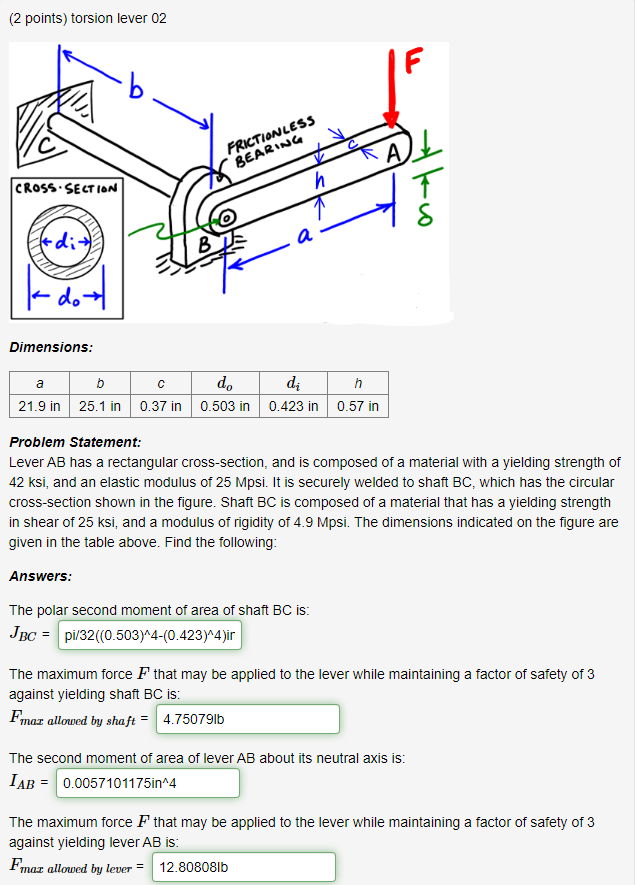
(2 points) torsion lever 02 Dimensions: Problem Statement: Lever \( A B \) has a rectangular cross-section, and is composed of a material with a yielding strength of \( 42 \mathrm{ksi} \), and an elastic modulus of \( 25 \mathrm{Mpsi} \). It is securely welded to shaft \( \mathrm{BC} \), which has the circular cross-section shown in the figure. Shaft BC is composed of a material that has a yielding strength in shear of \( 25 \mathrm{ksi} \), and a modulus of rigidity of \( 4.9 \mathrm{Mpsi} \). The dimensions indicated on the figure are given in the table above. Find the following: Answers: The polar second moment of area of shaft \( \mathrm{BC} \) is: \[ J_{B C}= \] The maximum force \( F \) that may be applied to the lever while maintaining a factor of safety of 3 against yielding shaft \( \mathrm{BC} \) is: \( F_{\text {max allowed by shaft }}= \) The second moment of area of lever \( \mathrm{AB} \) about its neutral axis is: \[ I_{A B}= \] The maximum force \( F \) that may be applied to the lever while maintaining a factor of safety of 3 against yielding lever \( \mathrm{AB} \) is: \( F_{\text {max allowed by lever }}= \) 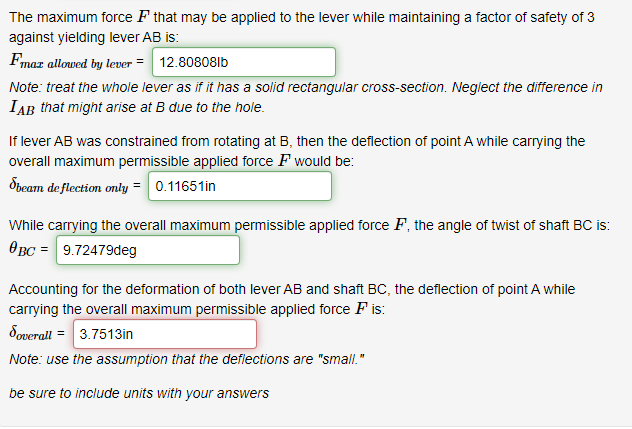The maximum force \( F \) that may be applied to the lever while maintaining a factor of safety of 3 against yielding lever \( A B \) is: \( F_{\text {max allowed by lever }}= \) Note: treat the whole lever as if it has a solid rectangular cross-section. Neglect the difference in \( I_{A B} \) that might arise at \( B \) due to the hole. If lever \( \mathrm{AB} \) was constrained from rotating at \( \mathrm{B} \), then the deflection of point \( \mathrm{A} \) while carrying the overall maximum permissible applied force \( F \) would be: \( \delta_{\text {beam deflection only }}= \) While carrying the overall maximum permissible applied force \( F \), the angle of twist of shaft BC is: \[ \theta_{B C}= \] Accounting for the deformation of both lever AB and shaft BC, the deflection of point A while carrying the overall maximum permissible applied force \( F \) is: \[ \delta_{\text {overall }}= \] Note: use the assumption that the deflections are "small." be sure to include units with your answers