(Solved): - In the Cartesian coordinate system any vector can - Using the definition of the dot product, gen ...

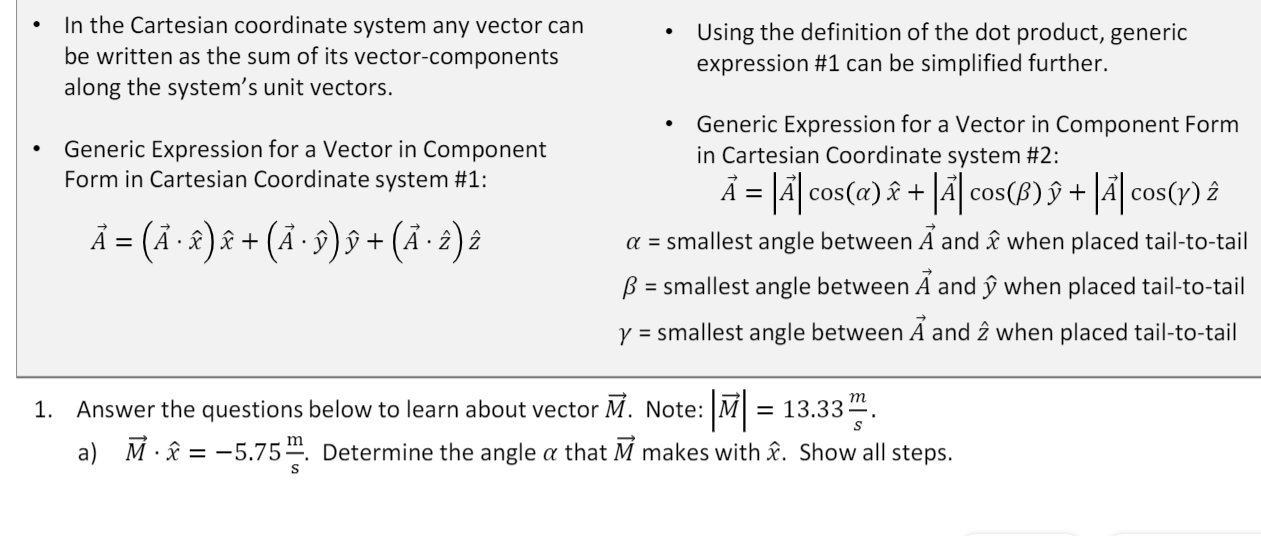
- In the Cartesian coordinate system any vector can - Using the definition of the dot product, generic be written as the sum of its vector-components expression \#1 can be simplified further. along the system's unit vectors. - Generic Expression for a Vector in Component Form - Generic Expression for a Vector in Component in Cartesian Coordinate system \#2: Form in Cartesian Coordinate system \#1: $$\vec{A}=?\vec{A}?\cos (?)\hat{x}+?\vec{A}?\cos (?)\hat{y}+?\vec{A}?\cos (?)\hat{z}$$ $$\vec{A}=(\vec{A}?\hat{x})\hat{x}+(\vec{A}?\hat{y})\hat{y}+(\vec{A}?\hat{z})\hat{z}?=$$ smallest angle between $$\vec{A}$$ and $$\hat{x}$$ when placed tail-to-tail $$?=$$ smallest angle between $$\vec{A}$$ and $$\hat{y}$$ when placed tail-to-tail $$?=$$ smallest angle between $$\vec{A}$$ and $$\hat{z}$$ when placed tail-to-tail 1. Answer the questions below to learn about vector $$\vec{M}$$. Note: $$?\vec{M}?=13.33\frac{\mathrm{m}}{\mathrm{s}}$$. a) $$\vec{M}?\hat{x}=?5.75\frac{\mathrm{m}}{\mathrm{s}}$$. Determine the angle $$?$$ that $$\vec{M}$$ makes with $$\hat{x}$$. Show all steps.