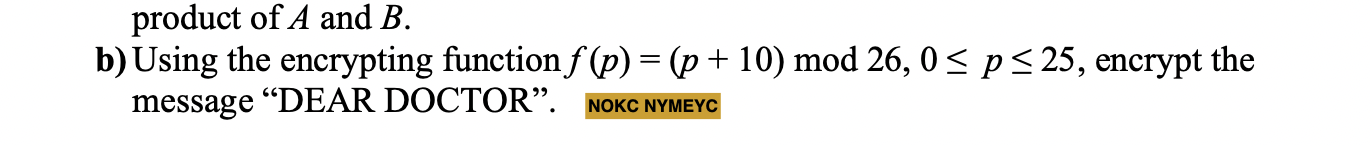Home /
Expert Answers /
Computer Science /
i-need-explanation-nbsp-and-correction-to-these-questions-q-2-the-negation-of-p-wedge-q-pa508
(Solved): I need explanation and correction to these questions Q-2: The negation of \( (P \wedge Q) \ ...
I need explanation and correction to these questions
Q-2: The negation of \( (P \wedge Q) \rightarrow R \) is a) \( \neg(P \wedge Q) \rightarrow \neg R \) b) \( \neg R \rightarrow \neg(P \wedge Q) \) c) \( R \rightarrow(P \wedge Q) \) d) \( P \wedge Q \wedge \neg R \) e) None of the above
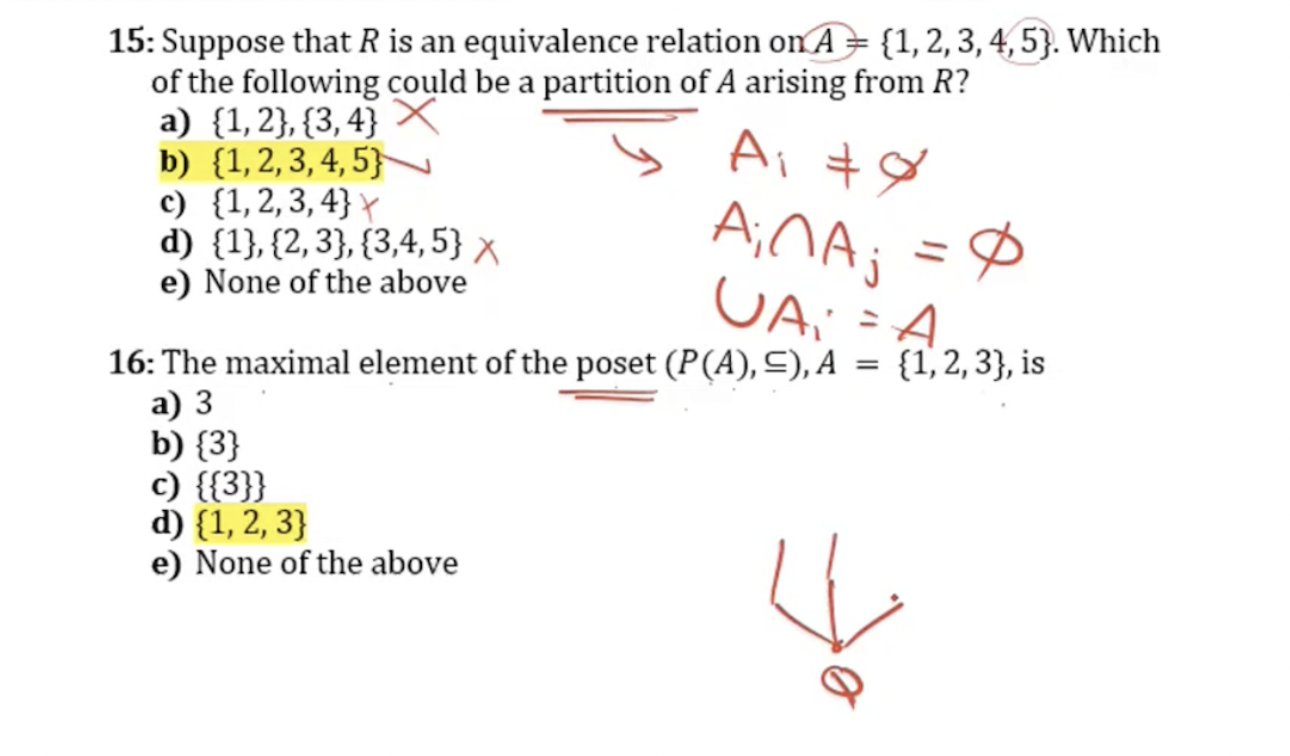
15: Suppose that \( R \) is an equivalence relation on \( A=\{1,2,3,4,5\} \). Which of the following could be a partition of \( A \) arising from \( R \) ? a) \( \{1,2\},\{3,4\} \chi \quad \stackrel{\varnothing}{\downarrow}\{1,2,3,4,5\} \) c) \( \{1,2,3,4\} \times \) d) \( \{1\},\{2,3\},\{3,4,5\} \chi \) e) None of the above \( A_{i} \cap A_{j}=\varnothing \) \( \cup A_{i^{\prime}}=A \) 16: The maximal element of the poset \( (P(A), \subseteq), A=\{1,2,3\} \), is a) 3 b) \( \{3\} \) c) \( \{\{3\}\} \) d) \( \{1,2,3\} \) e) None of the above
Q-1: If \( S \) is a set such that \( |S|=3 \), then \( |P(S)|= \) a) 9 b) 8 c) 6 d) 3 e) None of the above Q-2: The contrapositive of \( (P \wedge Q) \rightarrow \neg R \) is a) \( R \rightarrow \neg P \wedge \neg Q \) b) \( \neg(P \wedge Q) \rightarrow R \) c) \( R \rightarrow \neg P \vee \neg Q \) d) \( \neg P \vee \neg Q \rightarrow R \) e) None of the above Q-3: If \( a \equiv 7 \bmod 13 \), then \( a \) may equal a) 0 b) 14 c) 19 d) \( -19 \) e) None of the above Q-4: If \( A=\{1,2,3\} \) and \( B=\{4,5,6\} \), then \( A \oplus B= \) a) \( \Phi \) b) \( \{1,2,3\} \) c) \( \{4,5,6\} \) d) \( \{1,2,3,4,5,6\} \) e) None of the above Q-5: The hexadecimal representation of \( (1101101)_{2} \) is a) \( 6 \mathrm{D} \) b) D5 c) 155 d) 551
Q-1: [8+8 marks] a) Determine whether each of the following is TRUE or FALSE: i. \( 1+2=5 \) if and only if \( 3-1=1 \). F ii. \( 3 \mid 19 \) or \( 14 \equiv 23(\bmod 4) \). iii. \( x+5>9 \) for every real number \( x \). F iv. \( \neg \exists x(2 x=x) \), domain is the set of integers. b) Show that the statement \( \neg((P \wedge \neg Q) \rightarrow P \vee Q) \) is a contradiction using: i. Truth table. solved ii. Logic laws. Q-2: [4+6+6 marks] a) Find the bit-wise XOR of strings 11010011 and 10111010 . 01101001 b) Let \( B(x), E(x) \) and \( G(x) \) be the statements " \( x \) is a book" " \( x \) is expensive" and " \( x \) is good" respectively. Express each of the following statements using quantifiers and logical connectives, where the universe of discourse is the set of all objects: i. All expensive books are good. " will used A instead the quantifiers "All", and E instead of "Some" ii. Some good books are not expensive. \( \quad E E(x) E(x)=\in(x) \) c) Show that \( (B-A) \cup(C-A)=(B \cup C)-A \). Need to be solved Q-3: [6+4+6 marks] a) Find \( a, b \) and \( c \) if \( \left\{\begin{array}{l}a=43 \operatorname{div} 6 \\ a+b=-51 \bmod 6 . \\ a+c=64 \bmod 8 \\ c=4 \\ b=1\end{array}\right. \) b) Let \( a=2^{3} \cdot 3^{2} \cdot 5 \) and \( b=2^{2} \cdot 3^{3} \cdot 7^{2} \). Find \( \operatorname{GCD}(a, b) \) and \( \operatorname{LCM}(a, b) \). \( \mathrm{acD}=32 \) \( \operatorname{LCM}=7560 \) c) Are the numbers 96 and 175 relatively primes? Explain. NO, both of then accept division. \( 96 / 3 \) and \( 175 / 5 \) product of \( A \) and \( B \). b) Using the encrypting function \( f(p)=(p+10) \bmod 26,0 \leq p \leq 25 \), encrypt the message "DEAR DOCTOR".
product of \( A \) and \( B \). b) Using the encrypting function \( f(p)=(p+10) \bmod 26,0 \leq p \leq 25 \), encrypt the message "DEAR DOCTOR".
Expert Answer
Hey mate here is your answer ????????... Hope you would like it... Answer 2 d) P ^ Q ^ ¬R Answer 15 Equivalence relations on a set A are recursive, symmet
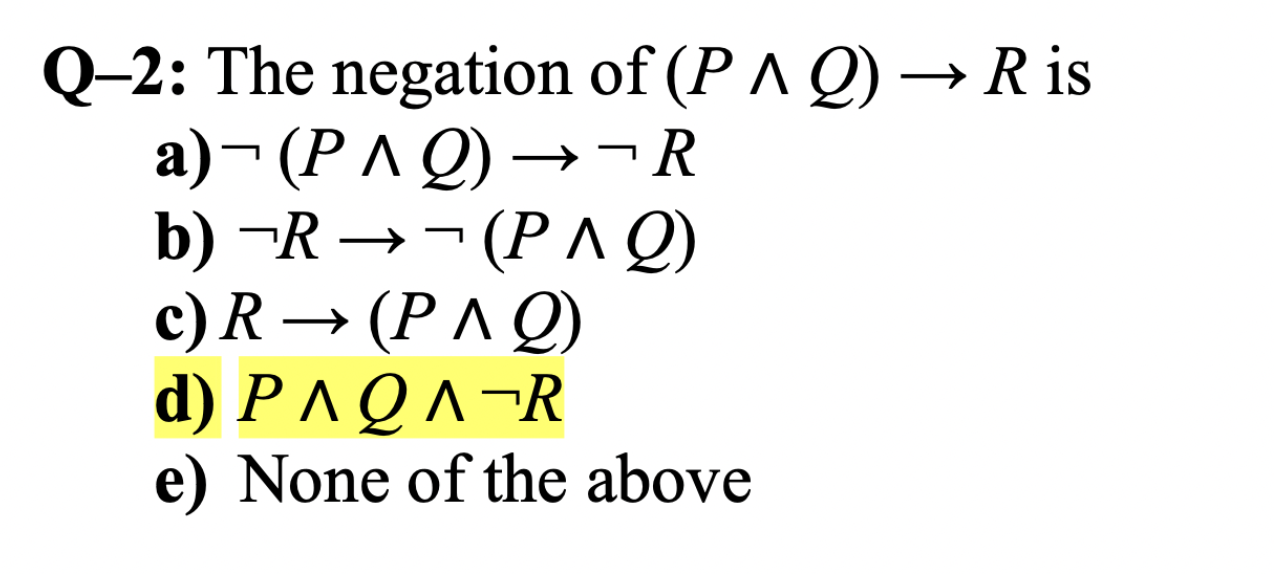

![Q-1: [8+8 marks]
a) Determine whether each of the following is TRUE or FALSE:
i. \( 1+2=5 \) if and only if \( 3-1=1 \). F
ii](https://media.cheggcdn.com/media/859/8593fdf9-d312-400c-b89d-0fdb3c2cb689/php8EGcBC)