(Solved): i) Calculate the self-weight of the concrete structure, in units of kN/m, in the direction of the br ...

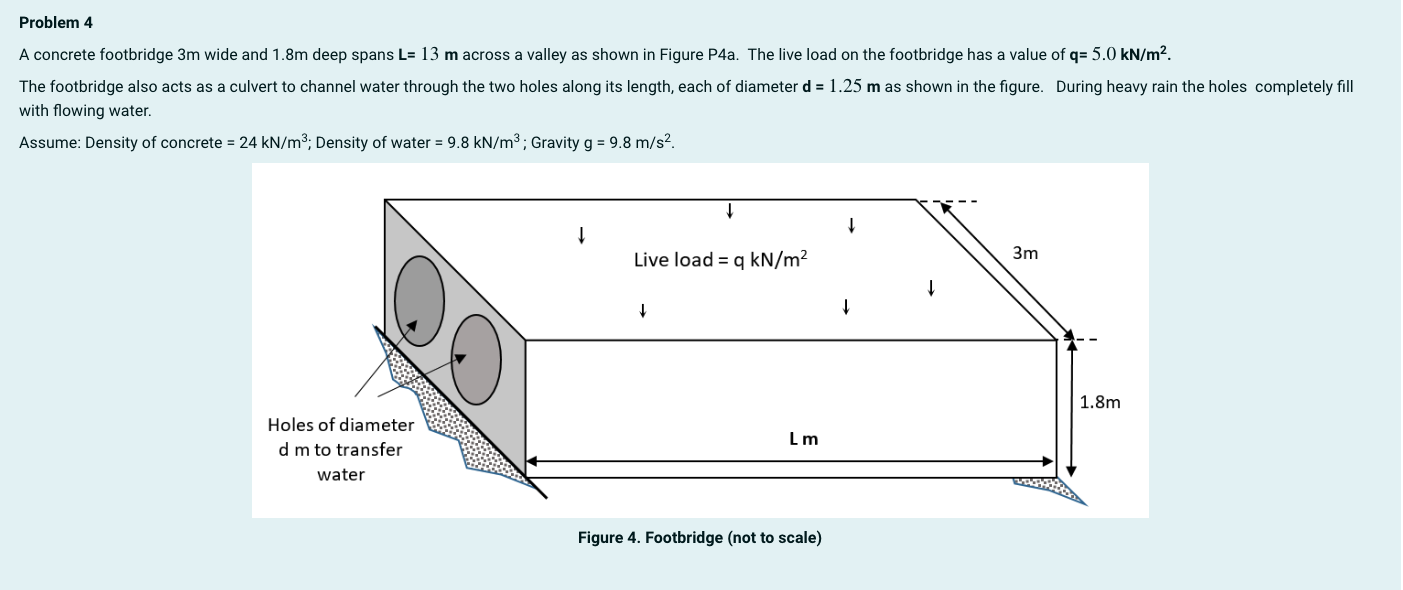
i) Calculate the self-weight of the concrete structure, in units of kN/m, in the direction of the bridge span. Provide your answer to at least 2 decimal places

ii) Calculate the maximum weight of the water that can be carried by the bridge at the one time. Provide your answer in units of kN/m to at least 2 decimal places. [1 mark]

iii) Calculate live load acting on the bridge in units of kN/m, in the direction of the bridge span. Provide your answer to at least to 2 decimal places. [1 mark]

iv) Based on the loading in parts a) to c) what is the ultimate limit state (ULS) loading that you would design the bridge to carry? Provide your answer in units of kN/m to at least 2 decimal places. [0.5 mark]

v) Based on the loading in parts a) to c) what is the serviceability limit state (SLS) loading that you would design the bridge to carry? Provide your answer in units of kN/m to at least 2 decimal places. [0.5 mark]

vi) When the water flow exceeds the bridge capacity the bridge is shut to traffic using gates at either end of the bridge. A typical gate 3m long and H = 0.6m high is shown in Figure P4b. It is made of solid rod members that individually weigh 6 kg/m. Calculate the weight of a gate in kN/m along its 3m length, assuming this load to act as a uniformly distributed load on the bridge. Provide your answer to at least 3 decimal places. [1 mark]