(Solved): how to find the yeta=.4037 EXAMPLE 2.11 An underdamped shock absorber is to be designed for a motorc ...

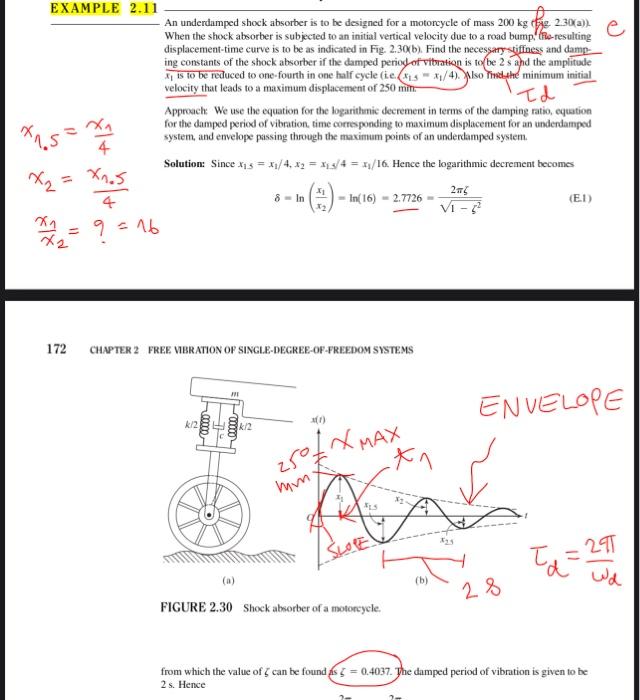
EXAMPLE 2.11 An underdamped shock absorber is to be designed for a motorcycle of mass 200 kg e 2.30(a)). When the shock absorber is subjected to an initial vertical velocity due to a road bump, e-resulting displacement-time curve is to be as indicated in Fig. 2.30(b). Find the necessary stiffness and damp ing constants of the shock absorber if the damped period of vibration is to be 2 s and the amplitude x is to be reduced to one-fourth in one half cycle (ie 15x1/4). Also The the minimum initial velocity that leads to a maximum displacement of 250 min. 'Id. *?5= x/? Approach: We use the equation for the logarithmic decrement in terms of the damping ratio, equation for the damped period of vibration, time corresponding to maximum displacement for an underdamped system, and envelope passing through the maximum points of an underdamped system. 4 Solution: Since x?5 = X?/4, X? = x?/4 = x?/16. Hence the logarithmic decrement becomes xn.s 4 8 - In -In(16)-2.7726- 3?, ??? VI-2 (E.I) 2/?? = 9 = 16 172 CHAPTER 2 FREE VIBRATION OF SINGLE-DEGREE-OF-FREEDOM SYSTEMS m ENVELOPE x(1) XMAX SLOPE =297 (a) (b) Wa FIGURE 2.30 Shock absorber of a motorcycle. 28 from which the value of g can be found as = 0.4037. The damped period of vibration is given to be 2 s. Hence 11 k/2 axo 00000 k/2 50 mm. _*^ 323 ?????