(Solved): Goal Apply Coulomb's law in two dimensions. Problem Consider three point charges at the corners of ...

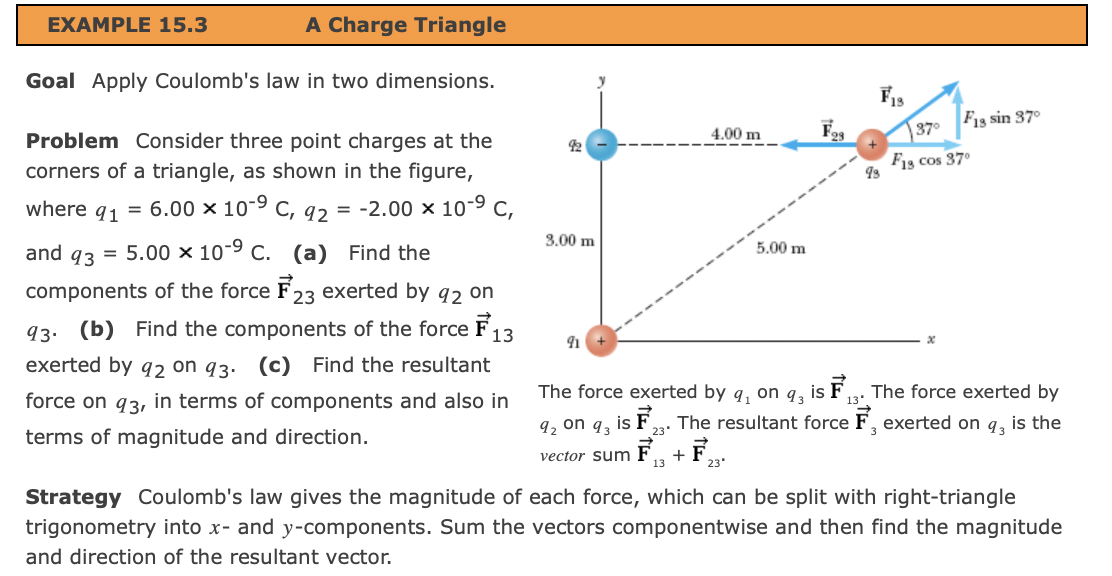
Goal Apply Coulomb's law in two dimensions. Problem Consider three point charges at the corners of a triangle, as shown in the figure, where $q_1=6.00\times 10^{?9}\mathrm{C},q_2=?2.00\times 10^{?9}\mathrm{C}$, and $q_3=5.00\times 10^{?9}\mathrm{C}$. (a) Find the components of the force ${\overrightarrow{\mathbf{F}}}_{23}$ exerted by $q_2$ on $q_3$. (b) Find the components of the force ${\overrightarrow{\mathbf{F}}}_{13}$ exerted by $q_2$ on $q_3$. (c) Find the resultant force on $q_3$, in terms of components and also in terms of magnitude and direction. The force exerted by $q_1$ on $q_3$ is ${\overrightarrow{\mathbf{F}}}_{13}$. The force exerted by $q_2$ on $q_3$ is ${\overrightarrow{\mathbf{F}}}_{23}$. The resultant force ${\overrightarrow{\mathbf{F}}}_3$ exerted on $q_3$ is the vector sum ${\overrightarrow{\mathbf{F}}}_{13}+{\overrightarrow{\mathbf{F}}}_{23^{?}}$ Strategy Coulomb's law gives the magnitude of each force, which can be split with right-triangle trigonometry into $x$ - and $y$-components. Sum the vectors componentwise and then find the magnitude and direction of the resultant vector. (a) Find the components of the force exerted by $q_2$ on $q_3$. Find the magnitude of ${\overrightarrow{\mathbf{F}}}_{23}$ with Coulomb's law: Coulomb's law: Because ${\overrightarrow{\mathbf{F}}}_{23}$ is horizontal and points in the negative $x$-direction, the negative of the magnitude gives the $x$-component, and the $y$-component is zero. $\begin{array}{l}{F}_{23}=k_e\frac{?q_2??q_3?}{r^2}\\ =\left(8.99\times 10^9\mathrm{N}?{\mathrm{m}}^2/{\mathrm{C}}^2\right)\frac{\left(2.00\times 10^{?9}\mathrm{C}\right)\left(5.00\times 10^{?9}\mathrm{C}\right)}{(4.00\mathrm{m}{)}^2}\end{array}$ $\begin{array}{l}{F}_{23}=5.62\times 10^{?9}\mathrm{N}\\ F_{23x}=?5.62\times 10^{?9}\mathrm{N}\\ F_{23y}=0\end{array}$ (b) Find the components of the force exerted by $q_1$ on $q_3$. Find the magnitude of ${\overrightarrow{\mathbf{F}}}_{13}$ : $\begin{array}{l}{F}_{13}=k_e\frac{?q_1??q_3?}{r^2}\\ =\left(8.99\times 10^9\mathrm{N}?{\mathrm{m}}^2/{\mathrm{C}}^2\right)\frac{\left(6.00\times 10^{?9}\mathrm{C}\right)\left(5.00\times 10^{?9}\mathrm{C}\right)}{(5.00\mathrm{m}{)}^2}\\ F_{13}=1.08\times 10^{?8}\mathrm{N}\\ F_{13x}=F_{13}\cos ?=\left(1.08\times 10^{?8}\mathrm{N}\right)\cos \left(37^{?}\right)=8.63\times 10^{?9}\mathrm{N}\\ F_{13y}=F_{13}\sin ?=\left(1.08\times 10^{?8}\mathrm{N}\right)\sin \left(37^{?}\right)=6.50\times 10^{?9}\mathrm{N}\end{array}$ (c) Find the components of the resultant vector. Sum the $x$-components to find the resultant $F_x$ : Sum the $y$-components to find the resultant $F_y$ : Find the magnitude of the resultant force on the charge $q_3$, using the Pythagorean theorem. Find the angle the resultant force makes with respect to the positive $x$ axis. $F_x=?5.62\times 10^{?9}\mathrm{N}+8.63\times 10^{?9}\mathrm{N}=3.01\times 10^{?9}\mathrm{N}$ $F_y=0+6.50\times 10^{?9}\mathrm{N}=6.50\times 10^{?9}\mathrm{N}$ $\begin{array}{l}=7.16\times 10^{?9}\mathrm{N}\\ ?={\tan }^{?1}\left(\frac{F_y}{F_x}\right)={\tan }^{?1}\left(\frac{6.50\times 10^{?9}\mathrm{N}}{3.01\times 10^{?9}\mathrm{N}}\right)=65.2^{?}\end{array}$ Use the worked example above to help you solve this problem. Consider three point charges at the corners of a triangle, as shown in the figure, where $q_1=5.71\times 10^{?9}\mathrm{C},q_2=?2.06\times 10^{?9}\mathrm{C}$, and $q_3=4.63\times 10^{?8}$ C. (a) Find the components of the force ${\overrightarrow{\mathbf{F}}}_{23}$ exerted by $q_2$ on $q_3$. $F_{23x}=$ $\mathrm{N}$ $F_{23y}=$ $\mathrm{N}$ (b) Find the components of the force ${\overrightarrow{\mathbf{F}}}_{13}$ exerted by $q_1$ on $q_3$. $F_{13x}=$ $\mathrm{N}$ $F_{13y}=$ $\mathrm{N}$ (c) Find the resultant force on $q_3$, in terms of components and also in terms of magnitude and direction. $\begin{array}{ll}{F}_x=& \mathrm{N}\\ F_y=& \mathrm{N}\\ \text{ magnitude }& \mathrm{N}\\ \text{ direction }& \end{array}$ EXERCISE HINTS: GETTING STARTED | I'M STUCK! Using the same triangle, find the vector components of the electric force on $q_1$ and the vector's magnitude and direction. (Use the charges given in the Practice It section.) $F_x=\frac{?7.608\mathrm{e}?9}{\text{ Go back to your diagram of the system. Use the directions of the force arrows help you to }}$ estimate the direction and relative size of the total force. Then check the vector addition. $\mathrm{N}$ $F_y=$ Go back to your diagram of the system. Use the directions of the force arrows help you to estimate the direction and relative size of the total force. Then check the vector addition. $\mathrm{N}$ magnitude You used an appropriate expression for $F$, but since at least one of your earlier answers is incorrect, this answer is wrong too. $\mathrm{N}$ direction Your expression for the angle is correct, but your value for $F_y/F_x$ is incorrect, so this answer is wrong too. ${}^{?}$ counterclockwise from the $+x$-axis