(Solved): Given three noncollinear points, there is one and only one circle that passes through them. Knowin ...

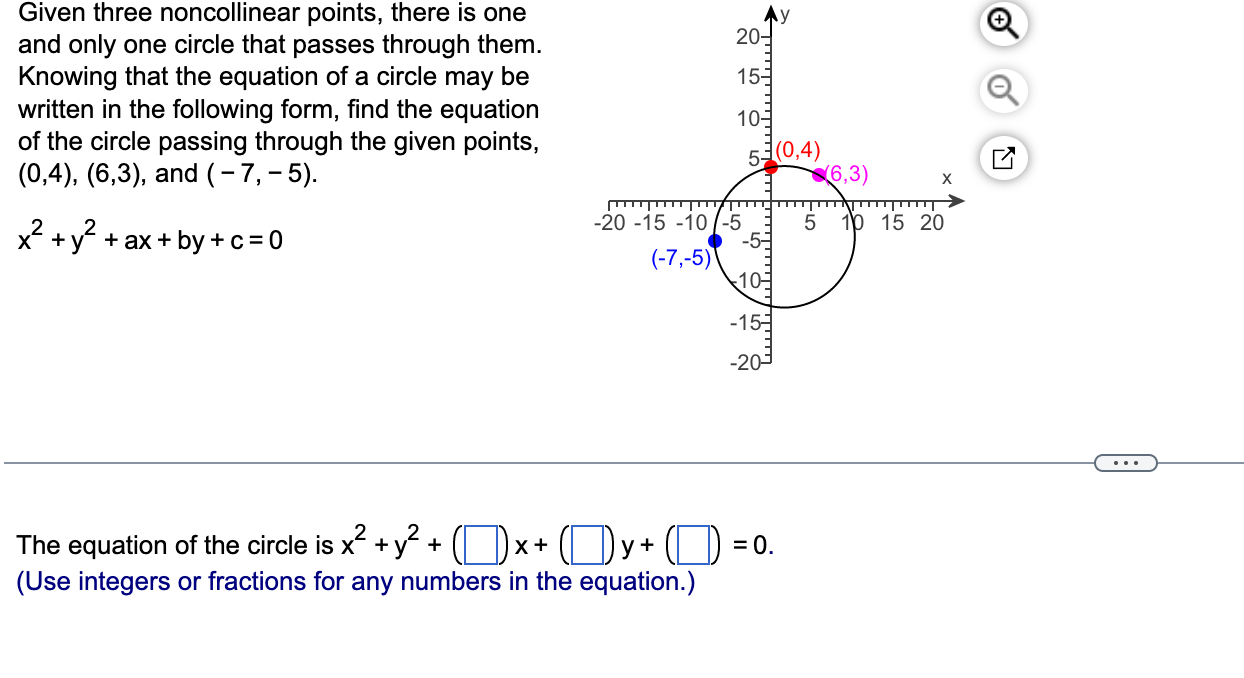
Given three noncollinear points, there is one and only one circle that passes through them. Knowing that the equation of a circle may be written in the following form, find the equation of the circle passing through the given points, \( (0,4),(6,3) \), and \( (-7,-5) \) \[ x^{2}+y^{2}+a x+b y+c=0 \] The equation of the circle is \( x^{2}+y^{2}+(\quad) x+(\quad) y+(\quad)=0 \). (Use integers or fractions for any numbers in the equation.)