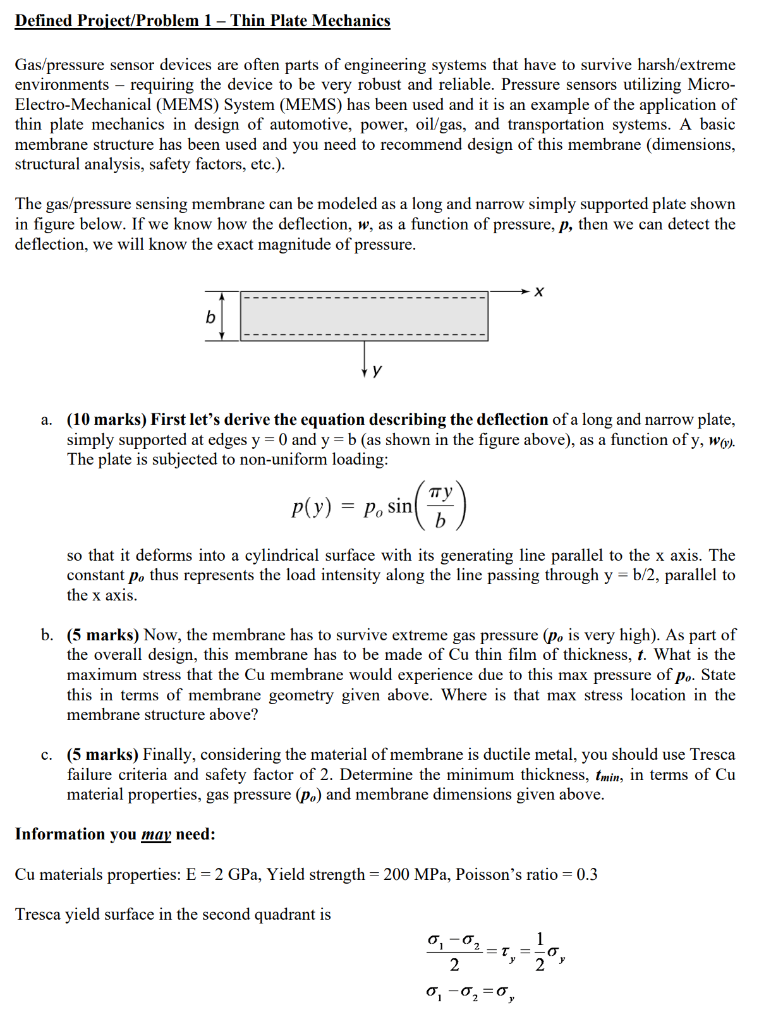
(Solved): Gas/pressure sensor devices are often parts of engineering systems that have to survive harsh/extrem ...

Gas/pressure sensor devices are often parts of engineering systems that have to survive harsh/extreme environments – requiring the device to be very robust and reliable. Pressure sensors utilizing MicroElectro-Mechanical (MEMS) System (MEMS) has been used and it is an example of the application of thin plate mechanics in design of automotive, power, oil/gas, and transportation systems. A basic membrane structure has been used and you need to recommend design of this membrane (dimensions, structural analysis, safety factors, etc.). The gas/pressure sensing membrane can be modeled as a long and narrow simply supported plate shown in figure below. If we know how the deflection, w, as a function of pressure, p, then we can detect the deflection, we will know the exact magnitude of pressure. a. (10 marks) First let’s derive the equation describing the deflection of a long and narrow plate, simply supported at edges y = 0 and y = b (as shown in the figure above), as a function of y, w(y). The plate is subjected to non-uniform loading: so that it deforms into a cylindrical surface with its generating line parallel to the x axis. The constant po thus represents the load intensity along the line passing through y = b/2, parallel to the x axis. b. (5 marks) Now, the membrane has to survive extreme gas pressure (po is very high). As part of the overall design, this membrane has to be made of Cu thin film of thickness, t. What is the maximum stress that the Cu membrane would experience due to this max pressure of po. State this in terms of membrane geometry given above. Where is that max stress location in the membrane structure above? c. (5 marks) Finally, considering the material of membrane is ductile metal, you should use Tresca failure criteria and safety factor of 2. Determine the minimum thickness, tmin, in terms of Cu material properties, gas pressure (po) and membrane dimensions given above.

Defined Project/Problem 1 - Thin Plate Mechanics Gas/pressure sensor devices are often parts of engineering systems that have to survive harsh/extreme environments - requiring the device to be very robust and reliable. Pressure sensors utilizing MicroElectro-Mechanical (MEMS) System (MEMS) has been used and it is an example of the application of thin plate mechanics in design of automotive, power, oil/gas, and transportation systems. A basic membrane structure has been used and you need to recommend design of this membrane (dimensions, structural analysis, safety factors, etc.). The gas/pressure sensing membrane can be modeled as a long and narrow simply supported plate shown in figure below. If we know how the deflection, $$w$$, as a function of pressure, $$\mathbf{p}$$, then we can detect the deflection, we will know the exact magnitude of pressure. a. (10 marks) First let's derive the equation describing the deflection of a long and narrow plate, simply supported at edges $$\mathrm{y}=0$$ and $$\mathrm{y}=\mathrm{b}$$ (as shown in the figure above), as a function of $$\mathrm{y},{\mathbf{w}}_{(y)}$$. The plate is subjected to non-uniform loading: $$p(y)=p_o\sin \left(\frac{?y}{b}\right)$$ so that it deforms into a cylindrical surface with its generating line parallel to the $$\mathrm{x}$$ axis. The constant $$p_o$$ thus represents the load intensity along the line passing through $$\mathrm{y}=\mathrm{b}/2$$, parallel to the $$x$$ axis. b. (5 marks) Now, the membrane has to survive extreme gas pressure ( $$p_o$$ is very high). As part of the overall design, this membrane has to be made of $$\mathrm{Cu}$$ thin film of thickness, $$\mathbf{t}$$. What is the maximum stress that the $$\mathrm{Cu}$$ membrane would experience due to this max pressure of $${\mathbf{p}}_0$$. State this in terms of membrane geometry given above. Where is that max stress location in the membrane structure above? c. (5 marks) Finally, considering the material of membrane is ductile metal, you should use Tresca failure criteria and safety factor of 2. Determine the minimum thickness, $${\mathbf{t}}_{\text{min }}$$, in terms of $$\mathrm{Cu}$$ material properties, gas pressure $$\left({\mathbf{p}}_{\mathbf{o}}\right)$$ and membrane dimensions given above. Information you mav need: Cu materials properties: $$\mathrm{E}=2\mathrm{GPa}$$, Yield strength $$=200\mathrm{MPa}$$, Poisson's ratio $$=0.3$$ Tresca yield surface in the second quadrant is $$\begin{array}{l}\frac{{?}_1?{?}_2}{2}={?}_y=\frac{1}{2}{?}_y\\ {?}_1?{?}_2={?}_y\end{array}$$