Home /
Expert Answers /
Statistics and Probability /
formulas-for-binomial-distribution-39-s-pmf-cdf-expected-value-and-variance-in-a-binomial-experime-pa285
(Solved): Formulas for Binomial Distribution's PMF, CDF, Expected Value, and Variance: In a Binomial Experime ...
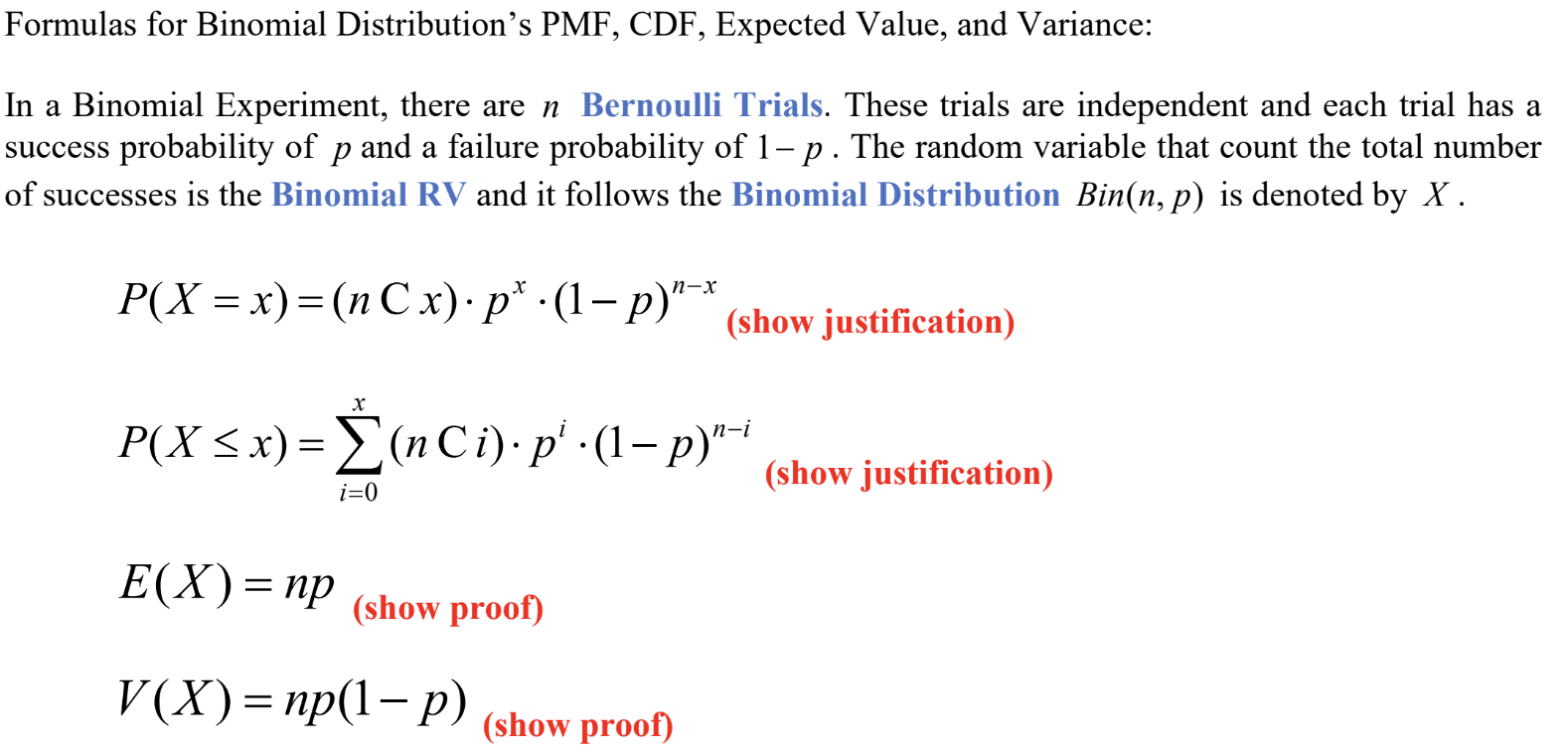
Formulas for Binomial Distribution's PMF, CDF, Expected Value, and Variance: In a Binomial Experiment, there are Bernoulli Trials. These trials are independent and each trial has a success probability of and a failure probability of . The random variable that count the total number of successes is the Binomial and it follows the Binomial Distribution is denoted by .
Expert Answer
The probability mass function (PMF) of the binomial distribution is given by:P(X = x) = (nCx) * p^x * (1 - p)^(n - x)Let's justify this formula step by step:1. (nCx) represents the number of ways to choose x successes out of n trials, which is calcul...