(Solved): Flux: Surface Integral of a Vector Field Let \( \mathbf{r}(u, v) \) be a surface described paramet ...

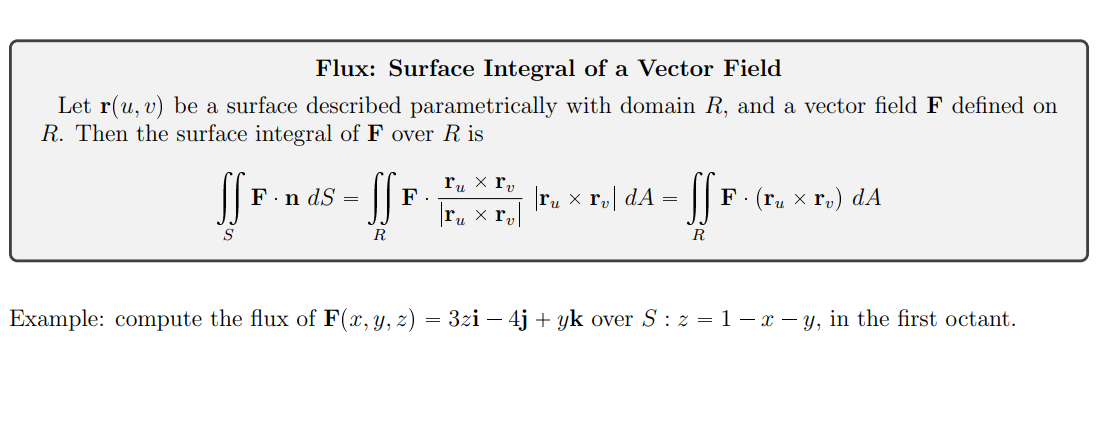
Flux: Surface Integral of a Vector Field Let \( \mathbf{r}(u, v) \) be a surface described parametrically with domain \( R \), and a vector field \( \mathbf{F} \) defined on \( R \). Then the surface integral of \( \mathbf{F} \) over \( R \) is \[ \iint_{S} \mathbf{F} \cdot \mathbf{n} d S=\iint_{R} \mathbf{F} \cdot \frac{\mathbf{r}_{u} \times \mathbf{r}_{v}}{\left|\mathbf{r}_{u} \times \mathbf{r}_{v}\right|}\left|\mathbf{r}_{u} \times \mathbf{r}_{v}\right| d A=\iint_{R} \mathbf{F} \cdot\left(\mathbf{r}_{u} \times \mathbf{r}_{v}\right) d A \] Example: compute the flux of \( \mathbf{F}(x, y, z)=3 z \mathbf{i}-4 \mathbf{j}+y \mathbf{k} \) over \( S: z=1-x-y \), in the first octant.