(Solved): Find the unit tangent vector, the principal normal vector, and an equation in \( x, y, z \) for th ...

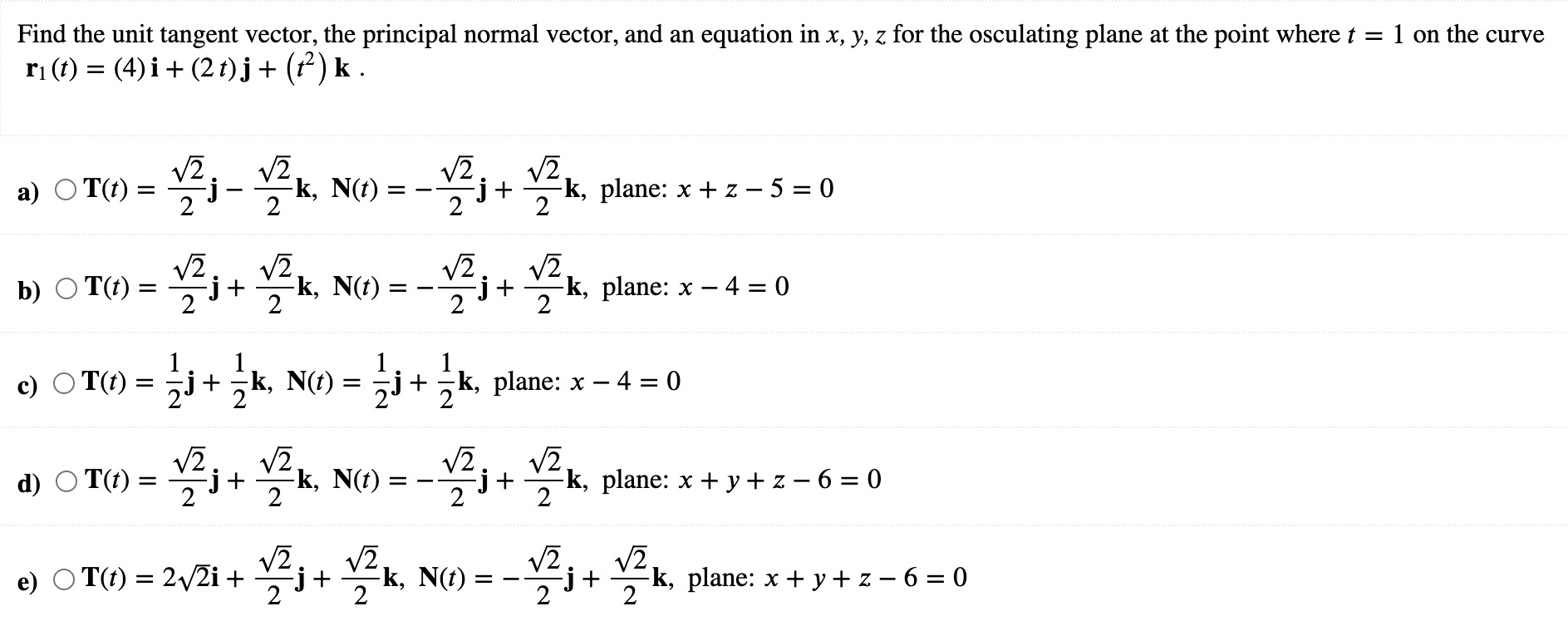
Find the unit tangent vector, the principal normal vector, and an equation in \( x, y, z \) for the osculating plane at the point where \( t=1 \) on the curve \( \mathbf{r}_{1}(t)=(4) \mathbf{i}+(2 t) \mathbf{j}+\left(t^{2}\right) \mathbf{k} \). a) \( \mathbf{T}(t)=\frac{\sqrt{2}}{2} \mathbf{j}-\frac{\sqrt{2}}{2} \mathbf{k}, \mathbf{N}(t)=-\frac{\sqrt{2}}{2} \mathbf{j}+\frac{\sqrt{2}}{2} \mathbf{k} \), plane: \( x+z-5=0 \) b) \( \mathbf{T}(t)=\frac{\sqrt{2}}{2} \mathbf{j}+\frac{\sqrt{2}}{2} \mathbf{k}, \mathbf{N}(t)=-\frac{\sqrt{2}}{2} \mathbf{j}+\frac{\sqrt{2}}{2} \mathbf{k} \), plane: \( x-4=0 \) c) \( \mathbf{T}(t)=\frac{1}{2} \mathbf{j}+\frac{1}{2} \mathbf{k}, \mathbf{N}(t)=\frac{1}{2} \mathbf{j}+\frac{1}{2} \mathbf{k} \), plane: \( x-4=0 \) d) \( \mathbf{T}(t)=\frac{\sqrt{2}}{2} \mathbf{j}+\frac{\sqrt{2}}{2} \mathbf{k}, \mathbf{N}(t)=-\frac{\sqrt{2}}{2} \mathbf{j}+\frac{\sqrt{2}}{2} \mathbf{k} \), plane: \( x+y+z-6=0 \) e) \( \quad \mathbf{T}(t)=2 \sqrt{2} \mathbf{i}+\frac{\sqrt{2}}{2} \mathbf{j}+\frac{\sqrt{2}}{2} \mathbf{k}, \mathbf{N}(t)=-\frac{\sqrt{2}}{2} \mathbf{j}+\frac{\sqrt{2}}{2} \mathbf{k} \), plane: \( x+y+z-6=0 \)