(Solved): Find the unit tangent vector of the given curve. \[ \begin{aligned} \mathbf{r}(\mathrm{t})=(9 & \le ...

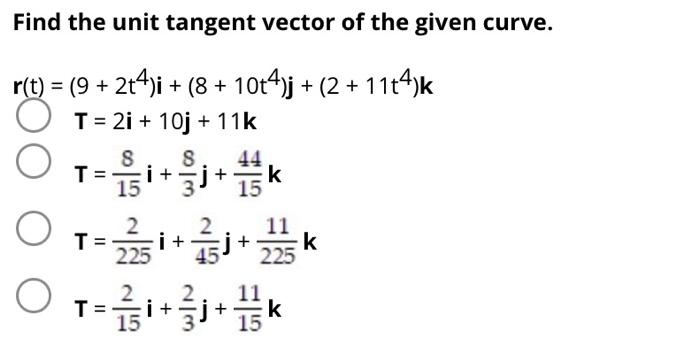
Find the unit tangent vector of the given curve. \[ \begin{aligned} \mathbf{r}(\mathrm{t})=(9 & \left.+2 \mathrm{t}^{4}\right) \mathbf{i}+\left(8+10 \mathrm{t}^{4}\right) \mathbf{j}+\left(2+11 \mathrm{t}^{4}\right) \mathbf{k} \\ \mathbf{T} & =2 \mathbf{i}+10 \mathbf{j}+11 \mathbf{k} \\ \mathbf{T} & =\frac{8}{15} \mathbf{i}+\frac{8}{3} \mathbf{j}+\frac{44}{15} \mathbf{k} \\ \mathbf{T} & =\frac{2}{225} \mathbf{i}+\frac{2}{45} \mathbf{j}+\frac{11}{225} \mathbf{k} \\ \mathbf{T} & =\frac{2}{15} \mathbf{i}+\frac{2}{3} \mathbf{j}+\frac{11}{15} \mathbf{k} \end{aligned} \]