(Solved): Find the unit tangent vector \( \mathbf{T} \) and the principal unit normal vector \( \mathbf{N} \ ...

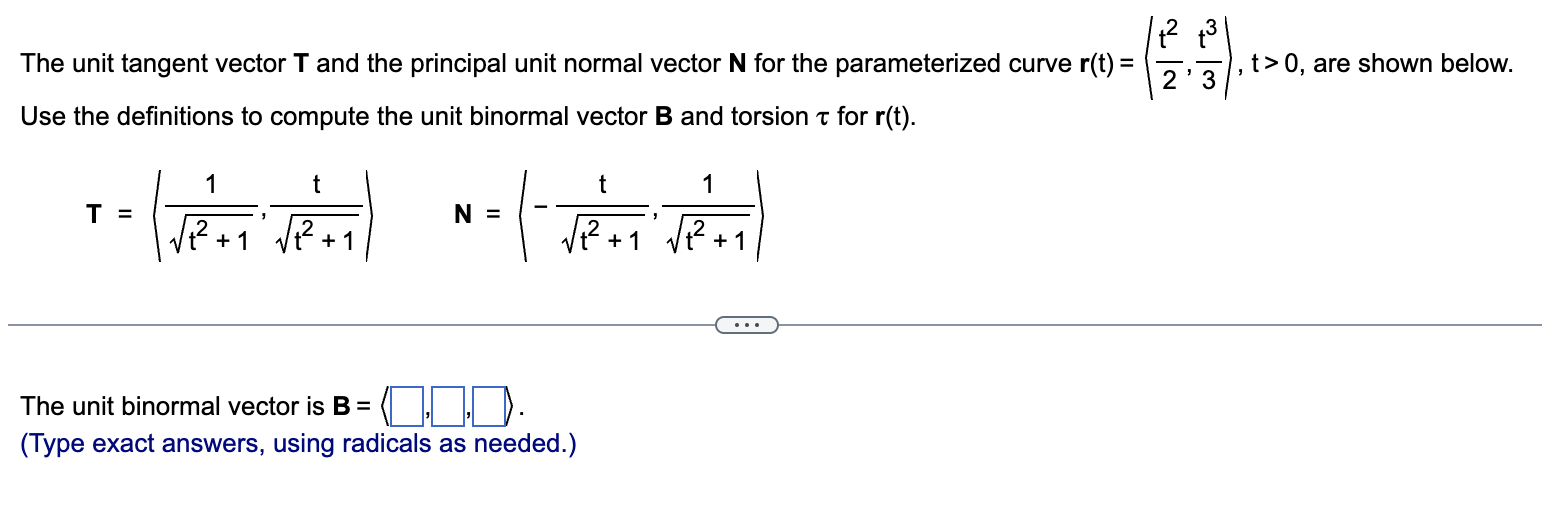
Find the unit tangent vector \( \mathbf{T} \) and the principal unit normal vector \( \mathbf{N} \) for the following parameterized curve. Verify that \( |\mathbf{T}|=|\mathbf{N}|=1 \) and \( \mathbf{T} \cdot \mathbf{N}=0 \). \[ r(t)=\langle-2 t,-2 \ln (\cos t)\rangle \text { for }-\frac{\pi}{2}0 \), are shown below. Use the definitions to compute the unit binormal vector \( \mathbf{B} \) and torsion \( \tau \) for \( \mathbf{r}(\mathrm{t}) \). \[ \mathbf{T}=\left\langle\frac{1}{\sqrt{t^{2}+1}}, \frac{t}{\sqrt{t^{2}+1}}\right| \quad \mathbf{N}=\left\langle-\frac{t}{\sqrt{t^{2}+1}}, \frac{1}{\sqrt{t^{2}+1}}\right| \] The unit binormal vector is \( \mathbf{B}=\langle\quad, \quad 1 \). 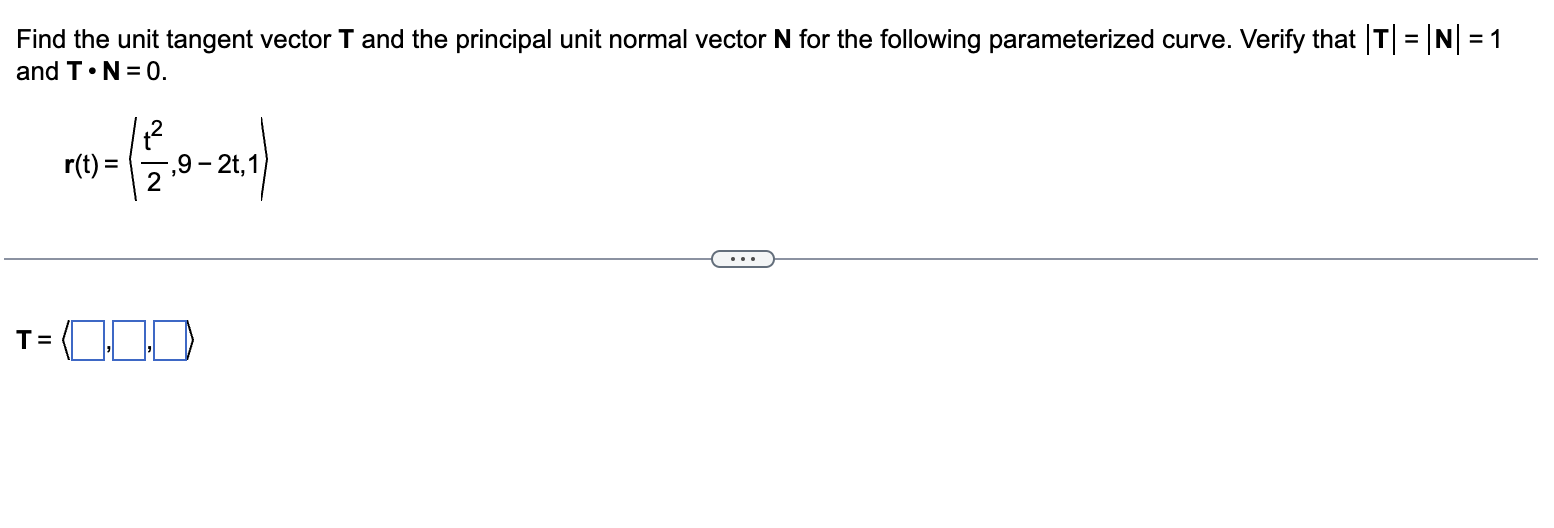(Type exact answers, using radicals as needed.) Find the unit tangent vector \( \mathbf{T} \) and the principal unit normal vector \( \mathbf{N} \) for the following parameterized curve. Verify that \( |\mathbf{T}|=|\mathbf{N}|=1 \) and \( \mathbf{T} \cdot \mathbf{N}=0 \). 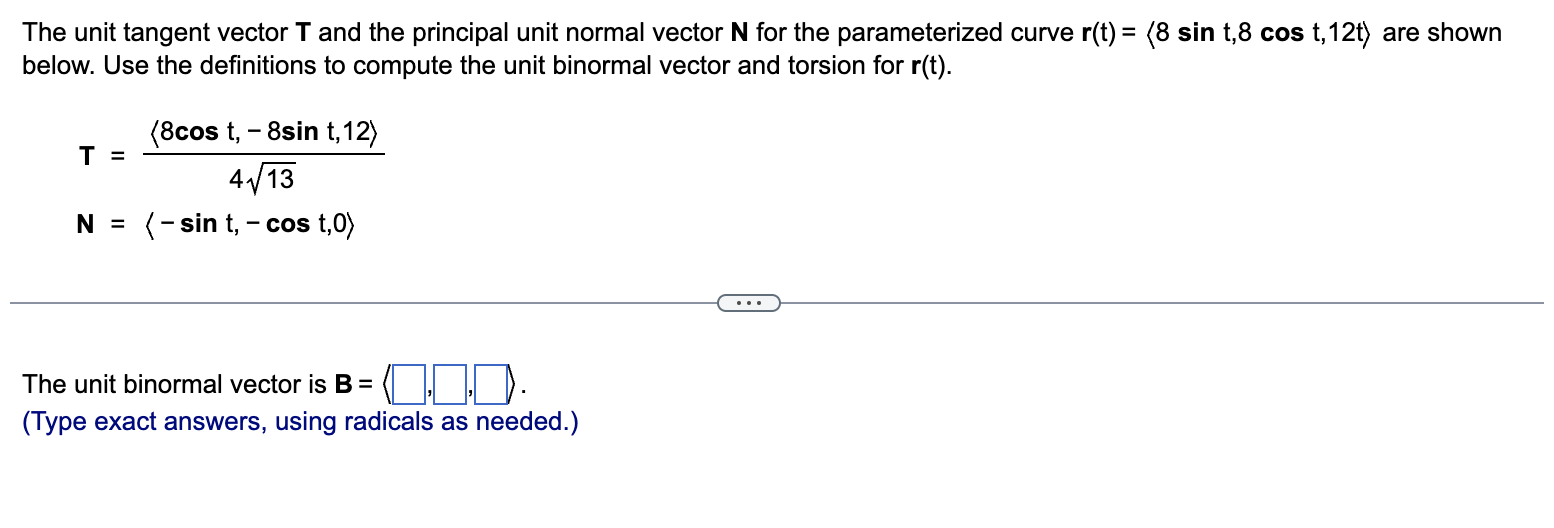\[ \mathbf{r}(t)=\left\langle\frac{t^{2}}{2}, 9-2 t, 1\right| \] The unit tangent vector \( \mathbf{T} \) and the principal unit normal vector \( \mathbf{N} \) for the parameterized curve \( \mathbf{r}(\mathrm{t})=\langle 8 \sin t, 8 \cos t, 12 \mathrm{t}\rangle \) are shown below. Use the definitions to compute the unit binormal vector and torsion for \( r(t) \). \[ \begin{array}{l} \mathbf{T}=\frac{\langle 8 \cos t,-8 \sin t, 12\rangle}{4 \sqrt{13}} \\ \mathbf{N}=\langle-\sin t,-\cos t, 0\rangle \end{array} \] The unit binormal vector is \( \mathbf{B}=\langle, \),\( rangle . \) (Type exact answers, using radicals as needed.)