(Solved): Find the matrix of the linear transformation \( \mathrm{T} \) with respect to the bases given: a) ...

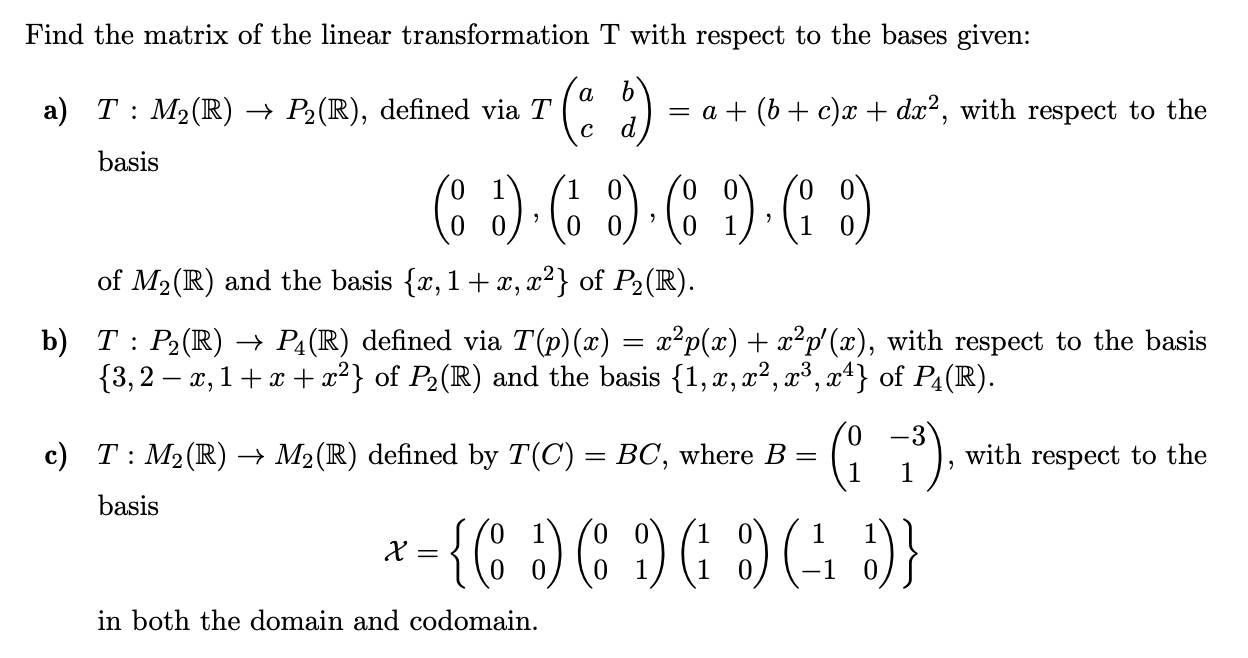
Find the matrix of the linear transformation \( \mathrm{T} \) with respect to the bases given: a) \( T: M_{2}(\mathbb{R}) \rightarrow P_{2}(\mathbb{R}) \), defined via \( T\left(\begin{array}{ll}a & b \\ c & d\end{array}\right)=a+(b+c) x+d x^{2} \), with respect to the basis \[ \left(\begin{array}{ll} 0 & 1 \\ 0 & 0 \end{array}\right),\left(\begin{array}{ll} 1 & 0 \\ 0 & 0 \end{array}\right),\left(\begin{array}{ll} 0 & 0 \\ 0 & 1 \end{array}\right),\left(\begin{array}{ll} 0 & 0 \\ 1 & 0 \end{array}\right) \] of \( M_{2}(\mathbb{R}) \) and the basis \( \left\{x, 1+x, x^{2}\right\} \) of \( P_{2}(\mathbb{R}) \). b) \( T: P_{2}(\mathbb{R}) \rightarrow P_{4}(\mathbb{R}) \) defined via \( T(p)(x)=x^{2} p(x)+x^{2} p^{\prime}(x) \), with respect to the basis \( \left\{3,2-x, 1+x+x^{2}\right\} \) of \( P_{2}(\mathbb{R}) \) and the basis \( \left\{1, x, x^{2}, x^{3}, x^{4}\right\} \) of \( P_{4}(\mathbb{R}) \). c) \( T: M_{2}(\mathbb{R}) \rightarrow M_{2}(\mathbb{R}) \) defined by \( T(C)=B C \), where \( B=\left(\begin{array}{cc}0 & -3 \\ 1 & 1\end{array}\right) \), with respect to the basis \[ \mathcal{X}=\left\{\left(\begin{array}{ll} 0 & 1 \\ 0 & 0 \end{array}\right)\left(\begin{array}{ll} 0 & 0 \\ 0 & 1 \end{array}\right)\left(\begin{array}{ll} 1 & 0 \\ 1 & 0 \end{array}\right)\left(\begin{array}{cc} 1 & 1 \\ -1 & 0 \end{array}\right)\right\} \] in both the domain and codomain.