(Solved): Find the characteristic equation and eigenvalues of the matrix and a basis for each of the corresp ...

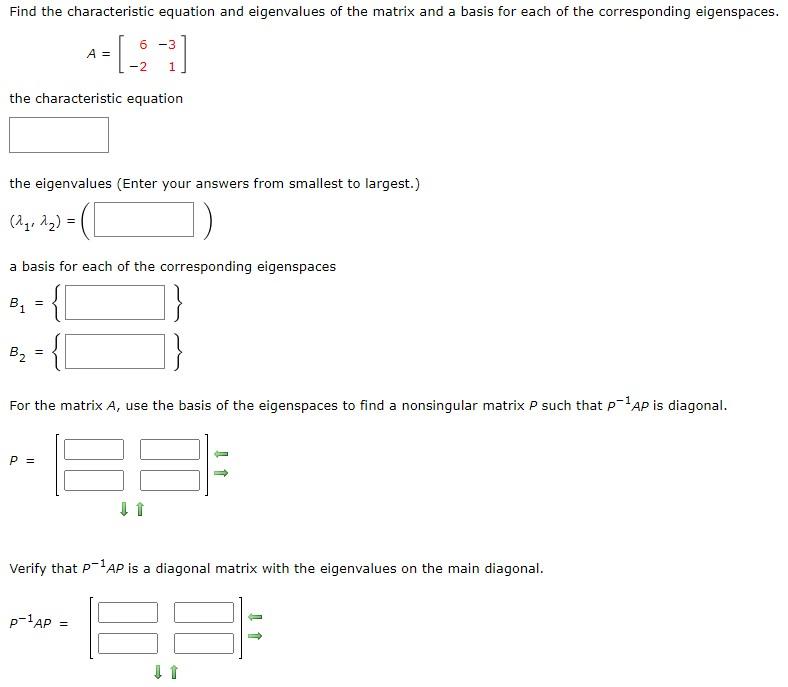
Find the characteristic equation and eigenvalues of the matrix and a basis for each of the corresponding eigenspaces. \[ A=\left[\begin{array}{rr} 6 & -3 \\ -2 & 1 \end{array}\right] \] the characteristic equation the eigenvalues (Enter your answers from smallest to largest.) \[ \left(\lambda_{1}, \lambda_{2}\right)=(\quad) \] a basis for each of the corresponding eigenspaces \[ \begin{array}{ll} B_{1}=\{ & \} \\ B_{2}=\{ & \} \end{array} \] For the matrix \( A \), use the basis of the eigenspaces to find a nonsingular matrix \( P \) such that \( P^{-1} A P \) is diagonal. \[ P=\left[\begin{array}{c} \Downarrow \mathbb{1} \end{array}\right] \Rightarrow \] Verify that \( P^{-1} A P \) is a diagonal matrix with the eigenvalues on the main diagonal.