(Solved): Find the Banzhaf power distribution of the weighted voting system \( [31: 20,17,13,11] \) Give each ...

Find the Banzhaf power distribution of the weighted voting system \( [31: 20,17,13,11] \) Give each player's power as a fraction or decimal value \[ \begin{array}{l} P_{1}= \\ P_{2}= \\ P_{3}= \\ P_{4}= \end{array} \] Consider the weighted voting system \( [11: 7,4,1] \) Find the Shapley-Shubik power distribution of this weighted voting system. 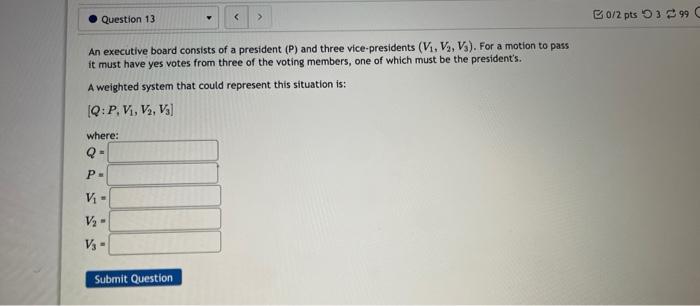List the power for each plaver as a fraction: \( P_{1} \) \( P_{2} \) \( P_{3} \) An executive board consists of a president \( (P) \) and three vice-presidents \( \left(V_{1}, V_{2}, V_{3}\right) \). For a motion to pass it must have yes votes from three of the voting members, one of which must be the president's. A weighted system that could represent this situation is: \[ \left[Q: P, V_{1}, V_{2}, V_{3}\right] \] where: \[ \begin{array}{l} Q= \\ P= \\ V_{1}= \\ V_{2}= \\ V_{3}= \end{array} \]