(Solved): Exercise 1: Analysis of thick cylinder under internal/external pressure A thick-walled cylinder un ...

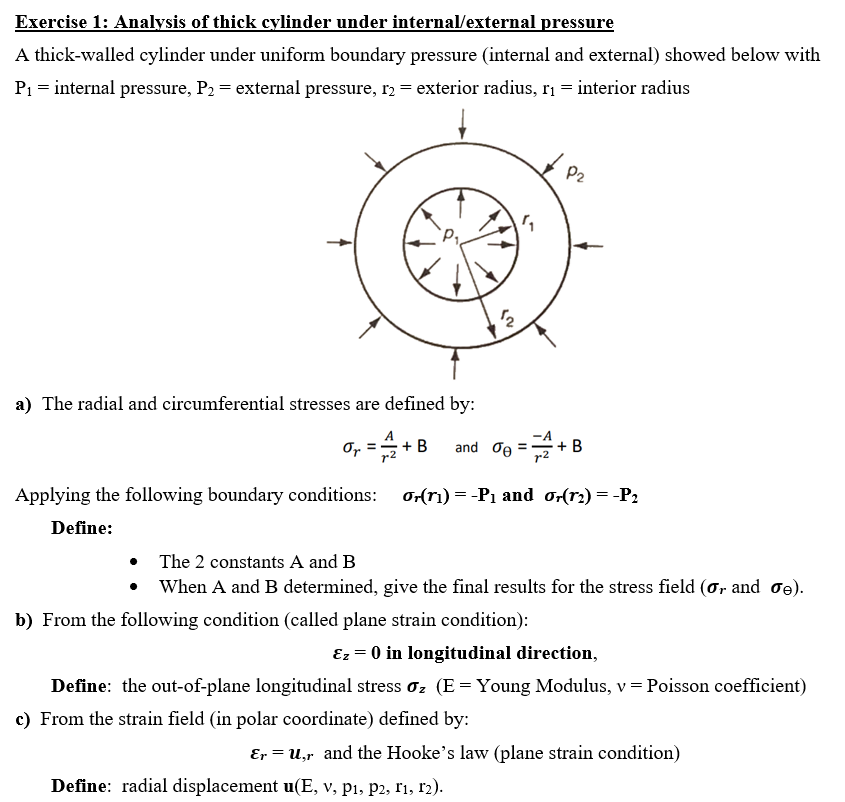
Exercise 1: Analysis of thick cylinder under internal/external pressure A thick-walled cylinder under uniform boundary pressure (internal and external) showed below with \( P_{1}= \) internal pressure, \( P_{2}= \) external pressure, \( r_{2}= \) exterior radius, \( r_{1}= \) interior radius a) The radial and circumferential stresses are defined by: \[ \sigma_{r}=\frac{A}{r^{2}}+\mathrm{B} \text { and } \sigma_{\theta}=\frac{-A}{r^{2}}+\mathrm{B} \] Applying the following boundary conditions: \( \quad \sigma_{r}\left(r_{1}\right)=-\mathbf{P}_{1} \) and \( \sigma_{r}\left(r_{2}\right)=-\mathbf{P}_{2} \) Define: - The 2 constants A and B - When \( \mathrm{A} \) and \( \mathrm{B} \) determined, give the final results for the stress field \( \left(\sigma_{r}\right. \) and \( \left.\sigma_{\ominus}\right) \). b) From the following condition (called plane strain condition): \( \varepsilon_{z}=0 \) in longitudinal direction, Define: the out-of-plane longitudinal stress \( \sigma_{z} \) ( \( \mathrm{E}= \) Young Modulus, \( v= \) Poisson coefficient) c) From the strain field (in polar coordinate) defined by: \( \boldsymbol{\varepsilon}_{r}=\boldsymbol{u}, \boldsymbol{r} \) and the Hooke's law (plane strain condition) Define: radial displacement \( \mathbf{u}\left(\mathrm{E}, v, \mathrm{p}_{1}, \mathrm{p}_{2}, \mathrm{r}_{1}, \mathrm{r}_{2}\right) \)