(Solved): Ex. 7 (a) 10 Write xy, xz, and (x2 - y) as components of a spherical (irreducible) tensor of ran ...

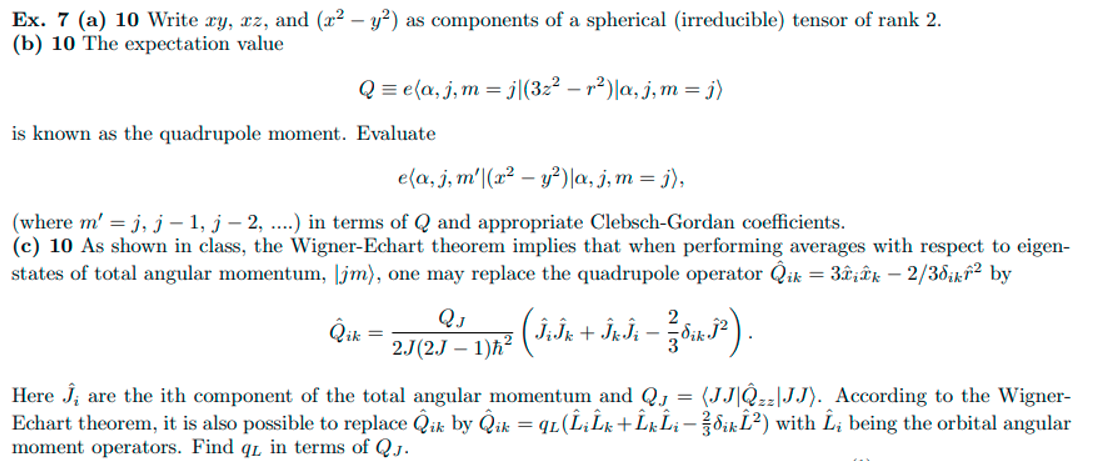
Ex. 7 (a) 10 Write xy, xz, and (x2 - y²) as components of a spherical (irreducible) tensor of rank 2. (b) 10 The expectation value Q = e(a, j,m=j|(3z2 – m2)]a, j, m=j) is known as the quadrupole moment. Evaluate e(a, j, m'|(x2 - y2)|a, j, m = j), (where m' = j, j - 1, j - 2, ....) in terms of Q and appropriate Clebsch-Gordan coefficients. (c) 10 As shown in class, the Wigner-Echart theorem implies that when performing averages with respect to eigen- states of total angular momentum, \jm), one may replace the quadrupole operator Qik = 3 ?zîk – 2/381112 by = 2 Qik Qj 2.J(2.J - - 1) JiJk+ ?z): – bu ja). Here ?i are the ith component of the total angular momentum and QJ = (JJ\@2z|J.J). According to the Wigner- Echart theorem, it is also possible to replace Qik by Qik = qu(οÎx+ .x; – dikî?) with Î; being the orbital angular moment operators. Find qu in terms of Q.J.