(Solved): Electromagnetic  1) A uniform current density given by \( J=2 J_{0} \mathrm{~A} / \mathrm{m}^{2 ...

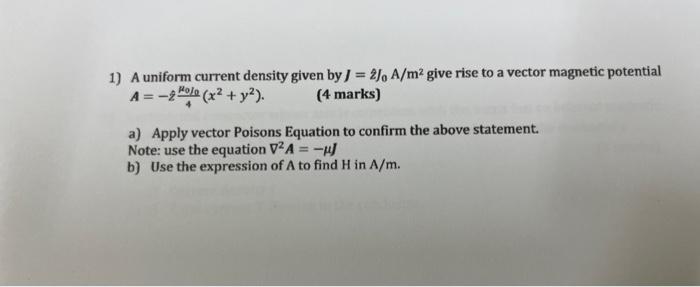
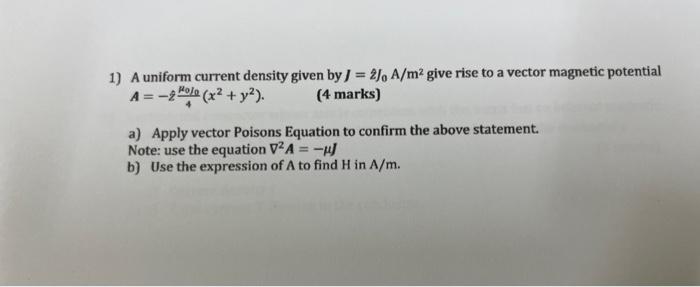
1) A uniform current density given by \( J=2 J_{0} \mathrm{~A} / \mathrm{m}^{2} \) give rise to a vector magnetic potential \( A=-2 \frac{\mu_{0} / 0}{4}\left(x^{2}+y^{2}\right) \) (4 marks) a) Apply vector Poisons Equation to confirm the above statement. Note: use the equation \( \nabla^{2} A=-\mu J \) b) Use the expression of \( A \) to find \( \mathrm{H} \) in \( \mathrm{A} / \mathrm{m} \). 2) A cylindrical conductor whose axis is coincident with the z-axis has an internal magnetic field given by \( H=\emptyset^{2} \frac{2}{r}\left[1-(4 r+1) e^{-4 r}\right] \mathrm{A} / \mathrm{m} \), for \( \mathrm{r} \leq \mathrm{a} \), where ' \( \mathrm{a} \) ' is the conductors radius. If \( a=5 \mathrm{~cm} \), find, (4 marks) a) Current density J b) Total current ' \( \mathrm{I} \) ' flowing in the conductor. 3) A coil consists of 200 turns of wire wrapped around a square frame of sides \( 0.25 \mathrm{~cm} \). The coil is centered at the origin with each of its sides parallel to the \( x \) or \( y \) axis. Find the induced emf across the open-circuited ends of the coil if the magnetic field is given by ( 8 marks) (a) \( \mathbf{B}=\mathbf{z} 20 e^{-3 t} \) (T) (b) \( \mathbf{B}=\hat{\mathbf{z}} 20 \cos x \cos 10^{3} t \) (T) (c) \( \mathbf{B}=\hat{\mathbf{z}} 20 \cos x \sin 2 y \cos 10^{3} t \) (T) 4) The current density in a conducting medium is given below. Determine the corresponding charge distribution \( \rho_{n} \). (4 marks) \[ \mathbf{J}(x, y, z ; t)=\left(\mathbf{x} z-\hat{y} 4 y^{2}+\mathbf{z} 2 x\right) \cos \omega t \] Hint: use the below relation and integrate the answer to get po \[ \nabla \cdot \mathbf{J}=-\frac{\partial \rho_{\mathrm{v}}}{\partial t} \]