(Solved): double integration method Problem 1: A beam with a square cross-sectional area is loaded and support ...

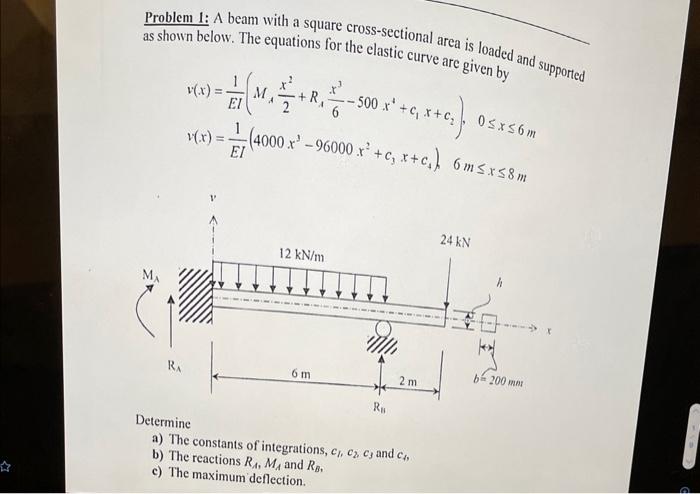
Problem 1: A beam with a square cross-sectional area is loaded and supported as shown below. The equations for the elastic curve are given by \[ \begin{array}{l} v(x)=\frac{1}{E I}\left(M_{1} \frac{x^{2}}{2}+R_{4} \frac{x^{3}}{6}-500 x^{4}+c_{1} x+c_{2}\right), 0 \leq x \leq 6 m \\ v(x)=\frac{1}{E I}\left(4000 x^{3}-96000 x^{2}+c_{3} x+c_{4}\right), \quad 6 m \leq x \leq 8 m \end{array} \] a) The constants of integrations, \( c_{1}, c_{2} c_{3} \) and \( c_{1} \), b) The reactions \( R_{A}, M_{A} \) and \( R_{B} \), c) The maximum deflection.