(Solved): Differntial equations An RLC electrical circuit is exactly analogous to a mechanical mass-spring-das ...

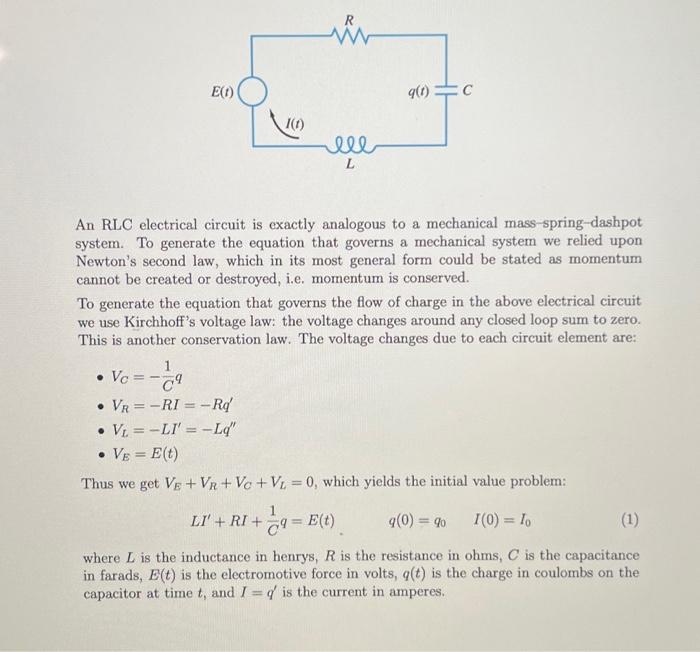
An RLC electrical circuit is exactly analogous to a mechanical mass-spring-dashpot system. To generate the equation that governs a mechanical system we relied upon Newton's second law, which in its most general form could be stated as momentum cannot be created or destroyed, i.e. momentum is conserved. To generate the equation that governs the flow of charge in the above electrical circuit we use Kirchhoff's voltage law: the voltage changes around any closed loop sum to zero. This is another conservation law. The voltage changes due to each circuit element are: - $V_C=?\frac{1}{C}q$ - $V_R=?RI=?R{q}^{?}$ - $V_L=?L{I}^{?}=?L{q}^{??}$ - $V_E=E(t)$ Thus we get $V_E+V_R+V_C+V_L=0$, which yields the initial value problem: $L{I}^{?}+RI+\frac{1}{C}q=E(t).q(0)=q_{0}I(0)=I_0$ where $L$ is the inductance in henrys, $R$ is the resistance in ohms, $C$ is the capacitance in farads, $E(t)$ is the electromotive force in volts, $q(t)$ is the charge in coulombs on the capacitor at time $t$, and $I=q^{?}$ is the current in amperes. Find the current at time $t$ if the charge on the capacitor is initially zero, the initial current is zero, $L=6\mathrm{H},R=24?,C=(1374{)}^{?1}\mathrm{F}$, and $E(t)=50\mathrm{V}$. (a) (4 points) Use the given values to write a differential equation for $I$ as a function of $t$. (Hint: Differentiate both sides of equation (1) to obtain a homogeneous linear second order equation for $I(t)$.) (b) (4 points) Find the roots of the characteristic equation. (c) (4 points) Find the general solution to the differential equation.