(Solved): differential equation for the velocity v of a falling mass m subjected to air resistance proportion ...

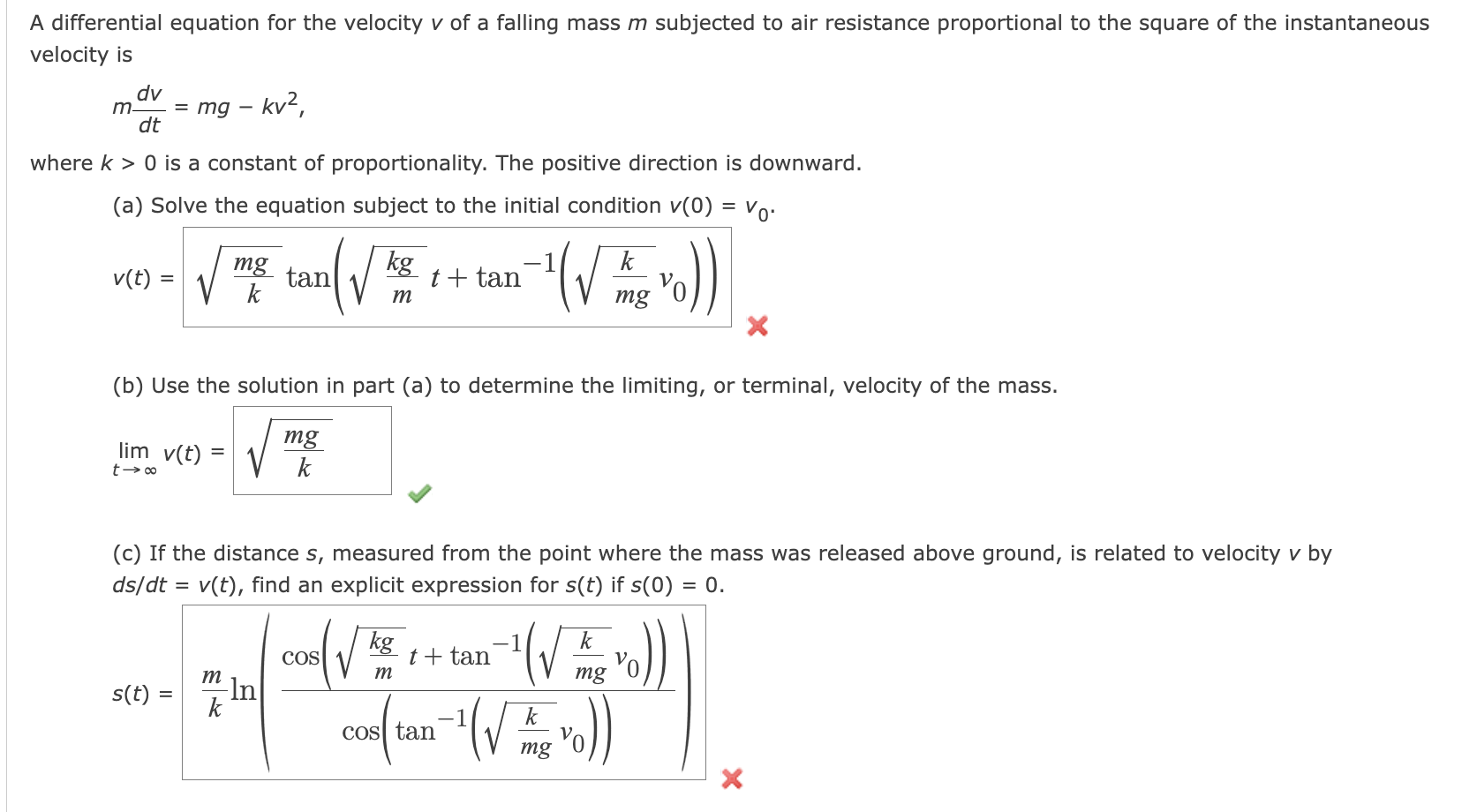
differential equation for the velocity $v$ of a falling mass $m$ subjected to air resistance proportional to the square of the instantaneous elocity is $m\frac{dv}{dt}=mg?k{v}^2$ vhere $k>0$ is a constant of proportionality. The positive direction is downward. (a) Solve the equation subject to the initial condition $v(0)=v_0$. $v(t)=\sqrt{\frac{mg}{k}}\tan \left(\sqrt{\frac{kg}{m}}t+{\tan }^{?1}\left(\sqrt{\frac{k}{mg}}{v}_0\right)\right)$ (b) Use the solution in part (a) to determine the limiting, or terminal, velocity of the mass. ${\lim }_{t??}v(t)=\sqrt{\frac{mg}{k}}$ (c) If the distance $s$, measured from the point where the mass was released above ground, is related to velocity $v$ by $ds/dt=v(t)$, find an explicit expression for $s(t)$ if $s(0)=0$. $s(t)=\frac{m}{k}\ln \left(\frac{\cos \left(\sqrt{\frac{kg}{m}}t+{\tan }^{?1}\left(\sqrt{\frac{k}{mg}}{v}_0\right)\right)}{\cos \left({\tan }^{?1}\left(\sqrt{\frac{k}{mg}}{v}_0\right)\right)}\right)$